Technical Note: Isotopic corrections for the radiocarbon composition of CO₂ in the soil gas environment must account for diffusion and diffusive mixing

Earth system scientists working with radiocarbon in organic samples use a stable carbon isotope (δ¹³C) correction to account for mass-dependent fractionation, but it has not been evaluated for the soil gas environment, wherein both diffusive gas transport and diffusive mixing are important. Using theory and an analytical soil gas transport model, we demonstrate that the conventional correction is inappropriate for interpreting the radioisotopic composition of CO₂ from biological production because it does not account for important gas transport mechanisms. Based on theory used to interpret δ¹³C of soil production from soil CO₂, we propose a new solution for radiocarbon applications in the soil gas environment that fully accounts for both mass-dependent diffusion and mass-independent diffusive mixing.

1 Introduction

Radiocarbon allows us to measure the age of soil-respired CO₂ (CO₂ diffusing from the soil surface to the atmosphere, also called soil flux as in Cerling et al., 1991), but the traditional reporting convention for radiocarbon was not established for soil gas-phase sampling; rather, it was established for solid (organic matter) sample analysis. The validity of this convention has never been explicitly tested for soil-respired CO₂.

The traditional radiocarbon reporting convention, Δ¹⁴C (Stuiver and Polach, 1977), uses a mass-dependent correction based on the isotopic composition of wood. Its purpose is to correct for biochemical fractionation against the radiocarbon isotopologue (¹⁴CO₂) abundance during photosynthesis, which is assumed to be twice as strong as for ¹³CO₂ based on their respective departures in molecular mass from ¹²CO₂. The classical reference describing these conventional calculations is Stuiver and Polach (1977).

In the soil gas environment, researchers have different implicit expectations for fractionation processes. They generally assume that ¹⁴C of CO₂ is not biochemically fractionated in the gas phase between the points of CO₂ production (biological production of CO₂ by soil organisms and roots) and measurement (subsurface or flux chamber samples). This assumption is reasonable based on the short residence time of CO₂ (minutes to days) in the soil profile before emission to the atmosphere. However, soil gas isotopic signatures depart in predictable ways from the signature of production because of physical fractionation. It has been recognized for decades that δ¹³C of CO₂ at any point in the soil profile will never equal the isotopic signature of production because of transport fractionations that alter produced CO₂ before it is measured (Cerling et al., 1991). This theory readily extends to ¹⁴C.

We argue here that in the case of soil pore space ¹⁴C in which mixing of air masses occurs, the assumption that mass-dependent fractionation is twice as large for ¹⁴C as δ¹³C by biochemical and physical processes no longer holds. Using theory and the physical modeling of soil gas transport to illustrate the issue with the current reporting convention correction, we propose an alternative approach for specific use cases.

2 Theory

To understand why the mass-dependent correction used in the Stuiver and Polach (1977) radiocarbon reporting convention may be a poor fit for soil gas studies, we can look at our current understanding of the stable isotopic composition, δ¹³C, of soil CO₂ (pore space CO₂, mole fraction with respect to dry air). We use delta notation to present the stable isotopic composition of CO₂:

$$\begin{array}{}\text{(1)}& {\mathit{\delta }}^{\mathrm{13}}\mathrm{C}=\left({\displaystyle \frac{R_{\mathrm{s}}}{R_{\mathrm{VPDB}}}}-\mathrm{1}\right)\mathrm{1000},\end{array}$$

where δ¹³C is the isotopic composition in per mill (see Table 1 for a full list of abbreviations), R_s is the ¹³C∕¹²C ratio of the sample, and R_VPDB is the ¹³C∕¹²C ratio of the international standard, Vienna Pee Dee Belemnite.

Table 1 List of symbols used. Note that the isotope composition ratios are also unitless but traditionally expressed using per mill (‰) notation.

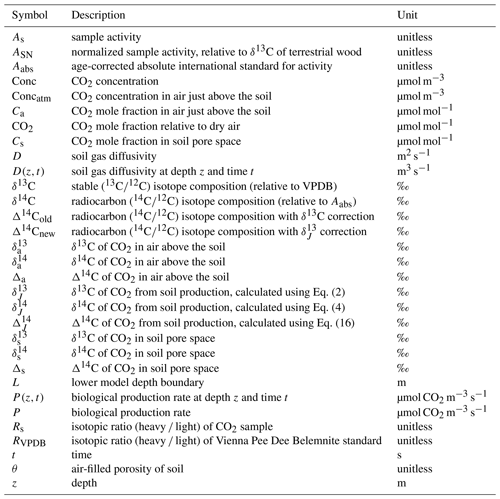
From foundational work done by Cerling et al. (1991) we know that the isotopic composition of soil CO₂ is different from that of soil-respired CO₂. Any change in δ¹³C of soil CO₂ with depth is influenced by (1) mixing of atmospheric and biological (or biogeochemical) sources of isotopically distinct CO₂, which may occur via diffusion (no bulk gas flow; referred to as diffusive mixing for the remainder of the paper) or advection (bulk gas flow), and (2) kinetic fractionation by diffusion. The effect of these is illustrated in Fig. 1 using a simulated soil gas profile. In panel (a) two depth profiles of δ¹³C of CO₂ that were modeled in a steady-state environment are shown (the model will be described in Sect. 3). The profiles differ only in soil diffusivity; all other characteristics were held constant, including rates of production, δ¹³C of CO₂ in the atmosphere (−8 ‰; circle), and biological production (−25 ‰; square with dashed line). In the resultant depth profile with higher soil diffusivity in panel (a), the δ¹³C of soil CO₂ ranges from −8 ‰ to −15.1 ‰. In the depth profile representing a soil with lower diffusivity, the δ¹³C of soil CO₂ ranges from −8 ‰ to −20.6 ‰. We stress again that these two isotopic depth profiles differ only due to differences in transport as a result of their varying soil diffusivities. In the depth profile with lower soil diffusivity, atmospheric CO₂ does not penetrate downwards as readily, so the profile shape is much steeper near the soil–atmosphere boundary and is more reflective of the production source composition, −25 ‰, at depth. In the depth profile with higher soil diffusivity, atmospheric air of −8 ‰ more readily mixes from the surface downward by diffusion, so the near-surface isotopic composition will be more reflective of the atmosphere due to diffusive mixing of these end-members near the soil surface.

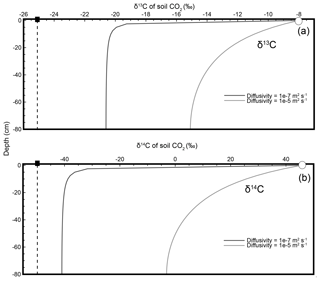
Figure 1 Modeled steady-state diffusive vertical depth profiles for δ¹³C and δ¹⁴C of soil CO₂. In panel (a) the δ¹³C of atmospheric CO₂ (circle) is −8 ‰ and CO₂ from biological production (square with dashed line; δ_J) is −25 ‰. In panel (b) the δ¹⁴C of atmospheric CO₂ (circle) is 45.5 ‰ and CO₂ from biological production (square with dashed line) is −50 ‰. Both profiles have the same biological production rates and isotopic composition of biological production, but each profile has a different soil diffusivity as indicated.

Importantly, the soil CO₂ never equals the δ¹³C of production (−25 ‰) at any depth in either profile in Fig. 1a. It is not possible to directly measure δ¹³C of production in situ because diffusion and diffusive mixing alter the character of CO₂ immediately after its production. From the site of production in the soil, ¹²CO₂ diffuses somewhat faster through the soil than ¹³CO₂ because the former has a lower mass. This diffusive difference leads to isotopic fractionation and results in depth profiles of δ¹³C of soil CO₂ that are isotopically enriched (less negative) compared to the source of production. Work by Cerling (1984) and later by Cerling et al. (1991) demonstrated that the mass differences between the two isotopologues led to a difference in the diffusion rate of each in air, amounting to a fractionation of 4.4 ‰ (note that this applies only to binary diffusion of CO₂ in air and will differ if CO₂ diffuses in other gases). As a result, the δ¹³C of soil CO₂ measured at any depth will be enriched by a minimum of 4.4 ‰ relative to the biological production CO₂ source. However, the δ¹³C of soil-respired CO₂ can be considerably more enriched than 4.4 ‰ relative to production due to diffusive mixing with the atmosphere as shown in Fig. 1a.

A convenient theoretical formulation for correcting δ¹³C for both diffusion fractionation and diffusive mixing was introduced by Davidson (1995), following on the work of Cerling (1984) and Cerling et al. (1991). This approach allows one to combine measurements of CO₂ and its isotopic composition within the soil and the air above it to infer the isotopic composition of CO₂ produced in the soil. This only applies when transport within the soil is purely by diffusion (no bulk air movement). The Davidson (Davidson, 1995) solution uses the difference between the diffusion coefficients for ¹²C and ¹³C as follows:

$$\begin{array}{}\text{(2)}& {\mathit{\delta }}_J^{\mathrm{13}}={\displaystyle \frac{C_{\mathrm{s}}\left({\mathit{\delta }}_{\mathrm{s}}^{\mathrm{13}}-\mathrm{4.4}\right)-C_{\mathrm{a}}({\mathit{\delta }}_{\mathrm{a}}^{\mathrm{13}}-\mathrm{4.4})}{\mathrm{1.0044}(C_{\mathrm{s}}-C_{\mathrm{a}})}},\end{array}$$

where ${\mathit{\delta }}_J^{\mathrm{13}}$ is the δ¹³C composition of CO₂ from soil production (biological respiration within the soil), C_s and ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{13}}$ are the mole fraction and isotopic composition of soil CO₂, and C_a and ${\mathit{\delta }}_{\mathrm{a}}^{\mathrm{13}}$ are the mole fraction and isotopic composition of CO₂ in the air just above the soil. In Fig. 2a the mole fraction and isotopic composition of soil CO₂ at a 40 cm depth and of the air just above the soil were “sampled” from model-generated soil depth profiles and the (unrounded) values were used to calculate the isotopic composition of production using Davidson's equation (C_s= 14 780 ppm, ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{13}}=-\mathrm{20.3832}$ ‰, C_a=380 ppm, and ${\mathit{\delta }}_{\mathrm{a}}^{\mathrm{13}}=-\mathrm{8}$ ‰). The resulting ${\mathit{\delta }}_J^{\mathrm{13}}$ (e.g., Eq. 2) at this depth equals the true isotopic composition of production (see inset box, Fig. 2a). However, because the Davidson approach accounts for diffusion and diffusive mixing, at any given soil depth, not just 40 cm, the modeled values of C_s and ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{13}}$ in Figs. 1a and 2a will always yield (via Eq. 2) the true isotopic composition of production, ${\mathit{\delta }}_J^{\mathrm{13}}=-\mathrm{25}$ ‰ (dashed line). If δ¹³C of soil CO₂ were (erroneously) interpreted to represent the δ¹³C of soil-respired CO₂, the error could be as large as the absolute value of (${\mathit{\delta }}_{\mathrm{a}}-{\mathit{\delta }}_J^{\mathrm{13}})-\mathrm{4.4}$ ‰. This Davidson (1995) ${\mathit{\delta }}_J^{\mathrm{13}}$ approach has been shown to be robust when applied to field data from natural soils (Breecker et al., 2012; Bowling et al., 2015; Liang et al., 2016).

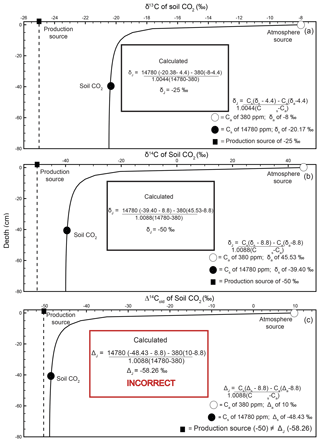
Figure 2 Modeled steady-state diffusive vertical depth profiles for δ¹³C (a), δ¹⁴C (b), and Δ¹⁴C_old (c) of soil CO₂. The three soil profiles were generated using the same soil production and diffusivity rates (2 µmol m⁻³ s⁻¹ and $\mathrm{1}\times {\mathrm{10}}^{-\mathrm{6}}$ m² s⁻¹, respectively). Panels (a) and (b) were prepared using δ¹³C and δ¹⁴C as noted. Panel (c) shows an approach consistent with present day, whereby the Δ¹⁴C profile generated by the model incorporates the traditional Stuiver and Polach (1974) correction for biochemical fractionation. Inset “Calculated” panels show how, using input data read directly from each depth profile, a user would arrive at either the correct or incorrect isotopic value of production using a Davidson approach to adjust for in-soil gas transport. The atmospheric source (C_a) composition is presented as a white circle, the soil CO₂ composition (C_s) is a black circle, and the isotopic composition of production is a black square. Note that values for the isotopic composition of soil in the three panels are rounded for ease of reading but are actually −20.3832 ‰, −39.3989 ‰, and −48.4319 ‰, respectively, for panels (a–c). These values are drawn from the curve at a depth of 40 cm.

While ¹⁴C is a radioactive isotope and thus decays with time, the half-life is sufficiently long so that ¹⁴CO₂ behaves similarly to stable isotopes on the timescales at which diffusion occurs in a soil gas system. In this way, δ¹³C diffusive fractionation theory can be applied to the radiocarbon isotopic composition, δ¹⁴C, so long as we account for the mass difference. The larger mass of ¹⁴C means that the diffusion fractionation factor is calculated to be 8.8 ‰ based on the atomic masses of ¹⁴CO₂, ¹²CO₂, and bulk air (Southon, 2011).

We can show that the ¹⁴CO₂ distribution in soils will be like that of ¹³CO₂ if we model its distribution through depth in the same synthetic soil gas environment. In Fig. 1b we present a modeled soil environment with defined atmospheric and production source CO₂ isotopic composition boundary conditions for δ¹⁴C, the ¹⁴C equivalent to δ¹³C (Stuiver and Polach, 1977):

$$\begin{array}{}\text{(3)}& {\mathit{\delta }}^{\mathrm{14}}\mathrm{C}=\left({\displaystyle \frac{A_{\mathrm{s}}}{A_{\mathrm{abs}}}}-\mathrm{1}\right)\mathrm{1000},\end{array}$$

where δ¹⁴C is the isotopic composition in per mill, A_s is the measured activity of the sample, and A_abs is the activity of the oxalic acid standard (both unitless). As in Fig. 1a, in panel (b), the profile with lower soil diffusivity, the downward penetration of atmospheric CO₂ into the soil profile is reduced, and as a consequence the isotopic depth profile more closely reflects (but does not equal) the composition of production (−50 ‰; dashed line). When the diffusion rate is high and transport is rapid, the atmospheric source is more readily able to penetrate the profile and mix with the production source. In both profiles, the measured value of soil CO₂ at a given depth will not equal the isotopic production value of −50 ‰ because of diffusion and diffusive mixing. Similar profiles of δ¹⁴C of soil CO₂ with depth, highlighting the diffusive effects, have been presented by Wang et al. (1994).

Since δ¹⁴C transport of soil CO₂ is like that of δ¹³C, it follows that we should apply corrections for δ¹⁴C like those in Eq. (2) in order to calculate the isotopic composition of production. The δ¹⁴C reformulation of Davidson's ${\mathit{\delta }}_J^{\mathrm{13}}$ equation is as follows:

$$\begin{array}{}\text{(4)}& {\mathit{\delta }}_J^{\mathrm{14}}={\displaystyle \frac{C_{\mathrm{s}}\left({\mathit{\delta }}_{\mathrm{s}}^{\mathrm{14}}-\mathrm{8.8}\right)-C_{\mathrm{a}}({\mathit{\delta }}_{\mathrm{a}}^{\mathrm{14}}-\mathrm{8.8})}{\mathrm{1.0088}(C_{\mathrm{s}}-C_{\mathrm{a}})}},\end{array}$$

where ${\mathit{\delta }}_J^{\mathrm{14}}$ is the δ¹⁴C composition of soil production, C_s and ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{14}}$ are the mole fraction and δ¹⁴C composition of the soil CO₂, and C_a and ${\mathit{\delta }}_{\mathrm{a}}^{\mathrm{14}}$ are the mole fraction and δ¹⁴C composition of CO₂ in the air just above the soil. This Davidson reformulation for δ¹⁴C, ${\mathit{\delta }}_J^{\mathrm{14}}$, was applied to a model-generated profile of soil δ¹⁴C at a 40 cm depth in Fig. 2b, like in panel (a) for δ¹³C (C_s= 14 780 ppm, ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{14}}=-\mathrm{39.3989}$ ‰, C_a=380 ppm, and ${\mathit{\delta }}_{\mathrm{a}}^{\mathrm{14}}=\mathrm{45.5276}$ ‰; see inset box, Fig. 2b). As was the case for δ¹³C in Fig. 2a, the modeled values of C_s and ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{14}}$ at any depth will yield the true isotopic composition of production, −50 ‰ (dashed line), because this approach accounts for diffusion and diffusive mixing.

The typical approach that has been used for interpreting the ¹⁴C composition of soil CO₂ and soil-respired CO₂ (e.g., Trumbore, 2000) differs from the δ¹⁴C example above because a δ¹³C correction is applied to account for the mass-dependent isotopic fractionation of biochemical origin, ultimately converting δ¹⁴C to a variant called Δ¹⁴C (Stuiver and Polach, 1977). The derivation of the mass-dependent correction is provided in Stuiver and Robinson (1974), wherein observations are normalized to an arbitrary baseline value of −25 ‰ for δ¹³C (a value for terrestrial wood), and the ¹³C fractionation factors are squared to account for the ¹⁴C∕¹²C fractionation factor as follows:

$$\begin{array}{}\text{(5)}& \begin{array}{rl}{A}_{\mathrm{SN}}& =A_{\mathrm{s}}{\left[{\displaystyle \frac{R_{\mathrm{s}}\left(-\mathrm{25}\right)}{R_{\mathrm{s}}}}\right]}^{\mathrm{2}},\\ & =A_{\mathrm{s}}{\displaystyle \frac{{\left[\left(\mathrm{1}-\frac{\mathrm{25}}{\mathrm{1000}}\right)\times R_{\mathrm{VPDB}}\right]}^{\mathrm{2}}}{{\left[\left(\mathrm{1}+\frac{{\mathit{\delta }}^{\mathrm{13}}\mathrm{C}}{\mathrm{1000}}\right)\times R_{\mathrm{VPDB}}\right]}^{\mathrm{2}}}},\\ & =A_{\mathrm{s}}{\displaystyle \frac{{\left[\left(\mathrm{1}-\frac{\mathrm{25}}{\mathrm{1000}}\right)\right]}^{\mathrm{2}}}{{\left[\left(\mathrm{1}+\frac{{\mathit{\delta }}^{\mathrm{13}}C}{\mathrm{1000}}\right)\right]}^{\mathrm{2}}}},\end{array}\end{array}$$

where A_SN is the normalized sample activity, A_s is the sample activity, and δ¹³C is the isotopic composition of the sample (soil CO₂ in our case). As explained in Stuiver and Robinson (1974), the 0.975 term sometimes used in forms of A_SN is equivalent to $\left(\mathrm{1}-\frac{\mathrm{25}}{\mathrm{1000}}\right)$, which we will retain for clarity. The equation for Δ¹⁴C, the δ¹³C-corrected variant of δ¹⁴C, can then be created from Eq. (5) by substituting in delta notation for Δ¹⁴C of Δ¹⁴C =  (A_SN∕A_abs -1) × 1000 following Stuiver and Robinson (1974):

$$\begin{array}{}\text{(6)}& {\mathrm{\Delta }}^{\mathrm{14}}\mathrm{C}=\left[\left(\mathrm{1}+{\displaystyle \frac{{\mathit{\delta }}^{\mathrm{14}}\mathrm{C}}{\mathrm{1000}}}\right){\displaystyle \frac{{\left(\mathrm{1}-\frac{\mathrm{25}}{\mathrm{1000}}\right)}^{\mathrm{2}}}{{\left(\mathrm{1}+\frac{{\mathit{\delta }}^{\mathrm{13}}\mathrm{C}}{\mathrm{1000}}\right)}^{\mathrm{2}}}}-\mathrm{1}\right]\mathrm{1000}.\end{array}$$

Combining Eqs. (3) and (6) leads to

$$\begin{array}{}\text{(7)}& {\mathrm{\Delta }}^{\mathrm{14}}{\mathrm{C}}_{\mathrm{old}}=\left[\left({\displaystyle \frac{A_{\mathrm{s}}}{A_{\mathrm{abs}}}}\right){\displaystyle \frac{{\left(\mathrm{1}-\frac{\mathrm{25}}{\mathrm{1000}}\right)}^{\mathrm{2}}}{{\left(\mathrm{1}+\frac{{\mathit{\delta }}^{\mathrm{13}}\mathrm{C}}{\mathrm{1000}}\right)}^{\mathrm{2}}}}-\mathrm{1}\right]\mathrm{1000}.\end{array}$$

For more information on the derivation of Eqs. (6) and (7) see Stuiver and Robinson (1974), page 88. In Eq. (7) we have added the subscript “old” to highlight that this is the common approach used to date for soil gas applications – we will introduce a “new” method with Eq. (15). The terms on the left-hand side of Eqs. (6) and (7) are identical. Note that A_abs in our notation is equivalent to A_O in Stuiver and Robinson (1974).

Equation (7) uses δ¹³C as an input parameter to make a mass-dependent correction to obtain Δ¹⁴C, but the profiles of δ¹³C and δ¹⁴C of soil CO₂ (Fig. 1) highlight the fact that both vary within the soil because of diffusion and diffusive mixing. This makes it unclear what form of δ¹³C should actually be used in the place of the mass-dependent correction in the soil gas environment (δ¹³C of the soil CO₂ is measured but δ¹³C of biological production is not) as diffusive mixing is not a mass-dependent process. When Δ¹⁴C_old is modeled through depth like δ¹³C and δ¹⁴C in Figs. 1 and 2 it also varies with depth as shown in Fig. 2c. However, using a Δ¹⁴C variant of Davidson's δ_J (as for δ¹⁴C in Fig. 2b) at the same 40 cm depth does not correctly reproduce the specified model value for the Δ¹⁴C of production of −50 ‰ like it did for δ¹³C and δ¹⁴C (C_s= 14 780 ppm, ${\mathrm{\Delta }}_{\mathrm{s}}=-\mathrm{48.4319}$ ‰, C_a=380 ppm, and Δ_a=10 ‰; see inset box, 2c). We therefore adapted the mass-dependent correction in Δ¹⁴C_old using Davidson's (1995) theory to demonstrate how and why it should be used for Δ¹⁴C soil gas applications.

3 Methods – model description

We used an analytical gas transport model to simulate a range of natural soil profiles of ¹²CO₂, ¹³CO₂, and ¹⁴CO₂ in order to present soil gas transport theory. The model is based on Fick's second law of diffusion:

$$\begin{array}{}\text{(8)}& \mathit{\theta }{\displaystyle \frac{\partial \mathrm{Conc}}{\partial t}}={\displaystyle \frac{\partial }{\partial z}}\left(D\left(z,t\right){\displaystyle \frac{\partial \mathrm{Conc}}{\partial z}}\right)+P\left(z,t\right),\end{array}$$

where θ is the soil air-filled pore space, Conc is the concentration, t is time, D(z,t) is the soil gas diffusion function, and P(z,t) is the biological production function, with the latter two dependent on both depth z and time t.

The model was run in steady state,

$$\begin{array}{}\text{(9)}& {\displaystyle \frac{\partial \mathrm{Conc}}{\partial t}}=\mathrm{0},\end{array}$$

and both diffusion and production rates were constant with depth:

$$\begin{array}{}\text{(10)}& {\displaystyle }& {\displaystyle }D\left(z\right)=D,\text{(11)}& {\displaystyle }& {\displaystyle }P\left(z\right)=P.\end{array}$$

The following boundary conditions were used:

$$\begin{array}{}\text{(12)}& {\displaystyle }& {\displaystyle }C\left(z=\mathrm{0}\right)={\mathrm{Conc}}_{\mathrm{atm}},\text{(13)}& {\displaystyle }& {\displaystyle \frac{\partial C}{\partial z}}{\mathrm{|}}_{z=L}=\mathrm{0},\end{array}$$

where Conc_atm is the concentration of CO₂ in air just above the soil and L is the model lower spatial boundary, the point below which no production or diffusion occurs. Equation (8) is solved analytically to yield the following equation:

$$\begin{array}{}\text{(14)}& \mathrm{Conc}\left(z\right)={\displaystyle \frac{P/L}{D}}\left(L\times z-{\displaystyle \frac{z^{\mathrm{2}}}{z}}\right)+{\mathrm{Conc}}_{\mathrm{atm}}.\end{array}$$

In the model, isotopologues of CO₂ are treated as independent gases, with their own specific concentration gradients and diffusion rates (Cerling et al., 1991; Risk and Kellman, 2008; Nickerson and Risk, 2009). We assume total CO₂ to be ¹²CO₂ because of its high abundance. The error associated with this assumption is less than 0.01 % (Amundson et al., 1998). Equation (14) is thus applied for ¹³CO₂ and ¹⁴CO₂. For the full derivation see Nickerson et al. (2014) Sect. 2.3.

The analytical gas transport model was applied across a range of soil diffusivity ($\mathrm{1}\times {\mathrm{10}}^{-\mathrm{7}}$, $\mathrm{1}\times {\mathrm{10}}^{-\mathrm{6}}$, and $\mathrm{1}\times {\mathrm{10}}^{-\mathrm{5}}$ m² s⁻¹) and soil production rates (0.5, 1, 2, and 4 µmol CO₂ m⁻³ s⁻¹). The specific soil diffusivity and production rates used to generate each profile are stated in the figure caption of that profile. We used a δ¹³C of biological production (−25 ‰) and atmospheric CO₂ (δ_a; −8‰) and Δ¹⁴C of biological production (−50 ‰) and atmospheric CO₂ (Δ_a; 10 ‰) to represent realistic conditions found in nature. The other model boundary conditions were as follows: L=0.8 m, z=0.025 m, and Conc_atm= 15 833 µmol m⁻³ (∼380 ppm). The output of the model under these applied conditions were profiles of ¹²CO₂, ¹³CO₂, and ¹⁴CO₂ for each depth (z) down to the bottom boundary (L).

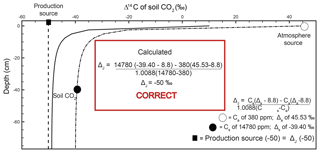
Figure 3 Modeled steady-state diffusive vertical depth profiles for Δ¹⁴C_old (solid line; same profile as in Fig. 2c) and Δ¹⁴C_new (dashed line) of soil CO₂. The Δ¹⁴C_new soil profile was calculated from the profiles in Fig. 2 (soil production and diffusivity rates of 2 µmol m⁻³ s⁻1 and $\mathrm{1}\times {\mathrm{10}}^{-\mathrm{6}}$ m² s⁻¹, respectively). The inset “Calculated” panels show how, using input data read directly from the depth profile of Δ¹⁴C_new, a user would arrive at the correct value of production using a Davidson approach to adjust for in-soil gas transport. The atmospheric source (C_a) composition is presented as a white circle, the soil CO₂ composition (C_s) is a black circle, and the isotopic composition of production is a black square. Note that values for the isotopic composition of soil and atmosphere are rounded for ease of reading but are actually −39.3989 ‰ and 45.5276 ‰, respectively. These values are drawn from the curve at a depth of 40 cm.

4 Results

4.1 Adapted correction for interpreting radiocarbon values of soil and soil-respired CO₂

Based on Davidson's (1995) theory and what we demonstrated with Fig. 2c, rather than using the δ¹³C soil pore space as a mass-dependent correction, we suggest instead using the value ${\mathit{\delta }}_J^{\mathrm{13}}$ (Eq. 2), the biological production of δ¹³C, in its place in the denominator of Eq. (7) as follows:

$$\begin{array}{}\text{(15)}& {\mathrm{\Delta }}^{\mathrm{14}}{\mathrm{C}}_{\mathrm{new}}=\left[\left({\displaystyle \frac{A_{\mathrm{s}}}{A_{\mathrm{abs}}}}\right){\displaystyle \frac{{\left(\mathrm{1}-\frac{\mathrm{25}}{\mathrm{1000}}\right)}^{\mathrm{2}}}{{\left(\mathrm{1}+\frac{{\mathit{\delta }}_J^{\mathrm{13}}}{\mathrm{1000}}\right)}^{\mathrm{2}}}}-\mathrm{1}\right]\mathrm{1000}.\end{array}$$

Values of Δ¹⁴C_new through depth represent transport-fractionation-corrected soil CO₂ values of radiocarbon, and in comparison to Δ¹⁴C_old, they are corrected for mass-independent diffusive mixing.

A depth profile of Δ¹⁴C_new is presented in Fig. 3 (dashed line). To generate this soil depth profile we used the numbers from the simulated profiles in Fig. 2 and inserted them into Eq. (2) to determine ${\mathit{\delta }}_J^{\mathrm{13}}$ at each depth. These values were then used in Eq. (15) to obtain Δ¹⁴C_new of soil CO₂ through depth. The Δ¹⁴C_new profile (dashed line) is more isotopically enriched compared to the Δ¹⁴C_old profile (solid line) in Fig. 3. Values sampled from the Δ¹⁴C_old profile (the same as the one presented in Fig. 2c) were not able to reproduce the specified model value for the Δ¹⁴C of production of −50 ‰ using a Δ¹⁴C variant of Davidson's δ_J. To demonstrate that Δ¹⁴C_new does correct for gas transport fractionations, it can be placed into ${\mathrm{\Delta }}_J^{\mathrm{14}}$, a Δ¹⁴C adaption of Davidson (1995) for ¹⁴C (Eq. 4), as follows:

$$\begin{array}{}\text{(16)}& {\mathrm{\Delta }}_J^{\mathrm{14}}={\displaystyle \frac{C_{\mathrm{s}}\left({\mathrm{\Delta }}^{\mathrm{14}}{\mathrm{C}}_{\mathrm{new}}-\mathrm{8.8}\right)-C_{\mathrm{a}}\left({\mathrm{\Delta }}_{\mathrm{a}}^{\mathrm{14}}-\mathrm{8.8}\right)}{\mathrm{1.0088}(C_{\mathrm{s}}-C_{\mathrm{a}})}},\end{array}$$

where ${\mathrm{\Delta }}_J^{\mathrm{14}}$ is the Δ¹⁴C composition of soil production, C_s and Δ¹⁴C_new are the mole fraction and Δ¹⁴C composition of the soil CO₂, and C_a and ${\mathrm{\Delta }}_{\mathrm{a}}^{\mathrm{14}}$ are the mole fraction and Δ¹⁴C composition of CO₂ in the air just above the soil.

Unlike in the case of Δ¹⁴C_old demonstrated in the inset box in Fig. 2c, using the same 40 cm depth from the Δ¹⁴C_new profile, we were able to reproduce the specified model value for the Δ¹⁴C of production of −50 ‰ (C_s= 1 780 ppm, ${\mathrm{\Delta }}_{\mathrm{s}}=-\mathrm{39.3989}$ ‰, C_a=380 ppm, and Δ_a=45.5276 ‰; see inset box, Fig. 3).

4.2 Work-arounds and establishing new best practice

In the soil gas environment, Δ¹⁴C_new convention should be used to properly interpret soil-respired CO₂ from soil CO₂, as it corrects for all related transport fractionations. For researchers who have soil CO₂ data previously interpreted using Δ¹⁴C_old, the following steps will help correct for transport fractionations: (1) use ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{13}}$ and Δ¹⁴C_old to back out the activity of the sample (A_s); (2) calculate the isotopic composition of production for δ¹³C using Eq. (2), ${\mathit{\delta }}_J^{\mathrm{13}}$; (3) use ${\mathit{\delta }}_J^{\mathrm{13}}$ and A_s in Eq. (7) to calculate Δ¹⁴C_new; and finally (4) determine the radiocarbon isotopic composition of production using Eq. (16), ${\mathrm{\Delta }}_J^{\mathrm{14}}$.

Going forward, several changes to best practice are recommended. On a lab level, for new soil CO₂ data, we propose that laboratories report radiocarbon using Eq. (3) for δ¹⁴C, the uncorrected radiocarbon variant, so that the first step above (use ${\mathit{\delta }}_{\mathrm{s}}^{\mathrm{13}}$ and Δ¹⁴C_old to back out the activity of the sample; A_s) can be avoided, and researchers can proceed with steps 2–4. We also suggest that researchers measure δ¹³ alongside Δ¹⁴C so that they are not dependent on the AMS-measured δ¹³ for potential back corrections.

The Davidson (1995) δ_J method was the gradient approach we used in our study, but alternative gradient approaches, such as those presented for δ¹³C by Goffin et al. (2014) and Nickerson et al. (2014) and for Δ¹⁴C by Phillips et al. (2013), would likely be similarly successful in producing depth-dependent compositions of production. They are, however, not quite as straightforward as the δ_J method.

5 Conclusions

This traditional Δ¹⁴C solution, which uses δ¹³C of soil CO₂ as a mass-dependent correction, is not appropriate for the soil gas environment, as it does not account for mass-independent mixing processes. We propose a new best practice for Δ¹⁴C work in the soil gas environment that accounts for gas transport fractionations and produces true estimates of Δ¹⁴C of production.