Multispecies expression of coccolithophore vital effects with changing CO₂ concentrations and pH in the laboratory with insights for reconstructing CO₂ levels in geological history

The coccolith sedimentary and micropalaeontological archive has fostered great interest in palaeoclimate applications. Indeed, the geochemistry of coccolith calcite has the potential to reconstruct both palaeo-CO₂ concentrations and palaeo-temperature of seawater. Studying coccolith geochemistry aims at better understanding the changes in the vital effect of coccoliths with changes in environmental parameters, especially the carbonate chemistry of seawater. To this aim, we need to deconvolve the biological imprint from the environmental signals recorded in the composition of coccoliths. We have undertaken culture experiments of four coccolithophore strains with various sizes and growth rates, grown under eight CO₂/pH conditions typifying the CO₂ evolution of the Cenozoic era. We propose an assessment of the expression of the vital effects for Emiliania huxleyi, Gephyrocapsa oceanica, Helicosphaera carteri and Coccolithus braarudii with simultaneous changes in dissolved inorganic carbon (DIC) and pH in the medium resulting in variations in dissolved CO₂ (CO_2 aq) availability to the cells. We have identified a distinct isotopic response of C. braarudii to pCO₂ levels on both sides of the 600 ppmv (pH 7.89) condition. We propose that this discrepancy is the result of a modification of the proton efflux across the plasma membrane through voltage-dependent proton channels. We further show that as the CO₂ level rises and pH decreases (from 200 to 500 ppmv and from 8.29 to 7.96 pH units, respectively), a significant increase in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of C. braarudii is expressed, along with a coeval decrease in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$. The constant physiological parameters of C. braarudii (growth rate, particulate inorganic carbon (PIC) and particulate organic carbon (POC)) across the 200 to 500 ppmv interval support the idea that the change in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ is the consequence of a lower fractionation between dissolved CO₂ and organic matter. Meanwhile, the small cells of E. huxleyi and G. oceanica are less carbon-limited and do not exhibit any change in their carbon vital effects with changes in carbonate chemistry of the environment across the whole CO₂ spectrum. Using this biogeochemical framework, we have established a calibration between CO_2 aq concentration and the differential vital effect (Δδ¹³C) between isotopically invariant small G. oceanica and large coccoliths C. braarudii, whose vital effect is CO₂-dependent at low CO₂. The CO₂–Δδ¹³C transfer equation allows palaeo-pCO₂ reconstructions based on isotope changes explained by physiological processes, especially at low and medium CO₂ levels.

1 Introduction

The atmospheric concentration of carbon dioxide (pCO₂) is a key parameter controlling global climate through its radiative forcing on Earth's surface temperatures. The reconstruction of past pCO₂ is challenging to handle as many caveats exist in palaeo-CO₂ barometry methods. The magnitude of the carbon isotope fractionation between membrane lipids called alkenones produced by the coccolithophores – found in sediments – and ambient aqueous CO₂ constitutes the basis of the foremost palaeo-pCO₂ proxy, referred to as ε_p-alk (Pagani et al., 2010; Zhang et al., 2019, 2020). This approach has led to significant advances in the understanding of the past CO₂ concentration, but its application still has some limitations. Conversely to the coccolith archive, alkenone molecules are not ubiquitously preserved in marine sediments, and the assessment of pCO₂ involves a cascade of calculations requiring assumptions made on the δ¹³C value of CO_2 aq. Furthermore, this method has recently been shown to overestimate pCO₂ below 270 ppmv (Badger et al., 2019), potentially leading to underestimated pCO₂ above 270 ppmv. Alongside this proxy, the boron isotope fractionation in foraminifera tests has increasingly been used to reconstruct palaeo-pH (Rae et al., 2021). Combined with another parameter of the carbonate chemistry system as dissolved inorganic carbon (DIC) or alkalinity, and with temperature, palaeo-pH can be used for palaeo-pCO₂ reconstructions (Foster, 2008; Sanyal et al., 1995). This proxy also suffers from uncertainties due to the evaluation of δ¹¹B of past seawater among other unknown factors (Klochko et al., 2006; Tripati et al., 2011).

In this study, we investigated the fractionation of carbon and oxygen isotopes in phytoplanktonic organic matter and coccolith calcite driven by changes in the carbonate chemistry of coccolithophores culture media. We have conducted in vivo culture experiments that provide a means to constrain the cellular and isotopic responses of coccolithophores to environmental changes. Our culture experiments were conducted to quantify the isotopic departure of coccoliths from inorganic calcite, called the vital effect. The latter only depends on physico-chemical parameters of the environment such as $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{sw}}$, $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{{\mathrm{CO}}_{\mathrm{2}}}$, temperature, salinity and pH (Zeebe and Wolf-Gladrow, 2001). However, when calcite is biomineralised intracellularly, biological parameters such as growth rate, cell size and the PIC$/$POC ratio – which refers to the distribution of carbon between particulate organic carbon (POC) and particulate inorganic carbon (PIC) produced by calcifying organisms – also influence this fractionation (Dudley et al., 1986; McClelland et al., 2017; Rickaby et al., 2010). The causes behind the changes in the isotopic fractionation between the different cellular compartments are to be investigated at various stages of carbon fixation. Aqueous CO₂, the main form of DIC entering coccolithophorid cells, diffuses through the plasma membrane via passive diffusion (Gutknecht et al., 1977). Aqueous CO₂ diffusion is governed primarily by Fick's first law but also by the specific conditions of the cell boundary layer on the external side of the plasma membrane (Reinfelder, 2011; Wolf-Gladrow and Riebesell, 1997). In addition to passive CO₂ diffusion, certain taxa of coccolithophores such as Emiliania possess CCMs (carbon concentration mechanisms), allowing the intake of ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$ through active transmembrane transport (Bach et al., 2013; Parker and Boron, 2013; Romero et al., 2004). As ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$ is enriched in ¹³C compared to CO₂, the greater the acquisition of ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$, the higher the δ¹³C values in the intracellular DIC pool and ultimately the higher the δ¹³C of coccolith calcite.

Once inside the cell, carbon is utilised by two main pathways: the fixation into organic matter via photosynthesis in chloroplasts and the precipitation of calcite-forming coccoliths within the coccolith vesicle. Carbon fixation into organic matter by the enzyme RuBisCO (ribulose 1,5-bisphosphate carboxylase/oxygenase) (Ellis, 1979) leads to fractionation as it preferentially fixes the lighter isotopes (¹²C) (Laws et al., 2001; Popp et al., 1998). Consequently, organic matter is relatively depleted in ¹³C (very negative $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$) compared to the carbon source and compared to the intracellular DIC pool (Bidigare et al., 1997; Guy et al., 1993; Rau et al., 1996). The fractionation induced by RuBisCO is one component of a more general fractionation between the CO_2 aq from the external environment and the organic matter synthesised within the cell, which leads to an enrichment, denoted ε_p (Jasper and Hayes, 1990; Pagani et al., 1999). In addition to being dependent on the fractionation associated with RuBisCO, ε_p is influenced by physiological parameters such as growth rate, cell size and the presence of CCMs (Laws et al., 1995; Popp et al., 1998).

In this work, we provide both $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ values with changes in pCO₂ and pH to explain the mechanisms responsible for the carbon isotope fractionation within the cell. The present study stems from several lines of evidence that the isotopic offset between coccoliths of different sizes conveys a specific pCO₂ signal, as shown in sedimentary records by the work of Bolton and Stoll (2013). This empirical observation has been repeatedly reported in culture data (Hermoso, 2015; Hermoso et al., 2016a; McClelland et al., 2017; Rickaby et al., 2010). This approach used to derive palaeo-CO₂ concentrations still needs to be fully constrained by implementing new cultures with a view to consolidating the calibration, although a proof of concept exists (Bolton and Stoll, 2013; Godbillot et al., 2022; Hermoso et al., 2016a, 2020; Tremblin et al., 2016). Modelling studies fed by culture data have identified and quantified the main forcing parameters behind the magnitude of the carbon isotope vital effect in coccolith calcite: growth rate, cell size, and the partitioning of CO₂ in particulate inorganic matter and particulate organic matter (PIC$/$POC ratio), among other ancillary parameters (McClelland et al., 2017).

Four strains of geological-relevant coccolithophores, representing a wide diversity of growth rate and coccolith and cell sizes, were cultured to study how the composition of the culture medium (DIC, pH) influences the magnitude of coccolith vital effects and to establish transfer functions between these vital effects and the aqueous CO₂ concentrations and pH. In contrast with previous culture studies and to accurately mimic the carbonate chemistry of the ocean through the Cenozoic era, we cultured these calcifying microalgae with coupled pCO₂ and pH perturbations of the medium. The culture conditions varied from 200 ppmv and 8.29 pH units (pre-industrial) to 1400 ppmv and 7.55 pH units (thought to represent the mid-Eocene levels), keeping all other parameters constant. Particular attention has been paid to conditions similar to those of the last 12 Myr (narrow step of 100 ppmv for pCO₂ between 200 and 500 ppmv) because of the scarcity of available data.

2 Materials and methods

2.1 Strains

We cultured four coccolithophore strains kindly provided by the biological station of Roscoff: RCC1200 Coccolithus braarudii, RCC1323 Helicosphaera carteri, RCC1314 Gephyrocapsa oceanica and RCC1256 Emiliania huxleyi (Fig. 1). These species represent a wide range of coccolith taxa with various coccosphere and cell sizes. The rationale of this choice is that they belong to the most abundant groups found in Neogene pelagic sediments (Bolton et al., 2012; Claxton et al., 2022).

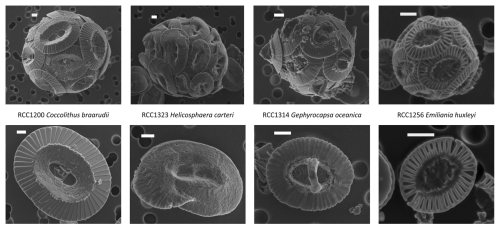
Figure 1 Scanning electron microscope (SEM) images of the four studied strains. Top images show the coccospheres, and bottom images show the coccoliths. The scale bars represent 1 µm.

If comparable with our own study (mode of culture, temperature, pH, etc.), the results of previously published biogeochemical work on the same species will be presented along with our own data in the figures.

2.2 Medium preparation

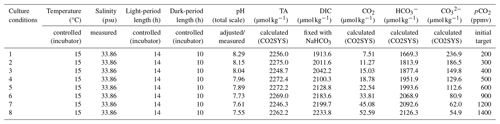
Our culture experiments were designed to represent pH and pCO₂ from the last greenhouse period (Eocene) to the pre-industrial Holocene oceanic conditions (Rae et al., 2021; Sosdian et al., 2018). Variable pCO₂ levels between 200 and 1400 ppmv at a constant alkalinity of 2300 µmol kg⁻¹ have been used to calculate the target pH (total scale) with the CO2SYS program (Lewis and Wallace, 1998). See Table 2 for details on the chemical parameters of the culture medium.

Artificial seawater with a salinity of 34 psu was prepared following the ESAW recipe of Berges et al. (2001). The use of artificial seawater was preferred over natural seawater in order to facilitate the production of the medium with the CO₂ level under 400 ppmv. Phosphate, nitrate, silica, Fe-EDTA, trace metals and vitamins were added to produce K/2 media according to Keller et al. (1987). The DIC concentration was reached by adding different amounts of NaHCO₃ (Sigma – batch CAS 144-55-8) (Table 1). The equivalence between the quantity of NaHCO₃ added and the resulting DIC concentration was checked beforehand with a Picarro G2131-i isotope and gas concentration analyser coupled with an Apollo SciTech AS-D1 DIC-δ¹³C analyser at LOCEAN laboratory (Sorbonne University) and by potentiometric dosing at the SNAPO-CO2 service facility at LOCEAN laboratory (Sorbonne University). The pH values were checked by electrometric measurement using a Mettler Toledo pH InLab^® Routine pro-ISM electrode. Calibration was performed using a T34 TRIS buffer solution (Del Valls and Dickson, 1998). The pH was adjusted with HCl and NaOH addition until the target pH was reached. This treatment led to a change in the relative abundance of the DIC species (see Bjerrum plot of carbonate speciation versus pH, Zeebe and Wolf-Gladrow, 2001). Lastly, the medium was sterilised with a 0.22 µm filtering step and stored without headspace in amber-coloured bottles at 15 °C in the dark.

Table 1 Amount of NaHCO₃ added to prepare the different media used in this study (conditions 1 to 8). The amount of NaHCO₃ corresponds to the production of 1 L of artificial seawater.

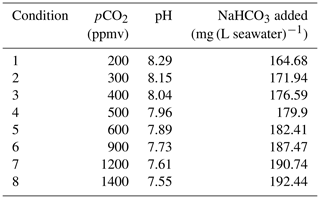
Table 2 Experimental parameters of the mediums. The salinity has been measured in the initial medium. The temperature and the light- and dark-period length are controlled by an incubator setup. The total alkalinity (TA) and the DIC, CO₂, ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$ and ${{\mathrm{CO}}_{\mathrm{3}}}^{\mathrm{2}-}$ concentrations are calculated by the CO2SYS program. pCO₂ and pH correspond to the culture conditions tested.

2.3 Culture growth

The cultures were undertaken at the Maison de la Recherche en Environment Naturel (ULCO – LOG) in Wimereux (northern France) in 2023–2024. The coccolithophore strains were first acclimatised to the medium during about 10 generations in 25 cm² polystyrene flasks. The cells were then inoculated in triplicate experiments in culture bottles of increasing size (75 cm² polystyrene flasks, 600 and 2300 mL polycarbonate bottles) until the target cell number of the culture was reached. The target cell number corresponds to a sufficient biomass for our analyses (between 2 and 10 mg for the small cells E. huxleyi and G. oceanica and between 50 and 60 mg for the large cells H. carteri and C. braarudii), while ensuring that the cultures remained adequately diluted (less than 8000 cells mL⁻¹ for C. braarudii and for H. carteri and less than 20 000 cells mL⁻¹ for E. huxleyi and for G. oceanica, in accordance with Riebesell et al., 2011).

The cell cultures were maintained at constant temperature (15 °C) with a 14–10 day–night cyclicity and an irradiance of 150 $\mathrm{\mu }\mathrm{mol}\mathrm{photons}{\mathrm{m}}^{-\mathrm{2}}{\mathrm{s}}^{-\mathrm{1}}$. The cells were regularly resuspended to avoid cell clustering and kept at relatively low concentrations to maintain homogeneous carbon bioavailability and light access during all the experiment. A daily control of the culture health was made with a reverse optical microscope under ×400 magnification. A final control of the coccoliths was made after the harvest with a Zeiss Supra 55 VP scanning electron microscope (SEM) at Sorbonne University.

Cell numeration and coccosphere sizes were measured using a Beckman Multisizer 4e Coulter counter calibrated with 10.16 µm latex beads. The measurements were always performed at the same time of the day (08:30–10:00 LT) to avoid biases related to the growth of the cell during the day phase.

The growth rate μ (in d⁻¹) of a microorganism culture corresponds to the increase in the cell number by time units commonly calculated with the formula:

$$\begin{array}{}\text{(1)}& \mathit{\mu }\left({\mathrm{d}}^{-\mathrm{1}}\right)={\displaystyle \frac{\ln \left(c_{\text{final}}\right)-\ln \left(c_{\text{initial}}\right)}{t_{\text{final}}-t_{\text{initial}}}},\end{array}$$

where c is the cell concentration, and t_final−t_initial is the number of days between the initial and the final cell concentration measurements. To take into account all the concentration measurements, the μ was derived from the slope of the linear regression of the function $f(t_x-t_{\text{initial}})=\ln \left(c_x\right)$, where x is the day of the measurement of c_x.

2.4 Isotopic reference of the culture medium

The δ¹³C of the dissolved inorganic carbon ($\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{DIC}}$) and the DIC concentrations were measured in 11 samples of various CO₂ and pH levels with a Picarro G2131-i isotope and gas concentration analyser coupled with an Apollo SciTech AS-D1 DIC-δ¹³C analyser at LOCEAN laboratory (Sorbonne University). The medium samples were preserved in 500 mL glass bottles with a ground neck with 0.3 mL saturated HgCl₂ solution. The measurements are calibrated with in-house standards. Since we used the same NaHCO₃ powder batch for the different experiments, all the culture media had the same $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{DIC}}$ values (−12.17 ‰ Vienna Pee Dee Belemnite (V-PDB) (±0.07 ‰)).

The δ¹⁸O of seawater was measured by cavity ring-down spectroscopy (CRDS) using a Picarro instrument (model L2130-i Isotopic H₂O) at LOCEAN, Sorbonne University. The in-house standards (freshwater) are calibrated using IAEA references V-SMOW (Vienna Standard Mean Ocean Water) and GISP (Greenland Ice Sheet Precipitation). The δ¹⁸O of the inorganic reference ($\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\mathrm{i}}$) in ‰V-PDB is calculated from the $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{sw}}$ and temperature (15 °C) according to the equation of Kim and O'Neil (1997), modified by Tremblin et al. (2016). The $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{sw}}$ slightly changed between successive culture campaigns: −6.44 ‰ V-SMOW (±0.03 ‰) for C. braarudii and G. oceanica, −6.67 ‰ V-SMOW (±0.03 ‰) for H. carteri, and −6.36 ‰ V-SMOW (±0.15 ‰) for E. huxleyi.

The oxygen vital effects are calculated as the difference between the δ¹⁸O of the coccoliths (VE¹⁸O) in ‰ V-PDB and the δ¹⁸O of a theoretical inorganic calcite ($\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\mathrm{i}}$) in ‰V-PDB:

$$\begin{array}{}\text{(2)}& \text{VE}{}^{\mathrm{18}}\mathrm{O}=\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccoliths}}-\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\mathrm{i}},\end{array}$$

2.5 Isotopic analysis on calcite

Two methods were used to collect the culture residues for further isotopic measurements of coccolith calcite:

• RCC1200 C. braarudii and RCC1323 H. carteri culture residues were centrifuged during 15 min at 4500 rpm (relative centrifugal force of 4302×g) in 500 mL centrifuge bottles. After removing the supernatant, samples were rinsed with three cycles of centrifugation with replacement of the supernatant with neutralised demineralised water and homogenisation between each cycle. The rinsing is employed to remove the salt from the culture water. The culture residues were finally dried at 40 °C after the removal of the last supernatant.

• RCC1256 E. huxleyi and RCC1314 G. oceanica samples were collected and rinsed three times with neutralised demineralised water onto polycarbonate filtering membranes and then dried in a desiccator. We applied a different harvesting method for those strains because of their small size, which induced too much material loss during the centrifugation process. All culture residues were stored at 5 °C after harvest.

Isotopic measurements on calcite were performed at the ISTeP laboratory (Sorbonne University). Between 30 and 60 µg of the samples were digested with 100 % phosphoric acid at 70 °C in pre-evacuated vials using a Kiel IV carbonate device. The evolved CO₂ was purified in a cryogenic trapping system, and carbon and oxygen isotope compositions were measured in an isotope-ratio mass spectrometer DELTA V advantage (Thermo Scientific) with a dual-inlet introduction system. The carbon and oxygen isotope compositions were expressed in the delta notation as a value relative to the Vienna Pee Dee Belemnite (V-PDB) and reported as per mil (‰). δ¹³C and δ¹⁸O values were calibrated using NBS-19 and NBS-18 international standards. Internal reproducibility and accuracy were monitored by replicate analysis of in-house calcite standard Marceau (δ¹³C=2.12 ‰ and $\mathit{\delta }{}^{\mathrm{18}}\mathrm{O}=-\mathrm{1.87}\mathrm{‰}$), the measured values for δ¹³C and δ¹⁸O being 2.10 ‰ ± 0.08 ‰ (1σ) and −1.89 ‰ ± 0.10 ‰ (1σ), respectively. The external reproducibility, obtained from replicate analysis of the samples, is better than 0.05 ‰ (1σ) for $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ and 0.10 ‰ (1σ) for $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$. Transfer equations between coccolith isotope ratios and CO₂ levels are proposed in this study. The residual errors (also accounting for pH/CO₂ uncertainties) are evaluated through Monte Carlo analysis code with 1 000 000 iterations and an uncertainty of 0.17 ‰ for the differential vital effect between small and large coccoliths $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ (1σ).

2.6 Carbon isotope analysis of the organic matter

The culture residues of C. braarudii, G. oceanica and E. huxleyi were gathered onto glass microfiber filters (GFFs), previously burnt at 400 °C for 3 h to remove all trace of residual organic matter. After gathering the culture residues, the GFFs were rinsed three times with neutralised demineralised (Millipore^®) water (adjusted to pH 7 by adding sodium hydroxide) and then stored at −18 °C. We then gently scratched the superficial part of the GFFs to collect all the culture residue. The culture residues were decarbonated with hydrochloric acid 2 N overnight. The samples were then rinsed to eliminate the excess of hydrochloric acid and released Ca²⁺ and alkalinity and then dried at 35 °C. Between 10 and 50 µg of decarbonated culture residue (only organic matter) was weighted and loaded into tin capsules.

The carbon isotope composition of the organic matter was measured at the Stable Isotope Geochemistry laboratory at IPGP (Institut de Physique du Globe de Paris). A Flash EA 1112 elemental analyser coupled in continuous helium flow to a Thermo Fisher Scientific DELTA V Plus isotope ratio mass spectrometer was used for the C. braarudii samples cultured between 200 and 500 ppmv (8.29 and 7.96 pH units). For G. oceanica and E. huxleyi for all culture conditions, and for C. braarudii between 500 and 1400 ppmv (7.96 and 7.55), we used an Elementar vario PYRO cube analyser coupled with the same DELTA V Plus spectrometer at IPGP. Three organic-rich internal standards, calibrated against international standards, were used to calculate the δ¹³C values of the samples, reported as per mil with respect to V-PDB. Replicate analysis of standards yielded an internal reproducibility better than 0.10 ‰ (1σ). Accuracy was assessed by measuring an in-house Tyrosine standard ($\mathit{\delta }{}^{\mathrm{13}}\mathrm{C}=-\mathrm{23.23}\mathrm{‰}$), for which the estimated error was better than 0.20 ‰. The external reproducibility for δ¹³C_org, obtained from replicate analysis of the samples, is better than 0.07 %.

2.7 Carbon content analysis

The PIC : POC ratios of the four strains studied under various pCO₂ and pH conditions were measured at the Wimereux marine station using a Thermo Fisher Flash 2000 elemental analyser. The culture residues were collected and stored in the same way for analyses of the δ¹³C_org. The calibration used for reconstructing carbon content was done with various amounts of acetanilide with a known amount of carbon and nitrogen. Samples for POC analysis were previously acidified. The filters were encapsulated in a tin disc before analysis.

3 Results

3.1 Physiological parameters and carbon content

3.1.1 Growth rates

The growth rates of the four strains do not show any statistical trend with changes in the carbonate chemistry of the medium (C. braarudii: r²=0.04 and p>0.05, H. carteri: r²=0.08 and p>0.05, G. oceanica: r²=0.11 and p>0.05, E. huxleyi: r²=0.38 and p<0.01) (Fig. 2). In particular, the linear regression between replicated values of growth rates and CO₂ concentration below 600 ppmv gives a p value of 0.052 and a r² of 0.41. More than 85 % of the data are within a ±15 % relative range of the mean growth rate for each strain (coloured band in Fig. 2). E. huxleyi and G. oceanica have the higher mean growth rate (0.87 and 0.67 d⁻¹, respectively), while the larger C. braarudii and H. carteri have a mean growth rate of 0.58 and 0.24 d⁻¹, respectively (Fig. 2). These absolute values are similar to those obtained in previous studies for E. huxleyi, G. oceanica and C. braarudii (Phelps et al., 2021; Rickaby et al., 2010). C. braarudii has been reported with decreasing growth rates with rising CO₂ levels (Hermoso et al., 2016a; Rickaby et al., 2010).

Figure 2 Specific growth rate (μ) of the four cultured strains with respect to CO₂ level and pH of the culture media. The empty dots show all the replicate data, and the filled dots denote the mean growth rates. Growth rates are given per day (d⁻¹) (left axis) and as a relative deviation of the mean growth rate of each strain (right axis). The coloured bands correspond to the range of ±15 % of variation from the mean growth rate. More than 85 % of the data are within this range.

3.1.2 Coccosphere sizes

The sizes of the coccospheres – i.e. the coccolithophore exoskeletons surrounding the cells, composed of coccoliths – approximate the evolution of cell size. The evolution of this parameter with CO₂ and pH varies among the different strains. C. braarudii displays the largest coccospheres amongst the cultured strains. The mean coccosphere diameter of C. braarudii increases from 16.7 to 18.4 µm with increasing CO₂ levels from 200 to 600 ppmv and decreasing pH from 8.29 to 7.89 units of pH. The +1.70 µm increase in the coccosphere size represents a relative variation of 10.2 % (r²=0.86, p<0.01). For higher pCO₂ and lower pH, the distribution of the coccosphere sizes is not linked with the carbonate chemistry (Fig. 3). The size of H. carteri presents a hyperbolic trend (r²=0.72, p<0.01) of the coccosphere size with increasing CO₂ level and decreasing pH (+0.69 µm from 200 ppmv and 8.29 pH units to 600 ppmv and 7.89 pH units and −1.05 µm from 600 ppmv and 7.89 pH units to 1400 ppmv and 7.55 pH units) (Fig. 3). The size of the coccospheres produced by G. oceanica decreases from 8.0 to 7.5 µm with increasing CO₂ levels from 200 to 1200 ppmv and decreasing pH from 8.29 to 7.55 (−0.51 µm, a relative variation of 6.4 %, r²=0.67, p<0.01) (Fig. 3). The coccosphere size of the small species E. huxleyi exhibits a +0.22 µm increase with increasing pCO₂ and decreasing pH until 600 ppmv and 7.89 pH units (a relative variation of 4.4 %, r²=0.77, p<0.001). At higher pCO₂ and lower pH conditions, the coccosphere size is constant (r²=0.04, p>0.05) (Fig. 3). These absolute values and trends in coccosphere size with changes in CO₂ level and pH are in line with previously reported coccosphere and cell sizes from previous culture studies (Hermoso, 2015; Hermoso et al., 2014, 2016a; Phelps et al., 2021; Rickaby et al., 2010).

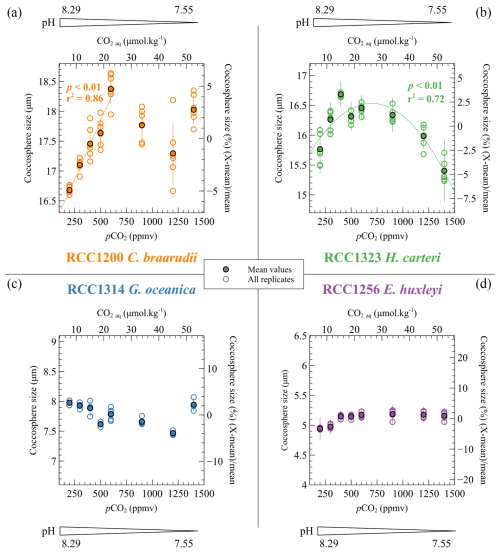
Figure 3 Evolution of the coccosphere sizes of the four cultured strains as a function of the CO₂ and pH culture conditions. The coccosphere sizes are given as the mean diameter of the coccospheres. They are given in micrometres (µm) (left axis) and as a percent relative to the mean coccosphere size of each strain (right axis). The empty points show the replicate data, and the filled points show the mean coccosphere sizes.

3.1.3 PIC : POC ratios

The PIC : POC ratio allows quantification of the respective allocation of carbon in its two fixation pathways (photosynthesis and calcification), which has isotopic implications for organic matter and coccoliths. The PIC : POC ratios of the four species do not show any statistically significant trend with changes in carbonate chemistry. Among the species cultured, C. braarudii has the highest PIC : POC ratio (mean value of 2.23 ± 0.49), followed by H. carteri (mean value of 1.44 ± 0.35), G. oceanica (0.98 ± 0.20) and E. huxleyi (0.50 ± 0.09) (Fig. 4). These data are consistent with previously published PIC : POC data (Krug et al., 2011; Langer et al., 2006; Müller et al., 2010; Rickaby et al., 2010; Riebesell et al., 2000).

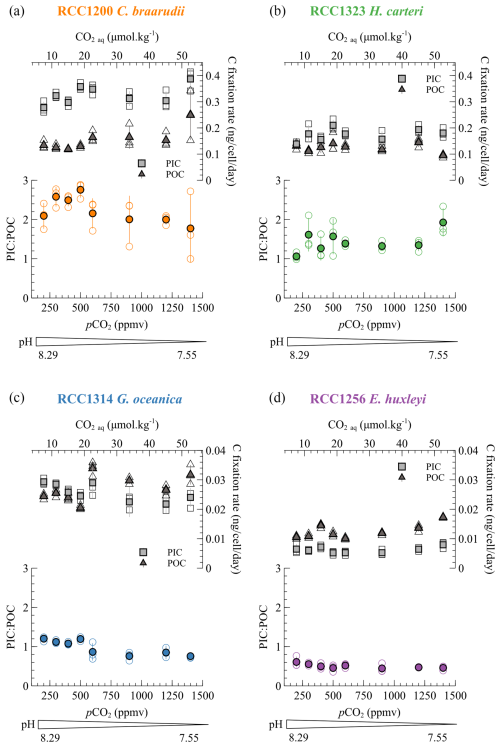
Figure 4 Particulate inorganic (square) and organic (triangle) carbon in nanograms (ng) per cell for each of the four species through the pCO₂ interval of the study (lower x axis) and the aqueous CO₂ (upper x axis). The PIC : POC ratios are also shown. The empty dots are all the replicate data, and the filled points are the mean values for each species/condition.

3.2 Carbon isotope ratios of coccolith calcite

The species E. huxleyi does not show any significant trend in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ with changes in pCO₂ and pH (r²=0.16, p>0.05). The taxon H. carteri exhibits a minor increase of less than 0.5 ‰ in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ with increasing CO₂ level et decreasing pH (r²=0.49, p<0.001) (Fig. 5). A key feature of the dataset pertains to the biogeochemical response of C braarudii. The distribution of the isotope data with changes in carbonate chemistry can be divided into two distinct trends for C. braarudii and G. oceanica. The first trend corresponds to low CO₂ level from 200 to 500 ppmv and high pH from 8.29 to 7.96. The second stage corresponds to high CO₂ level from 600 to 1400 ppmv and low pH from 7.89 to 7.55 pH units. At low CO₂ levels and high pH, the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of C. braarudii increases with increasing pCO₂ and decreasing pH (+2.41 ‰ V-PDB, a relative variation of 14 %, r²=0.83, p<0.001). Regarding G. oceanica, it exhibits a small $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ increase with increasing pCO₂ and decreasing pH (+0.41 ‰ V-PDB, a relative variation of 3 %, r²=0.61, p<0.01). Compared to low pCO₂ levels and high pH, the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of C. braarudii increases with a less steep slope at high CO₂ levels and low pH (+0.92 ‰ V-PDB, a relative variation of 7 %, r²=0.90, p<0.001). The $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of G. oceanica is steady at high pCO₂ and low pH (r²=0.10, p>0.05).

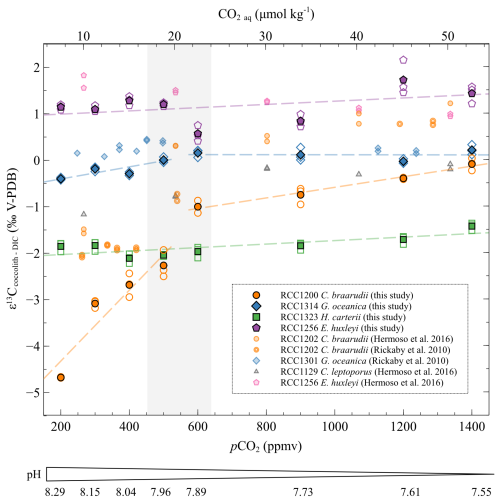
Figure 5 Evolution of the carbon isotopic ratios of the four studied strains with increasing CO₂ concentration and decreasing pH. The data are represented as the δ¹³C fractionation between coccoliths and DIC. The empty points are all the replicate data, and the filled points are the means (circles: Coccolithus braarudii, RCC 1200; diamonds: Gephyrocapsa oceanica, RCC 1314; squares: Helicosphaera carteri; pentagons: Emiliania huxleyi). The grey band represents the carbonate chemistry condition where a shift in the carbon and oxygen isotope ratio occurs (between 500 and 600 ppmv). The pH indicated below only refers to the pH of the cultures of this study. The sources of previously reported data (smaller symbols) are shown in the inset.

These δ¹³C data do not exhibit any consistent trend with changes in growth rate, nor with PIC and POC. G. oceanica and E. huxleyi show a carbon vital effect similar to that obtained in the work of Rickaby et al. (2010) and Hermoso et al. (2016a). It is also similar to the dataset published in McClelland et al. (2017). The vital effects of C. braarudii in this study have a similar evolution to previously published results (Hermoso et al., 2016a; Rickaby et al., 2010), with a 1 ‰ shift towards more negative values (Fig. 5). The δ¹³C ratios of C. braarudii are relatively similar to the results of Chauhan and Rickaby (2024), albeit with slightly lower absolute values in this latter work. The δ¹³C values observed by Chauhan and Rickaby (2024) for G. oceanica and for E. huxleyi are very negative for CO_2 aq concentrations greater than 20 µmol kg⁻¹ ($\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}-\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{DIC}}$ values between −2.5 ‰ and −8 ‰ V-PDB), which contrasts with previous studies published in the literature (Rickaby et al., 2010; Hermoso et al., 2016; McClelland et al., 2017).

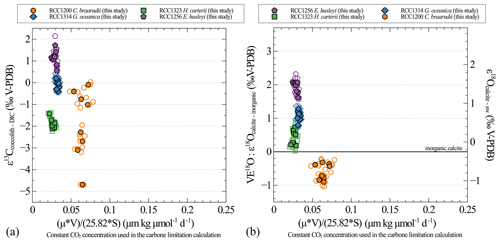
One of the results of our study is that growth rates remain unchanged despite the imposed changes in CO₂ concentration and pH for all strains and that cell size remains unchanged despite imposed changes in CO₂ concentration and pH for the small strains (namely G. oceanica and E. huxleyi). It thus turns out that the CO₂ concentration is the only parameter that dictates the carbon limitation here (Figs. A3 and A4). This is why we decided not to represent the isotopic data as a function of the carbon limitation (Bidigare et al., 1997) but only as a function of CO₂ level (Figs. A3 and A4).

3.3 Oxygen isotope ratios of coccolith calcite

The $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ of G. oceanica is constant regardless of ambient conditions (r²=0.02, p>0.05), as for $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ values of E. huxleyi (r²=0.15, p>0.05). The mean magnitude of the oxygen vital effects of G. oceanica and E. huxleyi is positive (+1.05 ‰ and +1.92 ‰, respectively), in good agreement with previously published data (Dudley et al., 1986; Hermoso et al., 2016a, b; Rickaby et al., 2010; Stevenson et al., 2014; Ziveri et al., 2003).

As already shown in the literature, H. carteri has a $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ close to the inorganic reference (mean $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ of +0.42 ‰) (Ziveri et al., 2003). Indeed, at low CO₂ concentrations and high pH, the $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ of H. carteri is slowly decreasing (−0.22 ‰, a relative variation of 4 %, r²=0.57, p<0.01). From 600 ppmv and 7.89 pH units, the $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ of H. carteri is stable (r²≈0, p>0.05) (Fig. 6).

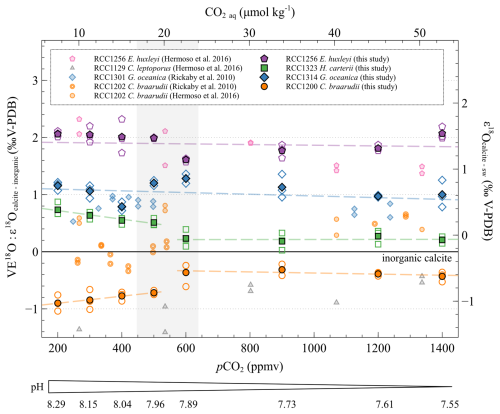
Figure 6 Evolution of the oxygen isotopic ratios of the four studied strains with increasing CO₂ concentration and decreasing pH. The data are represented as the vital effect (left axis) and as the isotopic fractionation between the seawater and the coccoliths δ¹⁸O (right axis). The empty points are all the replicate data, and the filled points are the means (circles: Coccolithus braarudii, RCC 1200; diamonds: Gephyrocapsa oceanica, RCC 1314; squares: Helicosphaera carteri; pentagons: Emiliania huxleyi). The vertical grey band represents the carbonate chemistry condition where a shift in the carbon and oxygen isotope ratio occurs. The black line shows the calculated isotope ratio of an inorganic calcite (see “Materials and methods”). The pH scale indicated below only refers to the data published in this study. The sources of previously reported data (smaller symbols) are shown in the inset.

The $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ of C. braarudii is constant at low pCO₂ and high pH with a mean value of −0.81 ‰ ± 0.13 ‰ V-PDB and is also constant at high pCO₂ and low pH with a mean value of −0.37 ‰ ± 0.12 ‰ V-PDB. A significant difference in the C. braarudii VE¹⁸O is registered between high and low CO₂ ambient conditions in the culture medium. This +0.5 ‰ shift occurs between 500 and 600 ppmv. While we present evidence of a negative vital effect of coccoliths for C. braarudii, previous studies have shown a $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ close to that of the inorganic for this species. (Hermoso, 2015; Hermoso et al., 2016b; Rickaby et al., 2010; Stevenson et al., 2014).

3.4 Carbon isotope ratios of organic matter

The carbon isotopic ratio of organic matter of C. braarudii is decreasing with increasing CO₂ level (from 200 to 500 ppmv) and decreasing pH (from 8.29 to 7.89 pH units) (r²=0.93, p<0.001). This result is mirrored by a large $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ increase (+2.4 ‰ V-PDB) (Fig. 5). Above 600 ppmv (below 7.89 pH units), $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ values of C. braarudii are stable and close to the values observed for the condition of 200 ppmv and 8.29 pH units (−30.77 ‰ ± 0.36 ‰ V-PDB) (Fig. 7). $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ values of G. oceanica do not change with changes in pCO₂ and pH (mean of −29.12 ‰ ± 0.41 ‰ V-PDB), similar to what was described for the changes in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$.

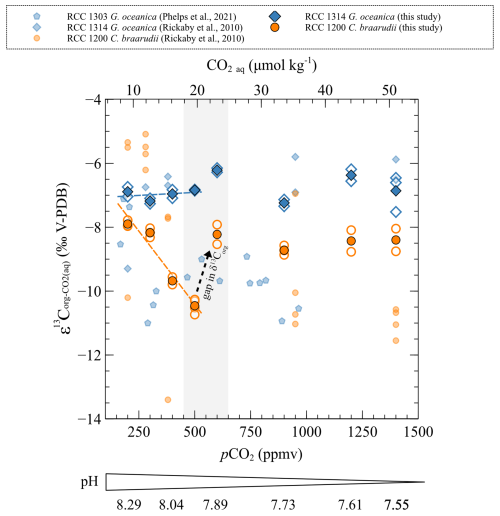
Figure 7 Carbon isotopic ratios of organic matter compared to $\mathit{\delta }{}^{\mathrm{13}}{\mathrm{C}}_{{\mathrm{CO}}_{\mathrm{2}},\text{aq}}$ of the strains studied with changes in CO₂ level and pH. The empty points are all the replicate data, and the filled points are the means. The grey band represents the carbonate chemistry condition where a shift in the carbon and oxygen isotope ratio occurs. The sources of previously reported data (smaller symbols) are shown in the inset.

4 Discussion

In this study, we chose to perturb only the carbonate chemistry of the culture medium in which the cells grew. It is important to remember that other environmental factors, such as light irradiance and temperature, also influence cellular growth and the magnitude of vital effects (Langer et al., 2009; Sett et al., 2014; Hermoso et al., 2016; Gafar et al., 2018).

The aim of our study is to quantify the environmental forcing exerted by CO₂ availability and ambient pH on the expression of vital effects of the coccolithophores with a view to develop and further palaeoenvironmental proxies. Our experimental results shed light on the link between the environment, cellular growth and the efficiency of carbon fixation, as these parameters collectively control the expression of biologically induced fractionation in organic and inorganic calcite. The published literature has revealed the role of carbon acquisition throughout the cell membrane and intracellular utilisation of carbon in the magnitude of the vital effect through laboratory and modelling studies (Laws et al., 1995; McClelland et al., 2017; Popp et al., 1998; Rau et al., 1996). Bolton and Stoll (2013) coined the concept of the demand-to-supply ratio to characterise the isotopic implications of carbon trafficking within the cells, with large implications for the carbon isotope vital effect at different timescales. Based on these studies, it appears that the carbon isotopic composition of coccolith calcite was primarily controlled by the interplay between inorganic carbon fixation in the coccolith vesicles and organic carbon fixation in the chloroplast. At a constant source of external carbon, the main driver dictating the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ is the amount and isotopic composition of organic matter produced through photosynthetic carbon fixation (POC per cell and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$, respectively) (McClelland et al., 2017).

As the foremost finding of our study, we show that C. braarudii exhibits a large $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ increase (+2.4 ‰ V-PDB) mirrored by a $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ decrease (−2.6 ‰ V-PDB) from 200 ppmv and 8.29 pH units to 500 ppmv and 7.96 pH units (low pCO₂ and high pH conditions) (Fig. 8). The correlation between $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ of C. braarudii is not seen at high pCO₂ and low pH (600 to 1400 ppmv/7.89 to 7.55 pH units) (Figs. 5 and 7). Based on this observation, we will treat the data obtained at low pCO₂–high pH separately from those obtained at high pCO₂–low pH in the following sections. It is also noteworthy that the small species G. oceanica and E. huxleyi do not show statistically significant changes in the magnitude of ¹³C fractionation, neither in coccolithophores calcite nor in the organic matter with changes in CO₂ level and pH (at neither low pCO₂ and high pH, nor over the whole interval). The invariant nature of inorganic and bulk organic isotopic composition with our CO₂ and pH treatment is an important result for research dealing with alkenone carbon isotope fractionation and requires closer examination by molecular geochemists.

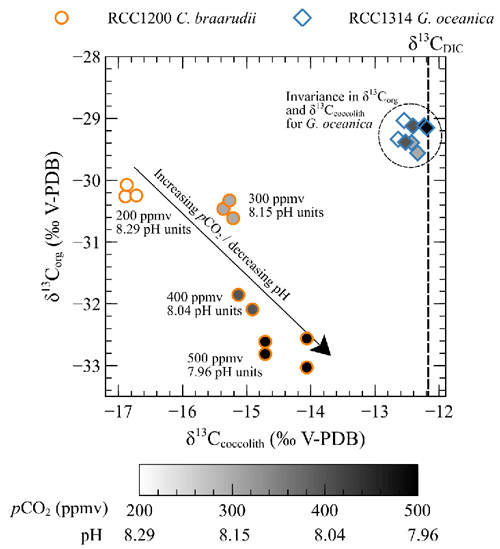
Figure 8 Scatter plot showing δ¹³C_org versus δ¹³C_coccolith values with changes in pCO₂ and pH (circles: Coccolithus braarudii, RCC 1200; diamonds: Gephyrocapsa oceanica, RCC 1314). Grey levels correspond to growth conditions (white: 200 ppmv–8.29 pH units, light grey: 300 ppmv–8.15 pH units, dark grey: 400 ppmv–8.04 pH units, black: 500 ppmv–7.96 pH units).

4.1 The biogeochemical causes for the changes in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of C. braarudii at low pCO₂ and high pH

Given that organic compounds are significantly ¹²C-enriched relative to CO₂ with typical $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ values around −25 ‰, the more organic matter produced, the isotopically heavier the residual intracellular carbon pool. As calcification derives from this latter pool, coccoliths produced by highly photosynthetic coccolithophore cells exhibit relatively higher δ¹³C values. This phenomenon is particularly expressed in small cells such as E. huxleyi and G. oceanica that are characterised by high POC contents and the highest δ¹³C values (Ziveri et al., 2003) (Figs. 4 and 5). We can hypothesise that a change in the efficiency of POC production with changes in carbon availability can induce a change in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ values, as it is apparent for C. braarudii with a +2.4 ‰ V-PDB shift between low and high pCO₂ conditions (Fig. 5). There is no apparent change in POC, PIC / POC or growth rates with the increase in δ¹³C at low pCO₂ and high pH (Figs. 2 and 4), despite an increase in chlorophyll a concentration within the cells between 200 and 500 ppmv (Fig. A2). These observations exclude a control of the amount of organic matter produced on the isotopic signature of the internal pool. Furthermore, a change in the efficiency of POC production could not explain the coeval −2.6 ‰ and progressive decrease in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ values within this low pCO₂ interval. Thus, another biogeochemical process has to be sought.

A second means to explain coeval changes in both $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ can rely on a change in the isotopic composition of the carbon acquired by the cell (CO₂ vs. ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$). Carbon-concentrating mechanisms (Giordano et al., 2005) can induce a shift in internal carbon pool to which a contribution by ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$ ions by active transport becomes significant (Bolton and Stoll, 2013). Under this circumstance, the internal carbon may exhibit higher δ¹³C values, as there is a typical 9 ‰ equilibrium fractionation between CO₂ and ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$ (Zeebe and Wolf-Gladrow, 2001). However, early biological work aiming at characterising inducible CCMs in phytoplankton has revealed the lack of such active strategies of carbon acquisition in the large and ancestral Coccolithus taxon (Nimer et al., 1999). Furthermore, the build-up of the carbon pool with a proportion of ${{\mathrm{HCO}}_{\mathrm{3}}}^{-}$ would have led to higher δ¹³C values in both organic and inorganic pool, which is not what is observed at the lowest pCO₂ (conversely, the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ values are lower) (Figs. 5 and 7). These two lines of evidence rule out the CCM hypothesis.

Culture and wild coccolithophore data have revealed that the carbon isotope composition of the organic matter decreases with increasing CO₂ concentration (Bidigare et al., 1997). The magnitude of carbon isotope fractionation between the CO₂ substrate and the organic matter is thus not constant when the concentration/availability of ambient CO₂ changes, forming the ground of the ε_p-alk proxy (Pagani, 2002; Popp et al., 1998). This modulation of the carbon kinetic fractionation by RuBisCO not only has consequences on the isotopic signature of the organic matter of which the compound-specific as alkenones but also has consequences on the residual carbon pool and ultimately on coccolith calcite.

This biogeochemical control is compatible with the 2.6 ‰ decrease observed in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ of C. braarudii between 200 and 500 ppmv (r²=0.93, p<0.001) (Fig. 7). As the inorganic carbon pool and organic carbon pool are linked at least from an isotopic perspective, we can interpret the +2.4 ‰ change in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ as the result of a modulation of kinetic fractionation by the enhanced fixation of ¹²C in organic matter by the enzyme RuBisCO – itself caused by the alleviated CO₂ limitation (Fig. 8).

As we do not observe any change in the growth rate nor in the POC per cell between low and high pCO₂ conditions, we suggest that the cells compensate for the decrease in carbon bioavailability in a different way. Indeed, the lower surface-to-volume ratio at 200 ppmv compared to 500 ppmv (cell diameter of 16.7 ± 0.2 µm at 200 ppmv and 17.6 ± 0.4 µm at 500 ppmv, in Fig. 3) may compensate for the lower supply with a lower demand to sustain growth rate (Bolton and Stoll, 2013; Rau et al., 1996). This point will be further discussed in Sect. 4.2 for the high CO₂ level and low pH conditions.

4.2 Change in the isotope biogeochemistry of coccolithophore cells in response to alleviated carbon limitation and enhanced proton concentration in the cell (Coccolithus braarudii versus smaller species)

The data for C. braarudii, and in particular the link between CO₂ availability and the isotopic composition of coccoliths and organic matter, cannot be explained by a uniform biogeochemical framework, as a gap is seen for C. braarudii between 500 and 600 ppmv (Figs. 5 and 7). The data show that above 600 ppmv, there is no correlation between ambient CO₂ levels and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$. Furthermore, there is no covariation between $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ values of C. braarudii at high pCO₂ and low pH, in contrast to the covariation observed at low pCO₂ and high pH. The latter point suggests that the organic-to-inorganic forcing that occurs at low pCO₂ levels no longer operates at high pCO₂ levels. The fact that there is no organic-to-inorganic forcing at high pCO₂ is presumably due to the alleviation of carbon limitation or at least due to a lower carbon demand-to-supply ratio (Bolton et al., 2012). Likewise, coccosphere sizes exhibit no discernible trend with CO₂ concentrations at high CO₂ levels (Fig. 3), in contrast to the findings at low CO₂ levels, which were tentatively attributed to a way to enhance CO₂ influx to the cells. This biogeochemical feature would indicate that the forcing of CO₂ availability on the apparent ${}^{\mathrm{13}}\mathrm{C}/{}^{\mathrm{12}}\mathrm{C}$ fractionation between the organic matter and calcite occurs only below 600 ppmv. Meanwhile, small species (E. huxleyi and G. oceanica) show unchanged isotopic values with the pCO₂/pH treatments. As explained in Rickaby et al. (2010) and Hermoso et al. (2016), the high surface-to-volume ratio of the small cells induces no carbon limitation at low CO₂ level and hence no impact of the change of pCO₂ and pH on the carbon isotopic system.

Elevated pCO₂ conditions are accompanied by greater proton concentrations in the environment (more acidic conditions). Calcifying phytoplankton such as the coccolithophores has to efflux the excess protons generated by calcification by an active process operated by transmembrane Hv channels (Kottmeier et al., 2022; Taylor et al., 2011). Previous biological studies have demonstrated that the opening of Hv channels is affected by intracellular pH (Taylor et al., 2011). Hv channels are closed at low pH to counter the influx of protons into the cell from the ambient environment. The pH threshold for the closure of the Hv channels has been assessed to be within the range of 8.1–7.5 pH units (Kottmeier et al., 2022). Therefore, assuming that the threshold stands between 7.96 and 7.89 pH units, the closure of the Hv channels is a good candidate for the 500–600 ppmv gap apparent in our dataset (Figs. 5–7). The closure of the Hv channels can thus result in an accumulation of H⁺ ions within the cytosol, leading to an intense decline in intracellular pH (Kottmeier et al., 2022; Taylor et al., 2011). The release of protons by coccolithogenesis is even more influential on intracellular pH for species such as C. braarudii, which produces a large amount of PIC compared to smaller cells (Fig. 4). The latter cells (G. oceanica and E. huxleyi) indeed do not exhibit such a gap in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ between 500 and 600 ppmv (small mean PIC values of 0.025 ± 0.004 and 0.006 ± 0.001 ng per cell in the 600–1400 ppmv interval, respectively). Interestingly, the gap in C. braarudii $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ between low and high CO₂ levels is not seen in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$. This observation could be the consequence of a decrease in the organic–inorganic carbon pool coupling when carbon supply is high, unlike below 500 ppmv when the small carbon pool imposes a high isotopic dependence between organic matter and calcite through Rayleigh distillation, governed by the organic carbon fixation.

Around the same pCO₂ and pH limit as presented for $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of C. braarudii, we observe a change in $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ values. The mean VE¹⁸O value is −0.81 ‰ ± 0.13 ‰ V-PDB at low pCO₂–high pH conditions, while the mean VE¹⁸O value is −0.37 ‰ ± 0.12 ‰ V-PDB at high pCO₂–low pH conditions (Fig. 6). The gap in $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ can be explained by the change in pH. When the pH in the environment is low, it has been demonstrated that the ${}^{\mathrm{18}}\mathrm{O}/{}^{\mathrm{16}}\mathrm{O}$ equilibration time between DIC species and H₂O is shorter compared to high pH conditions (Usdowski et al., 1991). The isotopic equilibration between $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{DIC}}$ and $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{sw}}$ due to the assimilation of isotopically heavy CO₂ into the cell is consequently more complete at low pH than at high pH. As a consequence, $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{DIC}}$ (and consequently $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$) values are closer to $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{sw}}$ at high pCO₂ and low pH (Fig. 6). The $\mathit{\delta }{{}^{\mathrm{18}}\mathrm{O}}_{\text{coccolith}}$ difference between high and low pH levels is consistent with a change in pH homeostasis strategy between 7.96 and 7.89 pH units that can be responsible for the δ¹³C before and after 500/600 ppmv. It has to be noted that coccolithophores possess internal carbonic anhydrase (CA) (Paneth and O'Leary, 1985). CA could also accelerate the sluggish H₂O–DIC equilibration isotopic time for the oxygen system, but the kinetics of isotopic re-equilibration of the internal carbon pool within the cell compartments is unknown.

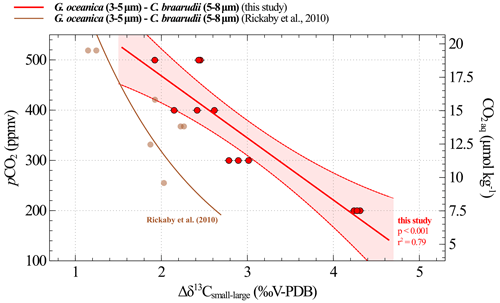
Figure 9 Calibration between CO₂ and $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ obtained from culture experiments. The results of our study are shown in red (replicate data as dots; the linear regression made between 200 and 500 ppmv corresponds to the solid line, and the confidence interval is shown with dotted lines). The brown curve is the equation recalculated from the Rickaby et al. (2010) dataset. The relation encompassing the 200–1400 ppmv spectrum is shown in Fig. A1.

4.3 Palaeoclimatic implications of the CO₂ proxy based on $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$

4.3.1 $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ evolution with changes in CO₂ levels and pH

The increase in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of C. braarudii with rising pCO₂ and decreasing pH shows potential as a proxy for reconstructing past carbonate chemistry and consequently atmospheric pCO₂. However, utilising absolute carbon vital effects of coccoliths for palaeo-pCO₂ reconstructions requires accurate knowledge of the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{inorganic}}$ (DIC) of past oceans, which remains challenging (Hermoso et al., 2020). To overcome this issue, previous studies (Bolton et al., 2012; Bolton and Stoll, 2013; Godbillot et al., 2022; Hermoso et al., 2016a; McClelland et al., 2017; Tremblin et al., 2016) have proposed the use of the offset between the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of small and large species, known as the differential vital effect (noted Δδ¹³C, Eq. 3). Indeed, the $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ of the small cells (Noelaerhabdaceae) is steady, regardless of the pCO₂ levels, due to their relatively large carbon pool, akin to C. braarudii under carbon replete conditions (at high pCO₂ and low pH). The coccolith-based palaeo-pCO₂ proxy is independent of the isotope ratio of ambient DIC, as both small and large coccoliths are produced by cells growing in the same shallow water. In practical terms, $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ can be expressed as follows:

$$\begin{array}{}\text{(3)}& \mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}=\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small coccolith}}-\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{large coccolith}},\end{array}$$

where δ¹³C is expressed in ‰V-PDB. The small coccoliths used in this work are those of G. oceanica, and the large coccoliths are those of C. braarudii.

Δδ¹³C and pCO₂ data highlight a linear relationship across the range of 200 to 500 ppmv (i.e. pH values between 8.29 and 7.96), which we previously explained by a modulation in the carbon isotope fractionation between CO_2 aq and the organic matter (Fig. 9, Eq. 4). The uncertainties associated with the constants in Eq. (4) represent the standard deviation obtained with a linear regression model.

$$\begin{array}{}\text{(4)}& \begin{array}{rl}{\mathrm{CO}}_{\text{2\hspace{0.17em}aq}}& (\pm \mathrm{3.17})=\\ & -\mathrm{4.65}(\pm \mathrm{0.72})\times \mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\mathit{\text{G.\hspace{0.17em}oceanica\hspace{0.17em}-\hspace{0.17em}C.\hspace{0.17em}braarudii}}}\\ & +\mathrm{26.90}(\pm \mathrm{2.21}),\end{array}\end{array}$$

where CO_2 aq is expressed in µmol kg⁻¹, and Δδ¹³C is expressed in ‰V-PDB.

A comparison of our calibration with the data published by Rickaby et al. (2010) in Fig. 9 reveals a discrepancy of up to 1.5 ‰ under the lowest pCO₂–highest pH conditions. Explaining this difference is challenging, particularly because the studies were conducted under different conditions, involved different strains and included only a few data points in this low CO₂ range, as documented by Rickaby et al. (2010). This observation may call into question the feasibility of achieving a species- and environment-integrated response in coccolithophores (see further discussion in Sect. 4.3.2).

4.3.2 Palaeoclimate application of carbon isotope culture data

In this study, we further develop a geological CO₂-sensitive probe by the use of the $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ isotopic offset. This probe forms the basis for a palaeo-CO₂ proxy transferable to sedimentary records from geological periods with low CO₂ levels, such as the Neogene and the Quaternary (Bolton and Stoll, 2013; Godbillot et al., 2022; Hermoso et al., 2020). Providing a new palaeo-pCO₂ proxy for those periods is of key interest, as it has been shown that alkenones are less sensitive to low and medium CO₂ level changes (Badger et al., 2019). Another strength of the pCO₂ proxy presented here is that it is based on coccolith calcite, which can be separated from other sedimentary components according to their size ranges by microfiltering and centrifuging protocols (Minoletti et al., 2008; Stoll and Ziveri, 2002; Zhang et al., 2018, 2021).

In addition, our study provides a biogeochemical explanation for $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ changes with CO₂ level and pH, thus supporting the reliability of these equations for palaeoclimate applications. We also demonstrate that the $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$-CO₂ calibration can be extended to the whole interval studied, i.e. above 600 ppmv (Fig. A1). Despite the fact that the link between $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$ and CO₂ levels is less constrained and understood at high CO₂ level than at low CO₂ levels, the relationship is statistically supported on the whole interval (r²=0.80, p<0.001) (Fig. A1). Thus, this calibration can potentially be used to reconstruct palaeo-CO₂ levels throughout the entire Cenozoic era.

It has to be mentioned that using C. braarudii as a culture model for large cells with a palaeoclimatic perspective is supported by the fact that C. pelagicus coccoliths were present in all sediments from the Equator to the mid latitudes (50° N) in the Atlantic and Pacific oceans before the Pliocene–Pleistocene boundary (Sato et al., 2004). Nowadays, C. braarudii is not a species thriving at low latitudes. One promising research avenue would be the study of the co-variation of CO₂ and pH levels crossed with temperature changes in new culture campaigns. This approach could have the potential to reveal the synergistic effect of discrete various controls on cell growth rates and refine the biogeochemical understanding of the vital effects.

5 Conclusion

The combined study of the impact of CO₂ concentration and pH on the fractionation of carbon and oxygen isotopes in coccolithophores (in their organic matter and calcite biominerals) provides an explanation of the cause of variations in $\mathrm{\Delta }\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{small–large}}$. One of the major findings of this study is the coeval variation of $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ in Coccolithus braarudii with changes in pCO₂ between 200 and 500 ppmv and pH between 8.29 and 7.96. Combined with the fact that physiological parameters (growth rates, PIC and POC) of C. braarudii remain unchanged despite changes in the availability of carbon, these results indicate that the cause of variations in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ is an environmental-driven change in the magnitude of the fractionation between ambient CO_2 aq and organic matter produced by C. braarudii. Above 500 ppmv, and for pH values below 7.96, greater carbon availability induces isotopic decoupling between $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ and $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$. On the other hand, small species exhibit no change in $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ or $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{org}}$ in response to changes in CO₂ levels and pH. By comparing $\mathit{\delta }{{}^{\mathrm{13}}\mathrm{C}}_{\text{coccolith}}$ values of small (G. oceanica or E. huxleyi) with those of large cells (C. braarudii), we have established an insightful Δδ¹³C–CO₂ transfer equation relevant for the Neogene and Quaternary time slices. The extent to which our biogeochemical calibration can be applied to wild and fossil coccoliths depends on the (palaeo-)environmental context. In these experiments, only the biogeochemical responses of monoclonal strains have been examined, while variations in light irradiance and temperature may interact with changes in the carbonate chemistry of water masses. In the future, a broader range of strains and additional physico-chemical parameters must be studied, integrating these factors to develop a unified response through modelling.

Appendix A

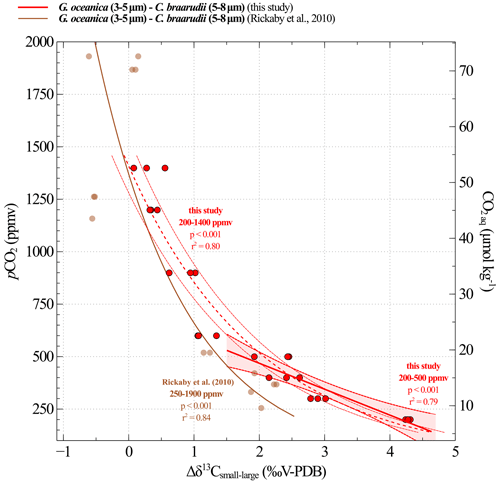
Figure A1 Calibration between CO₂ concentrations and Δδ¹³C_{small–large} obtained from culture experiments at low and high CO₂ levels. The results of our study are shown in red. Replicate data are illustrated with red dots. The linear regression made between 200 and 500 ppmv corresponds to the solid line and the confidence interval is shown with dotted lines. The dotted line shows the logarithmic regression made for the data on the whole interval (200 to 500 ppmv). The brown curve is the equation recalculated from Rickaby et al. (2010) dataset.

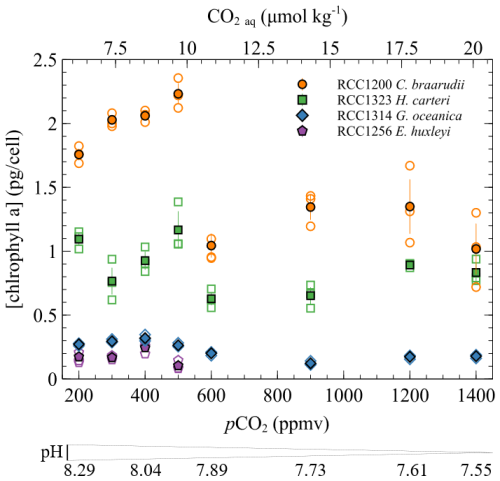
Figure A2 Chlorophyll a concentration (pg per cell) as a function of CO₂ and pH. The empty points are all the replicate data, and the filled points are the means (circles: Coccolithus braarudii, RCC 1200; diamonds: Gephyrocapsa oceanica, RCC 1314; squares: Helicosphaera carteri; pentagons: Emiliania huxleyi). Measurements of chlorophyll a concentration were conducted using fluorometry according to the SOMLIT national protocol (Yentsch and Menzel, 1963). A Turner Designs Trilogy fluorometer was employed to measure the fluorescence of our samples before and after acidification. The samples were acidified with 10 µL of hydrochloric acid per millilitre (mL) of acetone extract and left in the dark for 2 min between the two measurements. The equation used to calculate the chlorophyll concentration is taken from Lorenzen (1967).

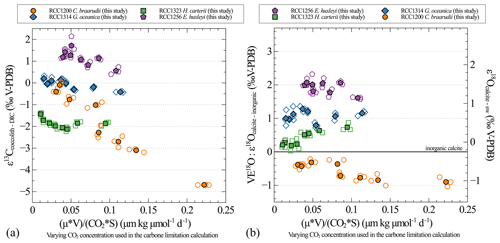
Figure A3 Carbon (a) and oxygen (b) isotopic effect with changes in the carbon limitation. Carbon limitation is calculated as the product of growth rate (μ; in d⁻¹) and coccosphere volume (V; in µm³) divided by the product of CO₂ concentration (CO₂; in µmol kg⁻¹) and coccosphere surface (S; in µm²), according to Bidigare et al. (1997).

Figure A4 Carbon (a) and oxygen (b) isotopic effect with changes in the carbon limitation calculated as for Fig. A3 with the difference that the CO₂ concentration is a constant (25.82 µmol kg⁻¹, mean CO₂ concentration of the medium conditions in this study).