Stable isotopic evidence for the excess leaching of unprocessed atmospheric nitrate from forested catchments under high nitrogen saturation

Owing to the elevated loading of nitrogen through atmospheric deposition, some forested ecosystems become nitrogen saturated, from which elevated levels of nitrate are exported. The average concentration of stream nitrate eluted from upstream and downstream of the Kasuya Research forested catchments (FK1 and FK2 catchments) in Japan were more than 90 µM, implying that these forested catchments were under nitrogen saturation. To verify that these forested catchments were under the nitrogen saturation, we determined the export flux of unprocessed atmospheric nitrate relative to the entire deposition flux ($M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio) in these catchments; because the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio has recently been proposed as a reliable index to evaluate nitrogen saturation in forested catchments. Specifically, we determined the temporal variation in the concentrations and stable isotopic compositions, including Δ¹⁷O, of stream nitrate in the FK catchments for more than 2 years. In addition, for comparison, the same parameters were also monitored in the Shiiba Research forested catchment (MY catchment) in Japan during the same period, where the average stream nitrate concentration was low, less than 10 µM. While showing the average nitrate concentrations of 109.5, 90.9, and 7.3 µM in FK1, FK2, and MY, respectively, the catchments showed average Δ¹⁷O values of +2.6 ‰, +1.5 ‰, and +0.6 ‰ in FK1, FK2, and MY, respectively. Thus, the average concentration of unprocessed atmospheric nitrate ([NO${}_{\mathrm{3}}^{-}$_atm]) was estimated to be 10.8, 5.1, and 0.2 µM in FK1, FK2, and MY, respectively, and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio was estimated to be 14.1 %, 6.6 %, and 1.3 % in FK1, FK2, and MY, respectively. The estimated $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in FK1 (14.1 %) was the highest ever reported from temperate forested catchments monitored for more than 1 year. Thus, we concluded that nitrogen saturation was responsible for the enrichment of stream nitrate in the FK catchments, together with the elevated NO${}_{\mathrm{3}}^{-}$_atm leaching from the catchments. While the stream nitrate concentration ([NO${}_{\mathrm{3}}^{-}$]) can be affected by the amount of precipitation, the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio is independent of the amount of precipitation; thus, the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio can be used as a robust index for evaluating nitrogen saturation in forested catchments.

1 Introduction

Nitrate is important as a nitrogenous nutrient in the biosphere. Traditionally, forested ecosystems have been considered as nitrogen limited (Vitousek and Howarth, 1991). However, owing to the elevated loading of nitrogen through atmospheric deposition, some forested ecosystems become nitrogen saturated (Aber et al., 1989), from which elevated levels of nitrate are exported (Mitchell et al., 1997; Peterjohn et al., 1996). Such excessive leaching of nitrate from forested catchments degrades water quality and causes eutrophication in downstream areas (Galloway et al., 2003; Paerl and Huisman, 2009). Thus, evaluating the stage of nitrogen saturation in each forested catchment, including its temporal variation, is critical for sustainable forest management, especially for forested ecosystems under high nitrogen deposition.

Both concentration and seasonal variation of stream nitrate have been used as indexes to evaluate the nitrogen saturation of each forested catchment in past studies (Aber, 1992; Rose et al., 2015; Stoddard, 1994). A forested stream eluted from Fernow Experimental Forest USA, for instance, showed an elevated average nitrate concentration of 60 µM, along with the absence of a seasonal variation in the stream nitrate concentration, so the forest was classified into stage 3, the highest stage of nitrogen saturation (Rose et al., 2015).

However, using both the concentration level (high or low) and seasonal variation (clear or absent) of stream nitrate as indexes to evaluate nitrogen saturation has limitations, including the following: (1) seasonal variation of soil nitrate can be buffered by groundwater with long residence time, so that the seasonal variation is unclear in stream nitrate concentration in Japan, even in normal forests under the nitrogen saturation stage of 0 (Mitchell et al., 1997); and (2) the stream nitrate concentration can be enriched or diluted depending on the volume of rainfall, so the concentration level can be high in low precipitation area irrespective of the stage of nitrogen saturation.

Nakagawa et al. (2018) lately proposed that the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio, the export flux of unprocessed atmospheric nitrate (M_atm) relative to the deposition flux of NO${}_{\mathrm{3}}^{-}$_atm (D_atm), can be an alternative, more robust index for evaluating nitrogen saturation in each forested catchment, because the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio directly reflects the demand for atmospheric nitrate deposited onto each forested catchment as a whole, and thus reflects the nitrogen saturation in each forested catchment. That is, we can expect high $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratios in forested catchments under nitrogen saturation and low $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratios in forested catchments with nitrogen deficiency.

To estimate the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio accurately and precisely in each forested catchment, the fraction of unprocessed atmospheric nitrate (NO${}_{\mathrm{3}}^{-}$_atm) in the stream needs to be estimated accurately and precisely. Triple oxygen isotopic compositions of nitrate (Δ¹⁷O) have recently been used as a conservative tracer of NO${}_{\mathrm{3}}^{-}$_atm deposited onto each forested catchment (Inoue et al., 2021; Michalski et al., 2004; Nakagawa et al., 2018; Tsunogai et al., 2014; Ding et al., 2022), showing distinctively different Δ¹⁷O from that of remineralized nitrate (NO${}_{\mathrm{3}}^{-}$_re) derived from organic nitrogen through general chemical reactions, including microbial N mineralization and microbial nitrification. While NO${}_{\mathrm{3}}^{-}$_re, the oxygen atoms of which are derived from either terrestrial O₂ or H₂O through microbial processing (i.e., nitrification), always shows the relation close to the “mass-dependent” relative relation between ${}^{\mathrm{17}}\mathrm{O}{/}^{\mathrm{16}}\mathrm{O}$ ratios and ${}^{\mathrm{18}}\mathrm{O}{/}^{\mathrm{16}}\mathrm{O}$ ratios, NO${}_{\mathrm{3}}^{-}$_atm displays an anomalous enrichment in ¹⁷O reflecting oxygen atom transfers from atmospheric ozone (O₃) during the conversion of NO_x to NO${}_{\mathrm{3}}^{-}$_atm (Alexander et al., 2009; Michalski et al., 2003; Morin et al., 2011; Nelson et al., 2018). As a result, the Δ¹⁷O signature defined by the following equation (Kaiser et al., 2007) enables us to distinguish NO${}_{\mathrm{3}}^{-}$_atm (Δ¹⁷O > 0) from NO${}_{\mathrm{3}}^{-}$_re (Δ¹⁷O = 0):

$$\begin{array}{}\text{(1)}& {\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}={\displaystyle \frac{\mathrm{1}+{\mathit{\delta }}^{\mathrm{17}}\mathrm{O}}{(\mathrm{1}+{\mathit{\delta }}^{\mathrm{18}}\mathrm{O}{)}^{\mathit{\beta }}}}-\mathrm{1},\end{array}$$

where the constant β is 0.5279 (Kaiser et al., 2007), δ¹⁸O = $R_{\mathrm{sample}}/R_{\mathrm{standard}}-\mathrm{1}$, and R is the ${}^{\mathrm{18}}\mathrm{O}{/}^{\mathrm{16}}\mathrm{O}$ ratio (or the ${}^{\mathrm{17}}\mathrm{O}{/}^{\mathrm{16}}\mathrm{O}$ ratio in the case of δ¹⁷O or the ${}^{\mathrm{15}}\mathrm{N}{/}^{\mathrm{14}}\mathrm{N}$ ratio in the case of δ¹⁵N) of the sample and each standard reference material. In addition, Δ¹⁷O is almost stable during mass-dependent isotope fractionation processes within terrestrial ecosystems. Therefore, while the δ¹⁵N or δ¹⁸O signature of NO${}_{\mathrm{3}}^{-}$_atm can be overprinted by the biological processes subsequent to deposition, Δ¹⁷O can be used as a robust tracer of unprocessed NO${}_{\mathrm{3}}^{-}$_atm to reflect its accurate mole fraction within total NO${}_{\mathrm{3}}^{-}$, regardless of the progress of the partial metabolism (partial removal of nitrate through denitrification and assimilation) subsequent to deposition (Michalski et al., 2004; Nakagawa et al., 2013, 2018; Tsunogai et al., 2011, 2014, 2018).

Past studies reported that the maximum concentration of stream nitrate was 58.4 µM in the KJ forested catchment in Japan, with the maximum value of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio as 9.4 % (Nakagawa et al., 2018; Sase et al., 2022). Whether the index of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio can be applied to forested catchments, where the leaching of stream nitrate is much higher than the KJ forested catchment, remained unclarified. Besides, the advantages of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio within the past indexes of nitrogen saturation have not been discussed.

Chiwa (2021) has recently reported the enrichment of nitrate of more than 90 µM on the annual average in forested streams eluted from the catchments (FK1 and FK2) in the Kasuya Research Forest, Kyushu University, Japan (Fig. 1a and b). The observed enrichment of stream nitrate implied that these forested catchments were under nitrogen saturation. Thus, in this study, we determined the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in the FK1 and FK2 forested catchments by monitoring both the concentration and Δ¹⁷O of stream nitrate for more than 2 years to verify that these forested catchments were under nitrogen saturation. For comparison, the catchment (MY catchment) in Shiiba Research Forest, Kyushu University, Japan (Fig. 1a and c), was also monitored during the same period, where the average stream nitrate concentration was low (less than 10 µM). Furthermore, the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratios in these forested catchments were compared with those reported in past studies to verify the reliability of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio as an index of nitrogen saturation.

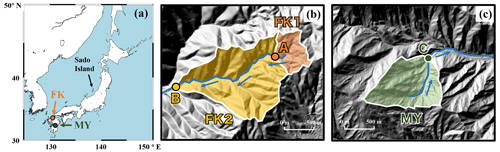
Figure 1 A map showing the locations of the study catchments (FK and MY) in Japan (a), and the maps of FK1, FK2 (b), and MY catchments (c), shown by orange, yellow, and green areas, respectively, together with the sampling station A, B, and C, respectively, shown by orange, yellow, and green circles, respectively. The blue arrows indicate the flow direction of stream water.

2 Methods

2.1 Study sites

The FK forested catchments (33^∘38^′ N, 130^∘31^′ E) are located in a suburban area about 15 km west of the Fukuoka metropolitan area (the fourth largest metropolitan area in Japan). The main plantation in these catchments was Japanese cedar/cypress (Table 1). The MY forested catchment (32^∘22^′ N, 131^∘09^′ E) is located in a rural area at the village of Shiiba in southern Japan's central Kyushu mountain range. This catchment is a mixed forest consisting of coniferous trees such as Abies firma Sieb. & Zucc. and Tsuga sieboldii Carr., as well as deciduous broadleaved trees such as Quercus crispula Blume, Fagus crenata Blume, and Acer sieboldianum Miq. Details on the studied forested catchments have been described in the past studies (Chiwa, 2020, 2021).

Table 1 Plant information for each forested catchment (Chiwa, 2021).

2.2 Sampling

The stream water eluted from the FK1 (14 ha), FK2 (62 ha), and MY (43 ha) forested catchments was collected about once every month in principle from November 2019 to December 2021 (Fig. 1). At the FK catchments, stream water was collected at upstream (station A) and downstream (station B) locations (Fig. 1b). At the MY catchment, stream water was collected at station C (Fig. 1c). Samples of stream water to determine the concentration and stable isotopic compositions (δ¹⁵N, δ¹⁸O, and Δ¹⁷O) of stream nitrate were collected manually in bottles washed with deionized water before sampling and then rinsed at least twice with the sample before sampling at each sampling site.

2.3 Analysis

All the stream water samples were passed through a membrane filter (pore size 0.45 µm) within 2 days after sampling and stored in a refrigerator (4 ^∘C) until analysis. The concentrations of nitrate were measured by ion chromatography (Prominence HIC-SP, Shimadzu, Japan). To determine the stable isotopic compositions of nitrate in the stream water samples, nitrate in each sample was chemically converted to N₂O using a method originally developed to determine the ${}^{\mathrm{15}}\mathrm{N}{/}^{\mathrm{14}}\mathrm{N}$ and ${}^{\mathrm{18}}\mathrm{O}{/}^{\mathrm{16}}\mathrm{O}$ ratios of seawater and freshwater nitrate (McIlvin and Altabet, 2005) that was later modified (Konno et al., 2010; Tsunogai et al., 2011; Yamazaki et al., 2011). In brief, 11 mL of each sample solution was pipetted into a vial with a septum cap. Then, 0.5 g of spongy cadmium was added, followed by 150 µL of a 1 M NaHCO₃ solution. The sample was then shaken for 18–24 h at a rate of 2 cycles s⁻¹. Then, the sample solution (10 mL) was decanted into a different vial with a septum cap. After purging the solution using high-purity helium, 0.4 mL of an azide–acetic acid buffer, which had also been purged using high-purity helium, was added. After 45 min, the solution was alkalinized by adding 0.2 mL of 6 M NaOH. Then, the stable isotopic compositions (δ¹⁵N, δ¹⁸O, and Δ¹⁷O) of the N₂O in each vial were determined using the continuous-flow isotope ratio mass spectrometry (CF-IRMS) system at Nagoya University. The analytical procedures performed using the CF-IRMS system were the same as those detailed in previous studies (Hirota et al., 2010; D. D. Komatsu et al., 2008). The obtained values of δ¹⁵N, δ¹⁸O, and Δ¹⁷O for the N₂O derived from the nitrate in each sample were compared with those derived from our local laboratory nitrate standards to calibrate the values of the sample nitrate to an international scale and to correct for both isotope fractionation during the chemical conversion to N₂O and the progress of oxygen isotope exchange between the nitrate-derived reaction intermediate and water (ca. 20 %). In this study, we adopted the internal standard method to calibrate the stable isotopic compositions of sample nitrate. Specifically, three kinds of the local laboratory nitrate standards were used in this study, which were named to be GG01 (δ¹⁵N = −3.07 ‰, δ¹⁸O = +1.10 ‰, and Δ¹⁷O = 0 ‰), HDLW02 (δ¹⁵N = +8.94 ‰, δ¹⁸O = +24.07 ‰), and NF (Δ¹⁷O = +19.16 ‰), which the GG01 and the HDLW02 were used to determine the δ¹⁵N and δ¹⁸O of stream nitrate, and the GG01 and the NF was used to determine the Δ¹⁷O of stream nitrate. The GG01, HDLW02, and NF had been calibrated using the internationally distributed isotope reference materials (USGS 34 and USGS 35). The oxygen exchange rate between nitrate and water during the chemical conversion was calculated through Eq. (2):

$$\begin{array}{}\text{(2)}& \begin{array}{rl}& \mathrm{Oxygen}\mathrm{exchange}\mathrm{rate}\left(\mathit{\%}\right)=\\ & {{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{N}}_{\mathrm{2}}\mathrm{O}\right)}_{\mathrm{NF}}/{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{NO}}_{\mathrm{3}}^{-}\right)}_{\mathrm{NF}},\end{array}\end{array}$$

where the Δ¹⁷O(N₂O)_NF denote the Δ¹⁷O value of N₂O that convert from the NF nitrate, and the Δ¹⁷O(NO${}_{\mathrm{3}}^{-}$)_NF denote the Δ¹⁷O value of NF nitrate (Δ¹⁷O = +19.16 ‰) (Tsunogai et al., 2016; Nakagawa et al., 2013, 2018; Ding et al., 2022).

The δ²H and δ¹⁸O values of H₂O of the stream water samples were analyzed using the cavity ring-down spectroscopy method by employing an L2120-i instrument (Picarro Inc., Santa Clara, CA, USA) equipped with an A0211 vaporizer and autosampler. The errors (standard errors of the mean) in this method were ±0.5 ‰ for δ²H and ±0.1 ‰ for δ¹⁸O. Both the VSMOW and standard light Antarctic precipitation (SLAP) were used to calibrate the values to the international scale. The δ¹⁸O values of H₂O were used to calibrate the differences in δ¹⁸O of H₂O between the samples and those our local laboratory nitrate standard samples (Tsunogai et al., 2010, 2011, 2014).

To determine whether the conversion rate from nitrate to N₂O was sufficient, the concentration of nitrate in the samples was determined each time we analyzed the isotopic composition using CF-IRMS based on the N₂O⁺ or O${}_{\mathrm{2}}^{+}$ outputs. We adopted the δ¹⁵N, δ¹⁸O, and Δ¹⁷O values only when the concentration measured via CF-IRMS correlated with the concentration measured via ion chromatography prior to isotope analysis within a difference of 10 %. We repeated the analysis of δ¹⁵N, δ¹⁸O, and Δ¹⁷O values for each sample at least three times to attain high precision. All samples had a nitrate concentration of greater than 3.5 µM, which corresponded to a nitrate quantity greater than 35 nmol in a 10 mL sample. Thus, all isotope values presented in this study have an error (standard error of the mean) better than ±0.2 ‰ for δ¹⁵N, ±0.3 ‰ for δ¹⁸O, and ±0.1 ‰ for Δ¹⁷O.

Nitrite (NO${}_{\mathrm{2}}^{-}$) in the samples interferes with the final N₂O produced from nitrate, because the chemical method also converts NO${}_{\mathrm{2}}^{-}$ to N₂O (McIlvin and Altabet, 2005). Therefore, it is sometimes necessary to remove NO${}_{\mathrm{2}}^{-}$ prior to converting nitrate to N₂O. In this study, however, we skipped the processes for removing NO${}_{\mathrm{2}}^{-}$ because all the stream samples analyzed for stable isotopic composition had NO${}_{\mathrm{2}}^{-}$ concentrations lower than the detection limit (0.05 µM).

2.4 Deposition rate of atmospheric nitrate

The annual deposition rate of atmospheric nitrate (D_atm; total dry and wet deposition rate of atmospheric nitrate) in each catchment was estimated using the annual “bulk” deposition rate of atmospheric nitrate (D_bulk) calculated in Chiwa (2020) at each catchment by multiplying the volume-weighted mean concentration of nitrate in the bulk deposition samples collected every 2 weeks at each catchment for 10 years (from January 2009 to December 2018) by the annual amount of precipitation. The bulk deposition samples were those accumulated in a plastic bucket installed in an open site of each catchment 55 cm above the ground. The distances between the monitoring sites of bulk deposition in the FK1, FK2, and MY forested catchments and the stations of stream water sampling (stations A, B, and C) were 3.9, 2.9, and 4.5 km, respectively. The concentrations of nitrate in the bulk deposition samples were measured by ion chromatography.

The D_bulk determined through this method, however, is less than D_atm (Aikawa et al., 2003) because the dry deposition velocities of gases and particles on the water surface of the plastic bucket are smaller than those on the forest (Matsuda, 2008). Thus, we corrected the differences by using Eq. (3) to estimate D_atm from D_bulk:

$$\begin{array}{}\text{(3)}& D_{\mathrm{atm}}=D_{\mathrm{bulk}}-D_{\mathrm{dry}}\left(W\right)+D_{\mathrm{dry}}\left(F\right),\end{array}$$

where D_dry(W) and D_dry(F) denote the annual dry deposition rates onto water and forest, respectively.

The D_dry(W) and D_dry(F) at each catchment were determined using an inferential method (Endo et al., 2011) through Eqs. (4) and (5), respectively:

$$\begin{array}{}\text{(4)}& {\displaystyle }\begin{array}{rl}{D}_{\mathrm{dry}}\left(W\right)& =[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}{]}_{\mathrm{gas}}\times V_{\mathrm{gas}}(W)\\ & +[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}{]}_{\mathrm{p}}\times V_{\mathrm{p}}(W),\end{array}\text{(5)}& {\displaystyle }\begin{array}{rl}{D}_{\mathrm{dry}}\left(F\right)& =[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}{]}_{\mathrm{gas}}\times V_{\mathrm{gas}}(F)\\ & +[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}{]}_{\mathrm{p}}\times V_{\mathrm{p}}(F),\end{array}\end{array}$$

where [NO${}_{\mathrm{3}}^{-}$_atm]_gas denotes the concentration of gaseous nitrate in air; [NO${}_{\mathrm{3}}^{-}$_atm]_p denotes the concentration of particle nitrate in air; V_gas(W) and V_gas(F) denote the deposition velocities of gaseous nitrate on the water surface and forest, respectively; and V_p(W) and V_p(F) denote the deposition velocities of particulate nitrate on the water surface and forest, respectively. Those determined by Chiwa (2010) using the annular denuder method from May 2006 to April 2007 were used for the [NO${}_{\mathrm{3}}^{-}$]_gas and [NO${}_{\mathrm{3}}^{-}$]_p in the FK catchments. Those determined by the National Institute for Environmental Studies (Environmental Laboratories Association of Japan, 2017) using the filter-pack method at Miyazaki (31^∘83^′ N, 131^∘42^′ E) from 2011 to 2017 were used for the [NO${}_{\mathrm{3}}^{-}$]_gas and [NO${}_{\mathrm{3}}^{-}$]_p in the MY catchment. The V_gas(F), V_gas(W), V_p(F), and V_p(W) of each catchment were determined by applying the estimation file for dry deposition (Matsuda, 2008; http://www.hro.or.jp/list/environmental/research/ies/katsudo/acid_rain/kanseichinchaku/kanseichinchaku.html, last access: 20 December 2022), where V_gas and V_p were calculated using the meteorological data of wind speed, temperature, humidity, radiation, cloud amount, and land use. The meteorological data monitored by the Japan Meteorological Agency at the nearest Fukuoka station (33^∘34^′ N, 130^∘22^′ E) and Miyazaki station (31^∘56^′ N, 131^∘24^′ E) from 2009 to 2021 were used for the FK and MY catchments, respectively. The forested land use of 100 % was chosen for each area.

2.5 Flux of stream water

The flux of stream water (F_stream) in each catchment was not measured fully in this study. Instead, the water balance in each catchment was used to estimate F_stream, assuming that the outflux of water from the study catchments to deep groundwater was negligible:

$$\begin{array}{}\text{(6)}& F_{\mathrm{stream}}=P-E,\end{array}$$

where P denotes the annual average precipitation and E denotes the annual evapotranspiration flux of water in each catchment. In this paper, the equation obtained by H. Komatsu et al. (2008) was used to estimate the E of the FK and MY catchments. Details on this equation are shown below.

H. Komatsu et al. (2008) compiled the annual flux of evapotranspiration determined in 43 forested catchments in Japan and found that E shows a positive correlation with the average temperature (T_avg) of each catchment. Thus, they proposed the modeled relation of E (mm) = 31.4 T_avg (^∘C) + 376 to estimate E in each forested catchment in Japan, where the standard error of 162.3 mm was included in the estimated evapotranspiration flux (E). They also confirmed that the estimated F_stream using the model corresponded well with the observed F_stream in three forested catchments, with estimated errors of less than 6 %. As a result, we utilized the water balance method proposed by H. Komatsu et al. (2008) to quantify the F_stream in each catchment.

2.6 Concentration of unprocessed NO${}_{\mathrm{3}}^{-}$_atm in each water sample

The Δ¹⁷O data of nitrate in each sample were used to estimate the concentration of NO${}_{\mathrm{3}}^{-}$_atm ([NO${}_{\mathrm{3}}^{-}$_atm]) in each water sample by applying Eq. (7):

$$\begin{array}{}\text{(7)}& \left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]/\left[{\mathrm{NO}}_{\mathrm{3}}^{-}\right]={\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}/{\mathrm{\Delta }}^{\mathrm{17}}{\mathrm{O}}_{\mathrm{atm}},\end{array}$$

where [NO${}_{\mathrm{3}}^{-}$_atm] and [NO${}_{\mathrm{3}}^{-}$] denote the concentrations of NO${}_{\mathrm{3}}^{-}$_atm and nitrate (total) in each water sample, respectively, and Δ¹⁷O_atm and Δ¹⁷O denote the Δ¹⁷O values of NO${}_{\mathrm{3}}^{-}$_atm and nitrate (total) in the stream water sample, respectively. In this study, we used the annual average Δ¹⁷O value of NO${}_{\mathrm{3}}^{-}$_atm determined at the Sado-Seki monitoring station in Japan (Sado Island; Fig. 1a) from April 2009 to March 2012 (Δ¹⁷O${}_{\mathrm{atm}}=+\mathrm{26.3}$ ‰; Tsunogai et al., 2016) for Δ¹⁷O_atm in Eq. (7) to estimate [NO${}_{\mathrm{3}}^{-}$_atm] in the stream. We allow for an error range of 3 ‰ in Δ¹⁷O_atm, where the factor changes in Δ¹⁷O_atm from +26.3 ‰ caused by both areal and seasonal variations in the Δ¹⁷O values of NO${}_{\mathrm{3}}^{-}$_atm have been considered (Nakagawa et al., 2018; Tsunogai et al., 2016; Ding et al., 2022).

The annual export flux of unprocessed NO${}_{\mathrm{3}}^{-}$_atm per unit area of the catchment (M_atm) was determined by applying Eq. (8):

$$\begin{array}{}\text{(8)}& M_{\mathrm{atm}}=[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}{]}_{\mathrm{avg}}\times F_{\mathrm{stream}},\end{array}$$

where [NO${}_{\mathrm{3}}^{-}$_atm]_avg denotes the annual average [NO${}_{\mathrm{3}}^{-}$_atm] in each stream. The index of nitrogen saturation ($M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio) was calculated by dividing M_atm with D_atm in each catchment.

2.7 Concentration and isotopic compositions of stream nitrate eluted only from the FK2 catchment

The concentration and isotopic compositions (δ¹⁵N, δ¹⁸O, and Δ¹⁷O) of stream nitrate determined at station B were the mixtures of those eluted from FK1 and FK2 catchments (Fig. 1b). Assuming that the stream nitrate eluted from FK1 catchment was stable during the flow path from station A to station B, the concentration of stream nitrate eluted from the FK2 catchment was determined by applying Eq. (9):

$$\begin{array}{}\text{(9)}& \begin{array}{rl}[{\mathrm{NO}}_{\mathrm{3}}^{-}{]}_{\mathrm{FK}\mathrm{2}}& =\left(\right[{\mathrm{NO}}_{\mathrm{3}}^{-}{]}_{\mathrm{FK}\mathrm{1}+\mathrm{FK}\mathrm{2}}\cdot F_{\mathrm{FK}\mathrm{1}+\mathrm{FK}\mathrm{2}}\\ & -[{\mathrm{NO}}_{\mathrm{3}}^{-}{]}_{\mathrm{FK}\mathrm{1}}\cdot F_{\mathrm{FK}\mathrm{1}})/F_{\mathrm{FK}\mathrm{2}},\end{array}\end{array}$$

where F_FK1, F_FK2, and F_FK1+FK2 denote the flux of stream water eluted from the FK1, FK2 (only), and FK1+FK2 catchment, respectively. [NO${}_{\mathrm{3}}^{-}$]_FK1, [NO${}_{\mathrm{3}}^{-}$]_FK2, and [NO${}_{\mathrm{3}}^{-}$]_FK1+FK2 denote the concentration of stream nitrate eluted from the FK1, FK2 (only), and FK1+FK2 catchment, respectively. In this study, the flow rates measured at stations A and B on 15 January 2021 by using the salt dilution method (Sappa et al., 2015) were used for F_FK1 (0.85 L s⁻¹) and F_FK1+FK2 (4.75 L s⁻¹), respectively, and the measured [NO${}_{\mathrm{3}}^{-}$] at stations A and B was used for [NO${}_{\mathrm{3}}^{-}$]_FK1 and [NO${}_{\mathrm{3}}^{-}$]_FK1+FK2, respectively. Because the relation between the measured flow rates was comparable with the relation between the catchment area of FK1 (14 ha) and that of FK1+FK2 (76 ha), we concluded that the measured flow rates of 0.85 L s⁻¹ and 4.75 L s⁻¹ were reasonable as for those representing the F_FK1 and F_FK1+FK2, respectively. According to the mass balance of water, we can estimate the F_FK2 eluted from the FK2 catchment only to be 3.90 L s⁻¹.

Assuming that the stream nitrate eluted from the FK1 catchment was stable during the flow path from station A to station B, the δ¹⁵N, δ¹⁸O, and Δ¹⁷O values of stream nitrate eluted from the FK2 catchment only were determined by applying Eq. (10):

$$\begin{array}{}\text{(10)}& \begin{array}{rl}{\mathit{\delta }}_{\mathrm{FK}\mathrm{2}}& =({\mathit{\delta }}_{\mathrm{FK}\mathrm{1}+\mathrm{FK}\mathrm{2}}\cdot [{\mathrm{NO}}_{\mathrm{3}}^{-}{]}_{\mathrm{FK}\mathrm{1}+\mathrm{FK}\mathrm{2}}\cdot F_{\mathrm{FK}\mathrm{1}+\mathrm{FK}\mathrm{2}}\\ & -{\mathit{\delta }}_{\mathrm{FK}\mathrm{1}}\cdot [{\mathrm{NO}}_{\mathrm{3}}^{-}{]}_{\mathrm{FK}\mathrm{1}}\cdot F_{\mathrm{FK}\mathrm{1}})/\left(\right[{\mathrm{NO}}_{\mathrm{3}}^{-}{]}_{\mathrm{FK}\mathrm{2}}\cdot F_{\mathrm{FK}\mathrm{2}}),\end{array}\end{array}$$

where δ_FK1, δ_FK2, and δ_FK1+FK2 denote the δ¹⁵N (or δ¹⁸O or Δ¹⁷O) of stream nitrate eluted from the FK1, FK2, and FK1+FK2 catchment, respectively. The δ¹⁵N (or δ¹⁸O or Δ¹⁷O) values of stream nitrate measured at stations A and B were used for δ_FK1 and δ_FK1+FK2, respectively.

3 Results

3.1 Deposition rate of atmospheric nitrate

The mean annual precipitation (P) from 2009 to 2021 was 1777 and 3981 mm for FK and MY catchments, respectively (Chiwa, 2020; Masaaki Chiwa, personal communication, 21 September 2022). The mean annual temperature (T_avg) was reported to be 15.9 and 10.8 ^∘C for FK and MY catchments, respectively (Chiwa, 2020). Based on these data, the annual flux of stream water (F_stream) was estimated to be 902.0±162.3 mm at FK catchments and 3266.1±162.3 mm at MY catchment, respectively, using Eq. (6).

Chiwa (2020) reported the annual bulk deposition rates of atmospheric nitrate (D_bulk) to be 34.0 mmol m⁻² yr⁻¹ at the FK catchments and 24.2 mmol m⁻² yr⁻¹ at the MY catchment. On the other hand, the annual dry deposition rate of atmospheric nitrate (D_dry) deposited on the forest (D_dry(F)) and on the water surface (D_dry(W)) were estimated to be 39.9 and 4.1 mmol m⁻² yr⁻¹, respectively, at FK catchments and 18.4 and 2.4 mmol m⁻² yr⁻¹, respectively, at MY catchment. As a result, D_atm was estimated to be 69.3 mmol m⁻² yr⁻¹ at FK catchments and 40.1 mmol m⁻² yr⁻¹ at MY catchment using Eq. (3).

3.2 Concentration and isotopic composition of stream nitrate

The concentrations of stream nitrate eluted from the FK1, FK2 (only), and MY catchments ranged from 97.5 to 121.3 µM, from 65.7 to 148.5 µM, and from 3.5 to 15.3 µM, respectively, with the average concentrations of 109.5, 90.9, and 7.3 µM, respectively, and the standard deviations (SD) of 6.3, 18.5, and 3.0 µM, respectively, which correspond to the coefficients of variation (CV) of 5.7 %, 20.4 %, and 40.7 %, respectively (Fig. 2a). All catchments showed no clear seasonal variation during the observation periods. The variation ranges and the average concentrations of stream nitrate eluted from the three catchments agreed well with the past observations performed in the same catchments (Chiwa, 2021).

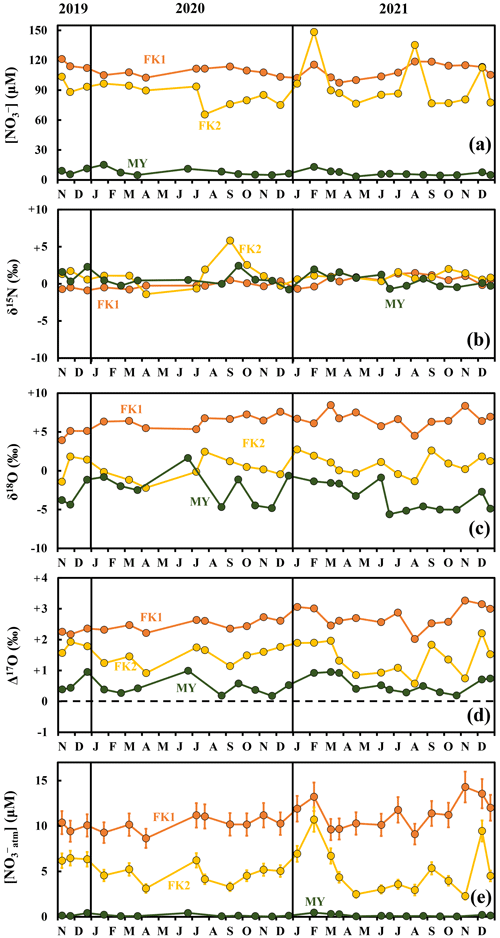
Figure 2 Temporal variations in concentrations of stream nitrate (FK1: orange circles; FK2: yellow circles; MY: green circles) (a), together with those in δ¹⁵N (b), δ¹⁸O (c), and Δ¹⁷O (d) of nitrate, and the concentration of unprocessed NO${}_{\mathrm{3}}^{-}$_atm ([NO${}_{\mathrm{3}}^{-}$_atm]) (e) in the stream water of the FK1, FK2, and MY forested catchments. Error bars smaller than the sizes of the symbols are not presented.

The stable isotopic compositions of stream nitrate eluted from the FK1, FK2 (only), and MY catchments ranged from −0.9 ‰ to +1.5 ‰, from −1.4 ‰ to +5.8 ‰, and from −0.8 ‰ to +2.4 ‰, respectively, for δ¹⁵N (Fig. 2b), from +3.9 ‰ to +8.5 ‰, from −2.2 ‰ to +2.8 ‰, and from −5.6 ‰ to +1.7 ‰, respectively, for δ¹⁸O (Fig. 2c), and from +2.0 ‰ to +3.3 ‰, from +0.6 ‰ to +2.2 ‰, and from +0.2 ‰ to +1.0 ‰, respectively, for Δ¹⁷O (Fig. 2d), with no clear seasonal variation during the observation periods. The concentration-weighted averages for the δ¹⁵N, δ¹⁸O, and Δ¹⁷O values of stream nitrate were +0.2 ‰, +6.4 ‰, and +2.6 ‰, respectively, at FK1, +1.0 ‰, +0.5 ‰, and +1.5 ‰, respectively, at FK2, +0.7 ‰, −2.5 ‰, and +0.6 ‰, respectively, at MY.

3.3 Concentration of unprocessed atmospheric nitrate and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in each catchment

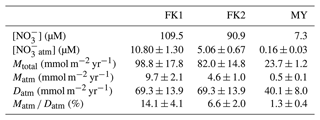
The concentration of unprocessed atmospheric nitrate ([NO${}_{\mathrm{3}}^{-}$_atm]) in the streams eluted from the FK1, FK2 (only), and MY catchments ranged from 8.64 to 14.30 µM, from 2.27 to 10.71 µM, and from 0.03 to 0.46 µM with the average concentration of 10.80 ± 1.30, 5.06 ± 0.67, and 0.16 ± 0.03 µM, respectively, even though these studied catchments showed little seasonal variations during the observation periods (Fig. 2e). The annual export flux of nitrate (M_total), the annual export flux of NO${}_{\mathrm{3}}^{-}$_atm (M_atm), and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio were 98.8 ± 17.8 mmol m⁻² yr⁻¹, 9.7 ± 2.1 mmol m⁻² yr⁻¹, and 14.1 ± 4.1 % at FK1 catchment, respectively, 82.0 ± 14.8 mmol m⁻² yr⁻¹, 4.6 ± 1.0 mmol m⁻² yr⁻¹, and 6.6 ± 2.0 % at FK2 catchment, respectively, 23.7 ± 1.2 mmol m⁻² yr⁻¹, 0.5 ± 0.1 mmol m⁻² yr⁻¹, and 1.3 ± 0.4 % at MY catchment, respectively (Table 2). The uncertainties of [NO${}_{\mathrm{3}}^{-}$_atm], M_atm, and $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in each catchment were determined from the uncertainties of Δ¹⁷O, Δ¹⁷O_atm, F_stream, and D_atm according to the equations of error propagation. The details were described in Appendix A.

Table 2 Average concentrations of stream nitrate ([NO${}_{\mathrm{3}}^{-}$]), the average concentrations of unprocessed NO${}_{\mathrm{3}}^{-}$_atm in streams ([NO${}_{\mathrm{3}}^{-}$_atm]), the annual export flux of NO${}_{\mathrm{3}}^{-}$ per unit area of catchments (M_total), the annual export flux of NO${}_{\mathrm{3}}^{-}$_atm per unit area of catchments (M_atm), the deposition flux of NO${}_{\mathrm{3}}^{-}$_atm per unit area of catchment (D_atm), and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratios in the study catchments.

4 Discussion

4.1 Deposition rate of atmospheric nitrate at the study catchments

Based on the air monitoring data determined at the stations of Fukuoka (33^∘51^′ N, 130^∘50^′ E) and Miyazaki (31^∘83^′ N, 131^∘42^′ E) from 2011 to 2017, the Environmental Laboratories Association of Japan (2017) reported D_atm to be 57.8 mmol m⁻² yr⁻¹ at Fukuoka and 49.1 mmol m⁻² yr⁻¹ at Miyazaki. Those values are consistent with the D_atm estimated in this study (69.3 and 40.1 mmol m⁻² yr⁻¹ at the FK and MY catchments, respectively), within a difference of approximately 20 %. Thus, we concluded that the D_atm estimated in this study was reliable within the error margin of 20 % (Table 2). Because the D_atm determined at the FK catchments was the highest among the forested catchments in Table 3, we further compared the D_atm of the FK catchments with those from the other air monitoring stations in Japan reported in past studies, along with that of the MY catchment (Table S1 in the Supplement). While the D_atm of the MY catchment corresponded to the average level among the sites compiled in Table S1, the D_atm of the FK catchments exceeded the average level significantly. In addition, the D_atm of the FK catchments corresponded to one of the highest among the Japanese forested areas (Table S1). All the catchments in Japan can be suffered from the long-range transport of air pollutants derived from megacities in the East Asian region (Chiwa, 2021; Chiwa et al., 2012, 2013). In addition, the shorter transport distance from the Fukuoka metropolitan area (total population: 1.62 million people; population density: 4715 people km⁻²) may be mainly responsible for the D_atm higher in FK than in MY, because the FK catchments are only 15 km west of the Fukuoka metropolitan area.

Table 3 The annual amount of precipitation (P), the average concentration of stream nitrate ([NO${}_{\mathrm{3}}^{-}$]), the nitrogen saturation stage, the average concentration of unprocessed NO${}_{\mathrm{3}}^{-}$_atm in streams ([NO${}_{\mathrm{3}}^{-}$_atm]), the annual export flux of NO${}_{\mathrm{3}}^{-}$ per unit area of catchment (M_total), the annual export flux of NO${}_{\mathrm{3}}^{-}$_atm per unit area of catchment (M_atm), the deposition flux of NO${}_{\mathrm{3}}^{-}$_atm per unit area of catchment (D_atm), and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in the FK1, FK2, and MY, along with those in the catchments studied in past studies using Δ¹⁷O of nitrate as a tracer.

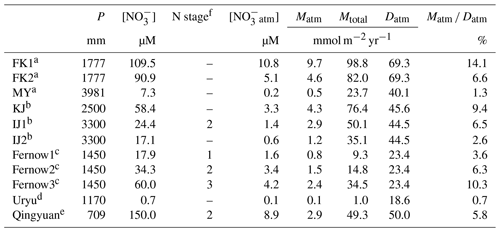
^a This study. ^b Nakagawa et al. (2018), Nakahara et al. (2010). ^c Rose et al. (2015). ^d Tsunogai et al. (2014). ^e Huang et al. (2020). ^f N saturation stage estimated in past studies – means no data.

4.2 Excess leaching of unprocessed atmospheric nitrate from FK catchments

The isotopic compositions (δ¹⁵N, δ¹⁸O, and Δ¹⁷O) of stream nitrate eluted from the FK and MY catchments were typical for those eluted from forested catchments (Hattori et al., 2019; Huang et al., 2020; Nakagawa et al., 2013, 2018; Riha et al., 2014; Sabo et al., 2016; Tsunogai et al., 2014, 2016). The striking features found in the FK catchments were that, in addition to the high [NO${}_{\mathrm{3}}^{-}$] and high M_total that had been clarified in a past study (Chiwa, 2021), both [NO${}_{\mathrm{3}}^{-}$_atm] and M_atm in FK were higher than those eluted from MY (Table 2). Especially, the average [NO${}_{\mathrm{3}}^{-}$_atm] in the stream eluted from the FK1 catchment was the highest ever reported in forested streams determined through continuous monitoring for more than 1 year (Bostic et al., 2021; Bourgeois et al., 2018a, b; Hattori et al., 2019; Huang et al., 2020; Nakagawa et al., 2018; Rose et al., 2015; Sabo et al., 2016; Tsunogai et al., 2014, 2016).

The observed high [NO${}_{\mathrm{3}}^{-}$_atm] in the stream eluted from the FK1 catchment could be caused just by the high [NO${}_{\mathrm{3}}^{-}$_atm] deposition in the catchment. Thus, we compiled all past data ever reported in forested streams through continuous monitoring in Table 3, where the data of average [NO${}_{\mathrm{3}}^{-}$], average [NO${}_{\mathrm{3}}^{-}$_atm], M_atm, M_total, D_atm, and $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio were included for comparison. The result showed that the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio, along with M_atm, was the highest as well in the FK1 catchment among the forested catchments (Table 3).

Elevated loading of nitrogen through atmospheric deposition was responsible for the occurrence of nitrogen saturation in forest ecosystems, from which elevated levels of nitrate are exported (Aber et al., 1989). Nakagawa et al. (2018) proposed that the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio can be an index for evaluating the nitrogen saturation in each forested catchment, because the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio directly reflects the present demand for atmospheric nitrate deposited in each forested catchment, and thus reflects the nitrogen saturation in each forested catchment. The high $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratios observed in the FK catchments implied that the demand for atmospheric nitrate was low in the FK catchments and that the stages of nitrogen saturation at the FK catchments were higher than those at other forested catchments. That is, the nitrogen saturation at the FK catchments was responsible for the observed high [NO${}_{\mathrm{3}}^{-}$] and high M_total at the FK catchments than at MY and any other catchment ever studied (Table 3).

The stand age of forests can affect the retention or loss of N (Fukushima et al., 2011; Ohrui and Mitchell, 1997). Fukushima et al. (2011) evaluated N uptake rates of Japanese cedars at different ages (5–89 years old) and demonstrated that the N uptake rates of Japanese cedars were higher in younger stands (53 kg N ha⁻¹ yr⁻¹ in 16 years old) than in older stands (29 kg N ha⁻¹ yr⁻¹ in 31 years old; 24 kg N ha⁻¹ yr⁻¹ in 42 years old; 34 kg N ha⁻¹ yr⁻¹ in 89 years old). In addition, Yang and Chiwa (2021) found that the nitrate concentration in the soil water taken beneath the rooting zone of matured artificial Japanese cedar plantations (607 ± 59 µM; 64–69 years old) was significantly higher than that of normal Japanese oak plantations (8.7 ± 8.1 µM; 24 years old). Moreover, by adding ammonium nitrate (50 kg N ha⁻¹ yr⁻¹) to the forest floor directly, Yang and Chiwa (2021) found that the nitrate concentration in the soil water of the matured artificial Japanese cedar plantations increased significantly faster than that of the normal Japanese oak plantations, probably because of the lower N uptake rates in the matured artificial Japanese cedar plantations. Because most of the artificial Japanese cedar/cypress plantations in the FK and MY catchments have reached their maturity (>50 years; Yang and Chiwa, 2021), the higher proportion of matured artificial Japanese cedar/cypress plantations in the FK1 catchment (Table 1) was highly responsible for the observed elevated leaching of nitrate, caused by the reduction in N uptake rates.

As a result, we concluded that the FK forested catchments were under the high nitrogen saturation stage, FK1 catchment especially, and the nitrogen saturation in the FK1 catchment was responsible for the elevated M_total, M_atm, [NO${}_{\mathrm{3}}^{-}$], [NO${}_{\mathrm{3}}^{-}$_atm] found in the stream eluted from the catchment (Fig. 3a, b, c and d).

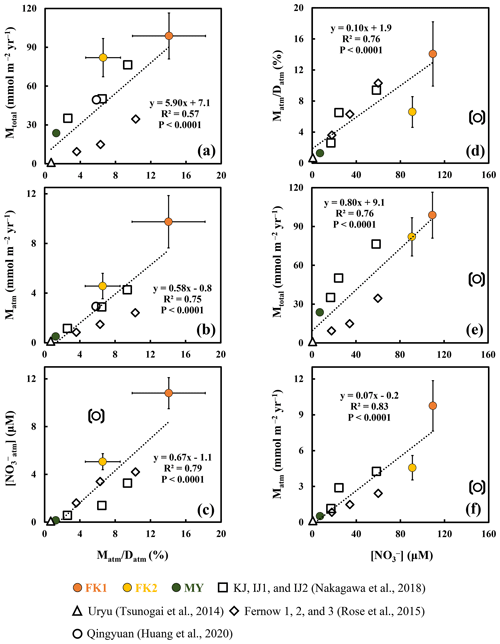
Figure 3 Annual export flux of nitrate per unit area (M_total) plotted as a function of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in each forested catchment (a); the annual export flux of unprocessed atmospheric nitrate per unit area (M_atm) plotted as a function of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio (b); the average concentration of NO${}_{\mathrm{3}}^{-}$_atm ([NO${}_{\mathrm{3}}^{-}$_atm]) plotted as a function of the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio (c); the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio plotted as a function of the average concentration of nitrate ([NO${}_{\mathrm{3}}^{-}$]) (d); the M_total plotted as a function of [NO${}_{\mathrm{3}}^{-}$] (e); the M_atm plotted as a function of [NO${}_{\mathrm{3}}^{-}$] (f) (FK1: orange circles; FK2: yellow circles; MY: green circles). Those determined for the forested catchments in past studies are plotted as well (Qingyuan: white circle, Huang et al., 2020; KJ, IJ1, and IJ2: white squares, Nakagawa et al., 2018; Fernow 1, 2, and 3: white diamonds, Rose et al., 2015; Uryu: white triangle, Tsunogai et al., 2014). The data obtained in the Qingyuan forested catchment are shown in parentheses and excluded from the calculation to estimate correlation coefficients (see text for the reason).

4.3 The $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio as an index of nitrogen saturation

Past studies have used the concentration of stream nitrate as one of the important indexes to evaluate the stage of nitrogen saturation in each forest (Aber, 1992; Huang et al., 2020; Rose et al., 2015; Stoddard, 1994). The strong linear relationship (R²=0.76; P<0.0001) between the stream nitrate concentration and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio, except for the Qingyuan forested catchment (Fig. 3d), further supported that the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio can be used as an alternative index of nitrogen saturation, as pointed out in Nakagawa et al. (2018).

The differences in the number of storm and/or snowmelt events could affect the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio as well, because NO${}_{\mathrm{3}}^{-}$_atm could be injected into the stream water directly, along with the storm/snowmelt water (Tsunogai et al., 2014; Ding et al., 2022; Inamdar and Mitchell, 2006). In a recent study, however, we found that storm events have little impact on the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio, based on monitoring temporal variation of [NO${}_{\mathrm{3}}^{-}$_atm] in stream water during storm events (Ding et al., 2022). In addition, the low $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio found in Uryu forested catchment (0.7 %; Table 3) implied that the snowmelt has little impact on the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio as well, because 30 % of the annual mean precipitation was snow in Uryu forested catchment (Tsunogai et al., 2014).

The differences in the amount of precipitation, temperature, and the flux of stream water could affect the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio as well. As a result, the annual amount of precipitation, mean temperature, and the annual mean flux of stream water (F_stream) in the forested catchments were compiled in Table S2. While the stream nitrate concentration showed a strong linear relationship (R²=0.76; P<0.0001) with the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio (Fig. 3d), the precipitation, temperature, and F_stream did not show a significant relationship with the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio (P>0.14; Fig. 4). As a result, we concluded that the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio was mainly controlled by the progress of nitrogen saturation, rather than the differences in the number of storm and/or snowmelt events, the amount of precipitation, temperature, and the flux of stream water.

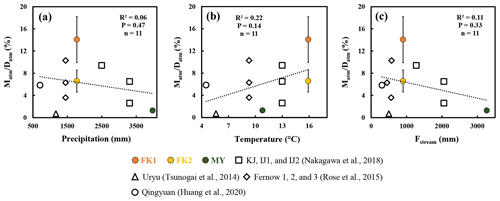
Figure 4 The $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio plotted as a function of the amount of precipitation (a), the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio plotted as a function of the temperature (b), and the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio plotted as a function of flux of stream water (c) (FK1: orange circles; FK2: yellow circles; MY: green circles). Those determined for the forested catchments in past studies are plotted as well.

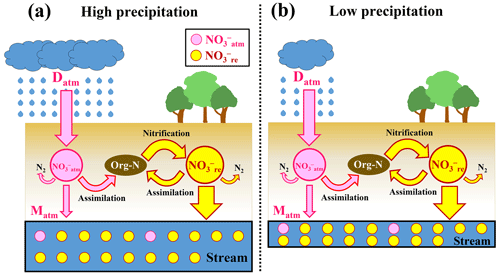
Figure 5 Schematic diagram showing the biogeochemical processing of nitrate in forested catchments under high precipitation (a) and low precipitation (b), where NO${}_{\mathrm{3}}^{-}$_atm (unprocessed atmospheric nitrate) is represented by pink circles, NO${}_{\mathrm{3}}^{-}$_re by yellow circles, the flows of NO${}_{\mathrm{3}}^{-}$_atm by pink arrows, and those of NO${}_{\mathrm{3}}^{-}$_re (remineralized nitrate) by yellow arrows (modified after Nakagawa et al., 2018). Although the deposition rates of NO${}_{\mathrm{3}}^{-}$_atm (D_atm) and the biogeochemical reaction rates between (a) and (b) are the same, we can expect high [NO${}_{\mathrm{3}}^{-}$] in (b). On the other hand, the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio between (a) and (b) are the same.

The differences in the residence time of water in each catchment could also impact the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio, as the residence time of water in forested catchments ranges from one month to more than one year (Asano et al., 2002; Farrick and Branfireun, 2015; Kabeya et al., 2008; Rodgers et al., 2005; Soulsby et al., 2006; Tetzlaff et al., 2007). While the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio could be higher in catchments with a shorter water residence time, it is difficult to explain high [NO${}_{\mathrm{3}}^{-}$] and high M_total eluted from the catchment by the residence time of water alone, as the majority of nitrate eluted from the catchment with a high $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio was NO${}_{\mathrm{3}}^{-}$_re produced by microbial nitrification. The significant correlation between M_total and $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratios (P<0.0001; Fig. 3a) supported nitrogen saturation as the leading cause of high M_total in catchments with a high $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio. Additionally, the high loading of atmospheric nitrogen, the type of plantation, and the old age of plantation in the FK1 catchment all supported the conclusion that the FK1 catchment was under nitrogen saturation.

The $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio is a more reliable and robust index than the stream nitrate concentration, as explained below. The Qingyuan forested catchment can be classified into the highest nitrogen saturation stage based only on the highest stream nitrate concentration of 150 µM (Table 3). However, based on the leaching flux of nitrogen via stream water monitored by Huang et al. (2020) for 4 years in the Qingyuan forested catchment, along with the deposition flux of nitrogen, we can obtain the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in the catchment to be a medium level of 5.8 ± 1.3 %, implying that the nitrogen saturation stage was not so high (Table 3). Huang et al. (2020) also concluded that the input of nitrogen exceeded the output in the catchment, and thus, the catchment was at stage 2 of nitrogen saturation. The $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio in the Qingyuan forested catchment with a medium level among all forested catchments (Fig. 3d) should be a more reliable index of nitrogen saturation.

Compared with those in the other forested catchments in Table 3, the annual amount of precipitation (P) has the lowest value of 709 mm in the Qingyuan forested catchment. The flux of stream water (F_stream) has the lowest value of 309 mm as well. Thus, we concluded that nitrate was relatively concentrated in the catchment because of the small precipitation, resulting in relative enrichment in the concentrations of both nitrate (150 µM) and unprocessed atmospheric nitrate (8.9 µM) in the stream.

While the concentration of stream nitrate, as an index of nitrogen saturation traditionally, can be influenced by the amount of precipitation, as demonstrated in the Qingyuan forested catchment, the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio is independent of the amount of precipitation (Fig. 5). Therefore, the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio can be used as a more robust index for evaluating nitrogen saturation in each forested catchment.

5 Conclusions

Both the concentrations and Δ¹⁷O of stream nitrate were determined for more than 2 years in the forested catchments of FK (FK1 and FK2) and MY to determine the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio for each catchment. The FK catchments exhibited higher $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio than the MY catchment and other forested catchments reported in past studies, implying that the progress of nitrogen saturation in the FK catchments was severe. Both age and proportion of artificial plantation in the FK catchments were responsible for the progress of nitrogen saturation. In addition, although past studies have commonly used the concentration of stream nitrate as an index to evaluate the progress of nitrogen saturation in forested catchments, it can be influenced by the amount of precipitation. As a result, we concluded that the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio should be used as a more reliable index for evaluating the progress of nitrogen saturation because the $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio is independent from the amount of precipitation.

Appendix A: Calculating of uncertainties in the values of [NO${}_{\mathrm{3}}^{-}$_atm], M_atm, and $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio

The uncertainty in the values of [NO${}_{\mathrm{3}}^{-}$_atm] was estimated from the uncertainties in the Δ¹⁷O values of stream nitrate (Δ¹⁷O) and NO${}_{\mathrm{3}}^{-}$_atm (Δ¹⁷O_atm) according to the divisive equation of error propagation (Eq. A1):

$$\begin{array}{}\text{(A1)}& \begin{array}{rl}{\mathit{\sigma }}_{\left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]}& =\left[{\mathrm{NO}}_{\mathrm{3}}^{-}\right]\\ & \cdot \sqrt{{\left({\displaystyle \frac{\mathrm{1}}{{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}_{\mathrm{atm}}}}\cdot {\mathit{\sigma }}_{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}\right)}^{\mathrm{2}}+{\left({\displaystyle \frac{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}{{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}_{\mathrm{atm}}^{\mathrm{2}}}}\cdot {\mathit{\sigma }}_{{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}_{\mathrm{atm}}}\right)}^{\mathrm{2}}},\end{array}\end{array}$$

where ${\mathit{\sigma }}_{\left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]}$, ${\mathit{\sigma }}_{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}$, and ${\mathit{\sigma }}_{{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}_{\mathrm{atm}}}$ denote the uncertainties in [NO${}_{\mathrm{3}}^{-}$_atm], Δ¹⁷O values of stream nitrate, and Δ¹⁷O values of NO${}_{\mathrm{3}}^{-}$_atm, respectively. The standard error of the mean (SE) of ±0.1 ‰ and the areal/seasonal variations of ±3 ‰ was used in calculating ${\mathit{\sigma }}_{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}$ and ${\mathit{\sigma }}_{{{\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}}_{\mathrm{atm}}}$, respectively. As a result, the uncertainty in [NO${}_{\mathrm{3}}^{-}$_atm] (${\mathit{\sigma }}_{\left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]}$) was ±1.30, ±0.67, and ±0.03 µM at FK1, FK2, and MY catchments, respectively.

The uncertainty in the values of M_atm was estimated from the uncertainties in [NO${}_{\mathrm{3}}^{-}$_atm] and in F_stream according to the multiplicative equation of error propagation (Eq. A2):

$$\begin{array}{}\text{(A2)}& {\mathit{\sigma }}_{M_{\mathrm{atm}}}=\sqrt{(F_{\mathrm{stream}}\cdot {\mathit{\sigma }}_{\left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]}{)}^{\mathrm{2}}+(\left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]\cdot {\mathit{\sigma }}_{F_{\mathrm{stream}}}{)}^{\mathrm{2}}},\end{array}$$

where ${\mathit{\sigma }}_{M_{\mathrm{atm}}}$, ${\mathit{\sigma }}_{\left[{{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\mathrm{atm}}\right]}$, and ${\mathit{\sigma }}_{F_{\mathrm{stream}}}$ denote the uncertainties in M_atm, [NO${}_{\mathrm{3}}^{-}$_atm], and F_stream, respectively. H. Komatsu et al. (2008) proposed the uncertainty in F_stream to be ±162.3 mm when using the water balance method in estimating F_stream. Here, the uncertainty in M_atm (${\mathit{\sigma }}_{M_{\mathrm{atm}}}$) was ±2.1, ±1.0, and ±0.1 mmol m⁻² yr⁻¹ at FK1, FK2, and MY catchments, respectively.

The uncertainty in $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio was estimated from the uncertainties in M_atm and in D_atm according to the divisive equation of error propagation (Eq. A3):

$$\begin{array}{}\text{(A3)}& {\mathit{\sigma }}_{M_{\mathrm{atm}}/D_{\mathrm{atm}}\mathrm{ratio}}=\sqrt{{\left({\displaystyle \frac{\mathrm{1}}{D_{\mathrm{atm}}}}\cdot {\mathit{\sigma }}_{M_{\mathrm{atm}}}\right)}^{\mathrm{2}}+{\left({\displaystyle \frac{M_{\mathrm{atm}}}{D_{{\mathrm{atm}}^{\mathrm{2}}}}}\cdot {\mathit{\sigma }}_{D_{\mathrm{atm}}}\right)}^{\mathrm{2}}},\end{array}$$

where ${\mathit{\sigma }}_{M_{\mathrm{atm}}/D_{\mathrm{atm}}\mathrm{ratio}}$, ${\mathit{\sigma }}_{M_{\mathrm{atm}}}$, and ${\mathit{\sigma }}_{D_{\mathrm{atm}}}$ denote the uncertainty in $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio, M_atm, and D_atm, respectively. Comparing the deposition rate of NO${}_{\mathrm{3}}^{-}$_atm obtained at the other atmospheric monitoring stations nearby, the uncertainty of 20 % was adopted for those of D_atm in each catchment, which corresponds to the uncertainty in D_atm of ±13.9, ±13.9, ±8.0 mmol m⁻² yr⁻¹ at FK1, FK2, and MY catchments, respectively. As a result, the uncertainty in $M_{\mathrm{atm}}/D_{\mathrm{atm}}$ ratio was ± 4.1 %, ±2.0 %, and ±0.4 % at FK1, FK2, and MY catchments, respectively.