the Creative Commons Attribution 4.0 License.
the Creative Commons Attribution 4.0 License.
Southern Ocean controls of the vertical marine δ13C gradient – a modelling study
Jörg Schwinger
Christoph Heinze
δ13C, the standardised 13C ∕ 12C ratio expressed in per mille, is a widely used ocean tracer to study changes in ocean circulation, water mass ventilation, atmospheric pCO2, and the biological carbon pump on timescales ranging from decades to tens of millions of years. δ13C data derived from ocean sediment core analysis provide information on δ13C of dissolved inorganic carbon and the vertical δ13C gradient (i.e. Δδ13C) in past oceans. In order to correctly interpret δ13C and Δδ13C variations, a good understanding is needed of the influence from ocean circulation, air–sea gas exchange and biological productivity on these variations. The Southern Ocean is a key region for these processes, and we show here that Δδ13C in all ocean basins is sensitive to changes in the biogeochemical state of the Southern Ocean. We conduct a set of idealised sensitivity experiments with the ocean biogeochemistry general circulation model HAMOCC2s to explore the effect of biogeochemical state changes of the Southern and Global Ocean on atmospheric δ13C, pCO2, and marine δ13C and Δδ13C. The experiments cover changes in air–sea gas exchange rates, particulate organic carbon sinking rates, sea ice cover, and nutrient uptake efficiency in an unchanged ocean circulation field. Our experiments show that global mean Δδ13C varies by up to about ±0.35 ‰ around the pre-industrial model reference (1.2 ‰) in response to biogeochemical change. The amplitude of this sensitivity can be larger at smaller scales, as seen from a maximum sensitivity of about −0.6 ‰ on ocean basin scale. The ocean's oldest water (North Pacific) responds most to biological changes, the young deep water (North Atlantic) responds strongly to air–sea gas exchange changes, and the vertically well-mixed water (SO) has a low or even reversed Δδ13C sensitivity compared to the other basins. This local Δδ13C sensitivity depends on the local thermodynamic disequilibrium and the Δδ13C sensitivity to local POC export production changes. The direction of both glacial (intensification of Δδ13C) and interglacial (weakening of Δδ13C) Δδ13C change matches the direction of the sensitivity of biogeochemical processes associated with these periods. This supports the idea that biogeochemistry likely explains part of the reconstructed variations in Δδ13C, in addition to changes in ocean circulation.
- Article
(3760 KB) - Full-text XML
-
Supplement
(1840 KB) - BibTeX
- EndNote
The vertical marine δ13C gradient (Δδ13C) is the surface-to-deep difference in δ13C of dissolved inorganic carbon (DIC), where the standardised 13C ∕ 12C ratio (δ13C) is expressed in per mille (Zeebe and Wolf-Gladrow, 2001):
Here, 13C ∕ 12Cstandard is the Pee Dee Belemnite standard (0.0112372) (Craig, 1957). 13C is slightly heavier than the 12C isotope, which causes a fractionation effect during air–sea gas exchange and photosynthesis, thereby changing δ13C and Δδ13C (Laws et al., 1997; Zhang et al., 1995; Mackenzie and Lerman, 2006). Photosynthetic fractionation increases the 13C ∕ 12C ratio of surface ocean DIC (i.e. a δ13C increase) due to the preferred uptake of the lighter 12C into biogenic matter (which therefore has a low δ13C). The deep sea DIC has a relatively low δ13C signature as a result of the remineralisation of low-δ13C biogenic matter at depth. The resulting vertical δ13C gradient is in addition influenced by air–sea gas exchange and circulation (Zeebe and Wolf-Gladrow, 2001; Emerson and Hedges, 2008; Ziegler et al., 2013). Both deep sea and surface ocean δ13C signatures are archived in the calcareous shells of foraminifera in the sediments. Such records of δ13C from planktic and benthic foraminiferal shell material cover tens of millions of years (Hilting et al., 2008). Using this archive, δ13C and Δδ13C have been used to reconstruct, for example, atmospheric CO2 concentration, ocean circulation, and the strength of the biological pump (Broecker, 1982; Shackleton and Pisias, 1985; Zahn et al., 1986; Oppo et al., 1990; Hollander and McKenzie, 1991; Keir, 1991; Crucifix, 2005; Curry and Oppo, 2005; Lisiecki, 2010; Broecker and McGee, 2013; Ziegler et al., 2013; Hoogakker et al., 2015; Bauska et al., 2016). Δδ13C is independent of whole-ocean δ13C shifts (due to terrestrial influences), because such influences would affect δ13C equally everywhere. This makes Δδ13C a valuable proxy to study the marine carbon cycle independent of changes in carbon storage on land. Besides the use of δ13C for understanding the past ocean, contemporary measurements of δ13C of DIC support the quantification of anthropogenic carbon uptake by the oceans as well as the study of the effects of biology and ocean circulation on tracer distributions (Kroopnick, 1980, 1985; Gruber and Keeling, 2001; Quay et al., 2003; Holden et al., 2013; Eide et al., 2017b). However, major uncertainties remain in the interpretation of foraminiferal δ13C records and Δδ13C (Oliver et al., 2010; Broecker and McGee, 2013) as well as in the interpretation of the present-day δ13C data (Eide et al., 2017b).
This article addresses part of these uncertainties by exploring the pre-industrial sensitivity of δ13C and Δδ13C to biogeochemical change in idealised model experiments. By doing so we can investigate a number of biogeochemical mechanisms that could explain (part of) the observed changes in δ13C and Δδ13C. We focus on the Southern Ocean (SO), the ocean south of 45∘ S, because the SO plays an important role in the global carbon cycle by regulating atmospheric CO2 concentrations and uptake of anthropogenic CO2 (Broecker and Maier-Reimer, 1992; Heinze, 2002; Marinov et al., 2006) as well as influencing the global efficiency of the biological pump, global primary production, and preformed nutrients (Primeau et al., 2013).
Variations in Δδ13C over the past few 100 000 years show that Δδ13C is generally increased during glacial periods, due to a higher contrast of deep δ13C with surface and mid-depth δ13C (Broecker, 1982; Shackleton and Pisias, 1985; Boyle, 1988; Charles et al., 2010; Oliver et al., 2010). Long-term δ13C and Δδ13C variations have been explained by ocean circulation changes (Duplessy et al., 1988; Jansen, 2017; Oppo et al., 1990; Toggweiler, 1999; Menviel et al., 2016). However, Δδ13C variability cannot be explained by ocean stratification or circulation changes alone: an interaction between biogeochemical and physical processes must be at play (Keir, 1991; Boyle, 1988; Mulitza et al., 1998; Charles et al., 2010; Ziegler et al., 2013; Schmittner and Somes, 2016). Δδ13C has been used in different ways over time: in earlier studies as the contrast between surface and deep water δ13C, derived from planktic versus benthic foraminifera (Broecker, 1982; Boyle, 1988; Shackleton et al., 1983; Duplessy et al., 1988), and now increasingly as the contrast of deep ocean (benthic) δ13C with thermocline or intermediate ocean δ13C (Mulitza et al., 1998; Charles et al., 2010; Lisiecki, 2010).
Here, we explore the sensitivity of δ13C and Δδ13C to changes in the biogeochemical state of the Global Ocean and Southern Ocean under a constant circulation field, to support the paleo-oceanographic interpretation of δ13C and Δδ13C as well as to improve the understanding of the SO role in global carbon cycling and its variability and sensitivity. In order to study biogeochemical mechanisms that could influence δ13C and Δδ13C, a set of sensitivity experiments is conducted with the ocean biogeochemistry general circulation model HAMOCC2s (Heinze et al., 2016). We first estimate the contribution of biology versus air–sea gas exchange to marine δ13C of DIC (Sect. 3.2). The experiments focus on one or more of the biogeochemical aspects assumed to be important for δ13C and Δδ13C, e.g. the biological pump efficiency and/or equilibration at the air–sea interface (Sect. 3.3.1–3.3.4). Together these experiments provide a broad spectrum of biogeochemical changes that could influence local and global δ13C and Δδ13C. The modelling results of Sect. 3.3.1–3.3.4 are discussed in context of observational data from sediment cores (Sect. 3.4). As δ13C and Δδ13C are used to study changes in atmospheric pCO2 (), a final section will cover the relationship between atmospheric δ13C, Δδ13C, and under different marine biogeochemical states (Sect. 3.5).
In this study we employ the ocean biogeochemistry general circulation model HAMOCC2s (Heinze et al., 1999, 2016), which simulates the inorganic and organic carbon cycle in the water column and in the sediments. The horizontal resolution of the model is and there are 11 depth layers in the ocean. HAMOCC2s has an annual time step and an annually averaged fixed circulation field, as well as a one-layer atmosphere component (permitting exchange of O2, 13CO2, and CO2 with the ocean component), which is assumed to be longitudinally well-mixed. The model is computationally very economic and thus an ideal tool for sensitivity experiments over long integration times. Biogenic particulate matter in the model is represented as particulate organic carbon (POC), calcium carbonate (CaCO3), and biogenic silica (opal). These biogenic particles are only modelled as export production due to the annual time step of the model. POC and opal export production are described by Michaelis–Menten kinetics for nutrient uptake, limited by phosphate and silicic acid respectively (Sect. 1A in the Supplement). CaCO3 export production depends on the ratio between opal and POC production. POC is carried as a tracer as well as transported downwards according to a set of mass balance equations that describe POC gain through surface-layer POC production and POC losses through constant sinking and remineralisation rates (Sect. 1A in the Supplement). This is done similarly for opal and CaCO3 sinking and dissolution. As the model has an annual time step, sea ice is always present south of ∼60∘ S and north of ∼70∘ N in the control run (Fig. S1 in the Supplement). A more detailed model description is provided in previous studies using a similar configuration of HAMOCC2s (Heinze, 2002; Heinze et al., 2016), as well as in Sect. 1A in the Supplement.
Fractionation between 13C and 12C during photosynthesis is set to a constant value of −20 ‰ (Lynch-Stieglitz et al., 1995; Tagliabue and Bopp, 2008), as model results are little influenced by the chosen parameterisation (Schmittner et al., 2013; Jahn et al., 2015). The fractionation during air–sea gas exchange depends on temperature according to + 23.89 ‰, where temperature (T) is measured in Kelvin (K) (Mook, 1986), causing stronger fractionation at lower temperatures (i.e. at high latitudes). Fractionation during CaCO3 formation is omitted from the model, as was done in previous studies (Lynch-Stieglitz et al., 1995; Marchal et al., 1998; Schmittner et al., 2013), as its size is uncertain but likely minor (∼1 ‰) and effects on δ13C and Δδ13C are small (Shackleton and Pisias, 1985). In the version of HAMOCC2s used in this study, a fixed weathering input is used for 13C to tune the ocean inventory to values comparable to observations. The weathering flux of 13C into the ocean was determined by an iterative procedure: The model was run over 100 000 years, replacing the weathering rate with the diagnosed burial rate for 13C continuously. After this, the model 13C inventory was recalibrated such that the atmospheric value for δ13C arrived at −6.5 ‰. This procedure was repeated over three iterations. Afterwards, the weathering rate of 13C was fixed to the last diagnosed value (0.36 Tmol 13C yr−1) – which results in a weathering flux δ13C of DIC of −11 ‰ . Another 100 000-year run was carried out with this constant input rate in order to check whether the global 13C distribution was stable in all reservoirs. The sensitivity experiments were then re-started from the result of that run. Weathering fluxes are added homogeneously over the first ocean layer as dissolved matter and in a fixed stoichiometric ratio for CaCO3, organic carbon, , alkalinity, and Si. Annual weathering fluxes (Tmol) are 27 for CaCO3, 5 for organic carbon, 5/rC : P for , 2 × CaCO3-rN : P × for alkalinity, and 4.5 for Si (with and ). These values are within the uncertainties of observational estimates for Si (5.6 Tmol yr−1; Tréguer, 2002), CaCO3 (∼32 Tmol yr−1; Milliman and Droxler, 1996), and organic carbon (4 Tmol yr−1; Broecker and Peng, 1987) and have been adjusted to improve the fit of the respective modelled marine tracer distributions as well as burial fluxes to observational estimates. The spin-up procedure described here created a model set-up with close-to-observed marine and atmospheric δ13C (δ13Catm) values and freely evolving atmospheric pCO2 and δ13C. This equilibrated model version is referred to as the “control run” in the remainder of this article. We define the vertical δ13C gradient (Δδ13C) as follows:
where δ13Csurface and δ13Cdeep are the volume-weighted mean δ13C of DIC in the surface ocean (< 50 m depth, i.e. the model photic zone) and the deep ocean (lowermost wet layer in the model, if the top of the layer is > 3 km depth), respectively. By doing so, we can compare the Δδ13C summarised as one number between the different sensitivity experiments.
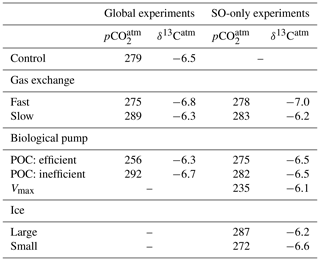
Table 1Description of the sensitivity experiments. The sensitivity experiments on the CO2 gas exchange rate and the biological pump have been done twice, once for the Global Ocean and once only making changes in the Southern Ocean (south of 45∘ S).

We conducted a set of sensitivity experiments to explore changes in air–sea gas exchange rate, sea ice extent (influencing both biological production and the air–sea gas exchange of carbon), and the efficiency of the biological pump through the POC sinking rate and nutrient uptake rate (Table 1). We employ the term “efficiency of the biological pump” as a measure of the success of phytoplankton to maintain low nutrient concentrations in the surface ocean. All experiments are run for 2000 model years starting from the end of the spin-up. These runtimes allowed for atmospheric quasi-equilibrium to establish (Fig. S5), with an exception for the long-term effects caused by POC sinking rate changes (as studied in more detail by Roth et al., 2014). The equilibration timescale of Δδ13C is much shorter than that of atmospheric δ13C: this is the case because (1) the long-term weathering-burial equilibration of δ13C affects the whole ocean reservoir simultaneously – thus keeping Δδ13C constant – and (2) the processes that potentially influence Δδ13C (changes in biological production and air–sea gas exchange) affect Δδ13C on shorter (centennial to millennial) timescales. In order to compare effects of SO change and global change, the gas exchange rate and POC sinking rate experiments are done twice – once changing the respective model parameter for the Global Ocean and once for the Southern Ocean only (SO-only). The model parameters were changed in a way that marine biogeochemical tracer distributions (e.g. , δ13C) remained reasonable but did provide an estimate of the sensitivity of the respective tracer to biogeochemical change. The model has a constant sea ice cover (Fig. S1), which permits gas transfer through the ice depending on ice cover thickness (the transfer rate is divided by ice thickness in centimetres) while light transfer is inhibited at ice thicknesses over 0.01 cm. The maximum and minimum sea ice cover experiments (“ice large” and “ice small”, Table 1) approximate the Last Glacial Maximum winter extent and the modern summer extent of SO sea ice, respectively (Crosta, 2009 and Fig. A22 therein) and assume full inhibition of gas and light transfer through ice for simplicity. The experiment on nutrient drawdown (Vmax) alters the Michaelis–Menten kinetics of POC production by changing the maximum nutrient (i.e. ) uptake rate ( in Sect. 1A in the Supplement). The gas exchange experiments alter the specific gas exchange rate kw, as described in more detail in Sect. 1B in the Supplement. The POC sinking rate experiments change the sinking velocity constant wPOC in the POC mass balance equations (Sect. 1A in the Supplement).
The contribution of biological processes versus air–sea gas exchange to δ13C is calculated using the method of Broecker and Maier-Reimer (1992) as done for observations by Eide et al. (2017b) and in a modelling context by Sonnerup and Quay (2012):
where , and the following model control run mean values are used: =2332.284 µmol kg−1, µmol kg−1 and ‰. These values result in the modelled δ13Cbio : relationship δ13Cbio . The constant 3.18 is somewhat higher than estimated for observed δ13C, for which a constant of 2.8 was found by Eide et al. (2017b). This higher constant originates from the over-prediction of the model mean δ13C and at depth, as seen in other models (Sonnerup and Quay, 2012). Equation (3) assumes a constant biological fractionation as well as a constant rC : P ratio, and these assumptions will introduce some error in the partition of biological and air–sea gas exchange signatures derived from observed δ13C to ratios (e.g. Eide et al. 2017b). For the purpose of determining δ13Cbio in our model, these assumptions are unproblematic, since rC : P and εphoto are actually taken to be constant in the model formulation. The air–sea gas signature δ13CAS is approximated as the residual (δ13CAS = is 0 ‰ when δ13Cmodel = δ13Cbio, i.e. when the δ13C can be explained by biology only. We express δ13Cbio as a percentage to aid interpretation of the results (denoted ), because the values of δ13Cbio in per mille depend strongly on the chosen “reference” values, i.e. mean DIC, , and δ13C (compare Broecker and Maier-Reimer, 1992; Lynch-Stieglitz et al., 1995; Sonnerup and Quay, 2012; Schmittner et al., 2013; Eide et al., 2017b). The conversion from δ13Cbio to a percentage is calculated as follows:
In our analysis, we define the total amount of air–sea carbon exchange as , with Fup as the upward annual carbon flux from the ocean into the atmosphere and Fdown its downward counterpart (Sect. 1B in the Supplement and Heinze and Maier-Reimer, 1999). Fu+d is relevant for understanding the sensitivity of δ13C. The net carbon exchange is defined as . The sign of Fnet indicates whether a region is a source or a sink for carbon and is relevant for understanding changes in .
3.1 Model control run
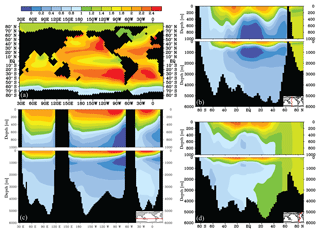
The model reproduces the main features of observed marine δ13C, as shown in Figs. 1 and S2. The modelled global mean surface ocean δ13Csurface of DIC is higher (1.88 ‰) than deep ocean δ13Cdeep (0.67 ‰), creating a mean ocean Δδ13C of 1.21 ‰. In the North Atlantic and SO, Δδ13C is the least pronounced (0.9 ‰ and 0.8 ‰ respectively) due to vertical mixing between surface and deep water during deep water formation and upwelling (Duplessy et al., 1988). Δδ13C increases with water mass age as expected from the increased imprint of remineralisation on δ13C. The mean modelled ocean δ13C is higher by 0.16 ‰ relative to observations (Eide et al., 2017b), which is especially pronounced in the oldest water masses (Fig. S2). This is observed in other models as well and attributed to the model's relative contribution of deep water production in the North Atlantic and Southern Ocean (Sonnerup and Quay, 2012). The modelled global export POC production is 9.6 Gt C yr−1, of which 18 % is produced in the SO, which is within the uncertainty of observational estimates (MacCready and Quay, 2001; Schlitzer, 2002; Dunne et al., 2007; Lutz et al., 2007; Nevison et al., 2012). The atmosphere has a modelled equilibrium of 279 ppm and a δ13Catm of −6.50 ‰ , which developed in the model from the “best-fit” weathering value as described above in Sect. 2. Net air–sea gas exchange is close to zero (ventilating Gt of carbon to the atmosphere annually). The resulting drift of the model control over 2000 years is ‰ for δ13Catm, and ppm for .
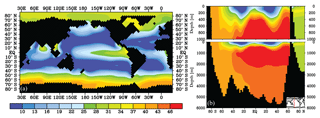
Figure 2, the contribution of biology to the local δ13C signal (%), as calculated using Eq. (4) at (a) 25 m depth and (b) a Pacific transect. The remainder of 100 % is attributed to air–sea gas exchange. The δ13Cbio and δ13CAS values in per mille are very similar to the values found by Schmittner et al. (2013).
3.2 Air–sea gas exchange versus biology
The contribution of biology based on Eqs. (2) and (3) to the δ13C distribution is presented in Fig. 2, broadly in agreement with previous studies (Kroopnick, 1985; Schmittner et al., 2013). The contribution of biology to the modelled δ13C distribution is generally below 45 % and has a steep gradient from the surface to the deep ocean. The (thermodynamic) fractionation effect of air–sea gas exchange on δ13C is strongly impeded by the long equilibration time of 13C, which leaves room for biological processes to contribute significantly to δ13C and Δδ13C (Lynch-Stieglitz et al., 1995; Murnane and Sarmiento, 2000; Schmittner et al., 2013; Eide et al., 2017a). In the ocean below 250 m, the influence of biology increases to 35 %–45 % due to the remineralisation of POC, with the exception of the Arctic Ocean, where no POC production is modelled due to the sea ice cover (Figs. 2b and S1). is close to 50 % around 1000 m depth in the northern Pacific and Indian oceans, due to the old water masses located there, which have accumulated a large fraction of remineralised DIC. At the surface, air–sea gas exchange dominates the δ13C signature of DIC, as is visible from the low (Fig. 2a). The only exception at the surface is in upwelling regions, where a relatively high is expected due to high POC production and upwelled remineralised carbon. High generally corresponds to a low-δ13C water mass (compare Figs. 1 and 2), as expected from the upwelling of 13C-depleted DIC and modelled and observed close to the Antarctic continent (Fig. 1a and observations by Eide et al., 2017a). The results presented in Fig. 2 appear to be quite robust as typically does not change by more than 5 %–10 % for the wide range of biogeochemical states, as is explored in the sensitivity experiments presented below.
3.3 Sensitivity of Δδ13C and δ13C
3.3.1 Air–sea gas exchange of carbon
Atmospheric and marine carbon isotopic ratios are generally in thermodynamic disequilibrium due to their relatively long equilibration timescales compared to the time of contact of a water parcel with the atmosphere. CO2 equilibration through the air–sea interface takes ∼4 months (Jones et al., 2014) and is inversely related to the Revelle buffer factor, and slowed down by a factor of ∼20 compared to inert gases due to carbon speciation (i.e. the adjustment of the bicarbonate pool). Again ∼10 times slower than CO2, the air–sea equilibration of the atmospheric isotope ratio 13CO2 ∕ 12CO2 (i.e. δ13Catm) with marine DI13C ∕ DI12C (i.e. δ13C) takes ∼4 years (Jones et al., 2014). The equilibration timescale of the carbon isotopes is not facilitated by the buffering reaction of CO2 with H2O, but instead depends on the DIC : CO2 ratio of seawater (Broecker and Peng, 1974; Jones et al., 2014; Galbraith et al., 2015). Over 90 % of the surface ocean δ13C signature is set by air–sea gas exchange outside of upwelling regions across the world's oceans (Fig. 2), making the equilibration across the air–sea interface important for surface ocean δ13C. Understanding the effects of equilibration across the air–sea interface is thus key to understanding global surface ocean δ13C signatures. Here we explore two extreme cases, very slow but non-zero gas exchange (“gas slow”, gas exchange rate divided by 4) and very fast gas exchange to bring the air–sea equilibration close to equilibrium (“gas fast”, gas exchange rate multiplied by 4).
Our results show that the effects of changes in air–sea gas exchange on δ13C mainly depend on the prior disequilibrium δ13Cdiseq (δ13Cdiseq = δ13C-δ13Ceq, where δ13Ceq represents the δ13C value a water parcel would have had if it would have fully equilibrated with the atmosphere; see also Gruber et al., 1999). Full isotopic equilibrium with the atmosphere results in a δ13Csurface of ∼0.5 to ∼4 ‰ at low and high latitudes, respectively (Menviel et al., 2015), where the range is caused by the temperature-dependent fractionation (Mook et al., 1986; Zhang et al., 1995). In this study, modelled ε is between 7.7 ‰ and 11 ‰ . This thermodynamic effect increases the difference between δ13Csurface and δ13Ceq at the poles (Menviel et al., 2015), thus increasing the potential of high-latitude surface water to be affected by air–sea gas exchange fluxes.
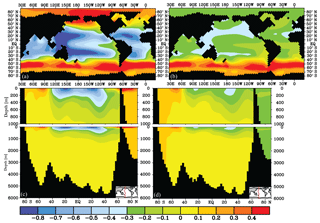
Figure 3δ13C of DIC (‰) difference after 2000 years for the fast gas exchange experiments (experiment − control): global experiments (a, c) and SO-only experiments (b, d), at 25 m depth (a, b) and as a Pacific transect of δ13C difference (c, d).
Our gas exchange experiments (Table 1) show a transient phase where the net global air–sea gas exchange flux Fnet is non-zero, which affects until a new quasi-equilibrium is established (Fig. S3). We find an increase in by 10 ppm (slow gas exchange) and a decrease by 4 ppm (fast gas exchange) after 2000 years, respectively. If gas exchange is only changed in the SO (i.e. for 22 % of the global ice-free ocean area), an effect of 3.7 and −0.7 ppm is found after 2000 years (Table 2). Gas exchange in the SO can thus cause a disproportionate response (∼30 % of the sensitivity) in . These changes occur in the first ∼600 years of the sensitivity experiments (Fig. S3), with the strongest changes occurring after ∼100 years. The air–sea pCO2 difference is smaller at increased gas exchange rates and larger at reduced gas exchange rates (Fig. S4). δ13Catm decreases by 0.3 ‰ during fast gas exchange and increases by 0.2 ‰ when the gas exchange rate is reduced. This is explained by the increase in the global amount of air–sea gas exchange Fu+d in the fast gas exchange experiment (4 times more, at 542 Gt C yr−1). Such an increase leads to a smaller thermodynamic disequilibrium, which increases the mean marine δ13C and lowers δ13Catm. Slow gas exchange reduces Fu+d (by 73 % to 36 Gt C yr−1), thus decreasing the role of air–sea gas exchange on surface ocean δ13C. This results in an increased contrast between atmospheric and surface ocean δ13C, which explains the increase in δ13Catm. Moreover, our SO-only experiments show that these effects on δ13Catm are more pronounced if gas exchange only changes in the SO. This indicates that the remainder of the ocean offsets part of the atmospheric sensitivity to SO change.
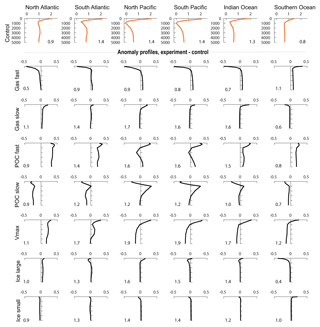
Figure 4Volume-weighted basin mean anomaly profiles of δ13C after 2000 years, with respect to the control profiles (upper row). Δδ13C denoted per basin in the lower right (control) and lower left (sensitivity experiments) corner of each subgraph. Results are presented for the global gas exchange and POC sinking experiments. Basin extent is visualised in Fig. S8.
δ13C shows a different response in high latitudes compared to the lower latitudes in the surface ocean (Figs. 3a and S5): an increased air–sea gas exchange rate lowers the surface ocean δ13C of DIC by 0.2 ‰ to 0.9 ‰ at the lower latitudes and increases surface ocean δ13C at high latitudes by 0.2 ‰–0.5 ‰ (Figs. 3 and 4). The direction of the response indicates whether δ13Cdiseq is positive or negative, and is in line with previous studies (Schmittner et al., 2013; Galbraith et al., 2015) that show that the disequilibrium is negative (δ13C < δ13Ceq) at high latitudes and in low-latitude upwelling regions, and positive elsewhere. The sign of δ13Cdiseq, and thus the direction of the δ13C response, is understood from the difference between the natural δ13C distribution (Fig. 1) and the δ13Ceq, which depends on thermodynamic fractionation (Sect. 2). At increased gas exchange rates (i.e. closer to equilibrium), δ13C has to increase in cool high-latitude surface waters and has to decrease in warm low-latitude surface waters in order to get closer to equilibrium (Menviel et al., 2015; Murnane and Sarmiento, 2000). The net effect of a slower gas exchange rate on surface ocean δ13C is less pronounced than the effect of an increased gas exchange rate (Figs. S5, 3). The smaller effects seen for slow gas exchange indicate that the control model ocean is a “slow ocean”, i.e. closer to (very) slow gas exchange than to thermodynamic equilibrium (infinitely fast gas exchange).
The effect of the gas exchange rate on marine δ13C is mostly established in the top 250 to 1000 m of the water column (Figs. 3c, d, 4). Recording this air–sea gas exchange signal thus strongly depends on the reliability of planktic δ13C-based δ13Csurface reconstructions and knowledge of the living depth represented by the planktic foraminifera. The signal penetrates deepest (to ∼2000 m depth) into the North Atlantic (Fig. 4), where δ13C is strongly influenced by air–sea gas exchange (Fig. 2a). However, the interpretation of variations in North Atlantic benthic δ13C as coming partly from air–sea gas exchange (Lear et al., 2016) is not supported by our experiment. Due to the limited export of the δ13C signal to depth, the sensitivity of Δδ13C to the gas exchange rate mainly comes from surface ocean δ13C. Globally, the Δδ13C is weakened to 0.87 ‰ when the thermodynamic disequilibrium is decreased (i.e. “Gas fast”, Fig. 5) and Δδ13C strengthens to 1.32 ‰ when the thermodynamic disequilibrium is increased (“Gas slow”, Fig. 5). The extent to which thermodynamic equilibrium can develop is thus an efficient way to change the biologically induced Δδ13C (Murnane and Sarmiento, 2000); however, this is only true at lower latitudes where δ13Cdiseq is positive: the direction of the high-latitude SO Δδ13C sensitivity mirrors the sensitivity of the low-latitude regions (Fig. 4) as well as the global mean due to its negative δ13Cdiseq.
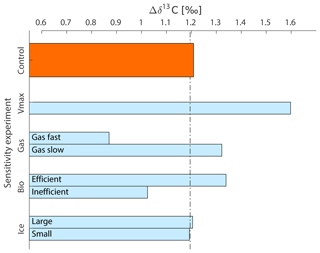
Figure 5Global mean Δδ13C after 2000 years for the different sensitivity experiments (Table 1). “Bio – Efficient” represents the high POC sinking rate experiment, “Bio – Inefficient” the slow POC sinking rate experiment. The results for the Southern Ocean-only experiments (Sect. 2) are described in the text.
3.3.2 The biological pump: POC sinking rate
The net effect of a regionally changed biological pump efficiency depends on the sequestration efficiency, which depends on the interplay between the biological pump and ocean circulation (DeVries et al., 2012). A more efficient biological pump (here, a higher POC sinking rate) leads to a loss of carbon to the sediments, which affects and δ13Catm on millennial timescales. Here we present results from a 2000-year simulation (as for the other experiments), which are thus transient results. A full equilibrium of the system could take as long as 200 000 years (Roth et al., 2014). On these long timescales other processes and feedbacks would occur (Tschumi et al., 2011), which complicates the attribution of changes to a primary trigger. A fast POC sinking rate leads to a decrease of 23 ppm and higher (+0.2 ‰) atmospheric δ13C after 2000 years (Table 2, Fig. S3) as well as an increase in mean ocean δ13C of 0.15 ‰ , caused by the transient sediment burial of low-δ13C POC. The transient imbalance between weathering and burial fluxes can thus cause profound changes in both marine and atmospheric δ13C, and moreover provides an important feedback for the long-term marine carbon cycle (Tschumi et al., 2011; Roth et al., 2014). In our experiment, an efficient biological pump leads to a global ∼6 % decrease in the amount of air–sea gas exchange Fu+d because of efficient export of carbon to depth, thereby lowering the net upward advection of carbon. A mirrored but weaker response is modelled for a decrease in biological pump efficiency: halving the POC sinking rate leads to a 13 ppm increase in (of which 23 % can be explained by the SO) and a more negative atmospheric δ13C (−6.7 ‰ ) and 7 % increased Fu+d (Table 2, Fig. S3).
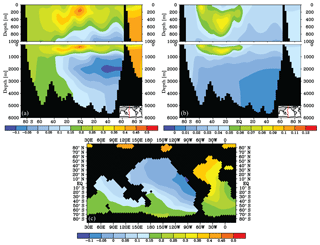
Figure 6δ13C of DIC (‰) difference after 2000 years for the POC sinking rate experiments (experiment − control): (a) the global efficient biological pump (high POC sinking rate) experiment for a Pacific transect and (b) the SO-only efficient biological pump experiment for a Pacific transect and (c) the global efficient biological pump experiment at 3000 m depth. Note the different scales.
Surface ocean δ13C is mostly influenced by the changes in productivity and the vertical displacement of the POC remineralisation depth. The deepening of the remineralisation depth has been extensively discussed in the literature (Boyle, 1988; Keir, 1991; Mulitza et al., 1998; Roth et al., 2014) and likely explains lowered mid-depth glacial δ13C together with changes in ocean circulation (for example, Toggweiler, 1999). POC sinking removes nutrients and preferentially light 12C carbon from the surface ocean, while exporting them to the deep ocean. If POC sinking rates are high, this increases the mean surface ocean δ13C (contributing to the δ13Catm increase) by 0.12 ‰ and lowers mean deep ocean δ13C by 0.01 ‰ (Fig. 6) – with values corrected for the overall 0.15 ‰ increase in marine δ13C, which occurs due to transient imbalance between weathering and sediment burial. Therefore, even though the absolute export production is globally reduced by 26 %, the biological pump is more efficient as any new nutrients in the surface ocean will immediately be used and exported. With a halving of the POC sinking rate, the remineralisation is confined closer to the surface ocean (Fig. 4). The net effect is that surface ocean δ13C is reduced (by a mean of 0.21 ‰ – corrected for the mean ocean change of 0.04 ‰) throughout the ocean (Fig. 4), because the fractionation effect during photosynthesis is counteracted by the remineralisation of POC (which would normally have occurred at depth). The SO plays a relatively minor role in the sensitivity to the POC experiments (Fig. 6b). Changes in deep ocean δ13C depend on the water mass age (Fig. 6c): old water (North Pacific) has a larger remineralisation signal when the biological pump is efficient. Independent of the biological pump efficiency, the relatively young waters of the deep North Atlantic generally adopt about the same δ13C signal as the surface ocean δ13C, which is set by air–sea gas exchange. This is in agreement with a relatively low estimate for the deep North Atlantic (∼30 %).
The sensitivity of Δδ13C to changes in POC sinking rate depends strongly on location (Figs. 4 and 6). In general, the Δδ13C is strengthened for an increased biological pump efficiency (Fig. 5), and this effect is stronger with water mass age (Figs. 6c and 4). The downward shift of the remineralisation depth of low-δ13C POC drives this increase in Δδ13C, a mechanism discussed by Boyle (1988) and Mulitza et al. (1998), among others, to understand glacial Δδ13C increase. Our results show that the vertical displacement of the δ13C profile is most pronounced in the North and South Pacific (Fig. 4). The North Atlantic Δδ13C is much less affected as these waters are mostly influenced by air–sea gas exchange. Instead, the entire North Atlantic profile is shifted more than in the other ocean basins (Fig. 4). Δδ13C weakens for a reduced biological pump efficiency (Figs. 4 and 5), especially in older water where is higher (Fig. 2a). It is worth noting, however, that the changes in Δδ13C in the SO are comparably small because the vertical mixing in the SO of the low-δ13C deep water mostly causes shifts in the entire δ13C profile, not a change in the gradient (Fig. 4).
3.3.3 The biological pump: SO nutrient depletion
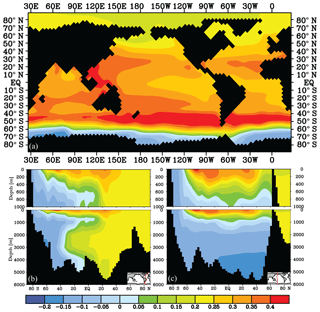
Consistent with previous studies (Sarmiento et al., 2004; Marinov et al., 2006; Primeau et al., 2013), we find a large atmospheric impact of our SO nutrient depletion experiment. The high SO nutrient uptake efficiency (i.e. an efficient biological pump) causes a 44 ppm reduction in after 2000 years. The Vmax experiment reaches quasi-equilibrium after ∼800 years, as seen from the time evolution of and δ13Catm (Fig. S3). δ13Catm increases to −6.1 ‰ due to the increased surface ocean δ13C (Fig. 7a). This 0.4 ‰ increase is high compared to the results of Menviel et al. (2015), who found a δ13Catm sensitivity of 0.1 ‰–0.2 ‰ in response to changes in SO nutrient utilisation. The different development time compared to the fast POC sinking rate experiment is explained by the absence of long-term loss of carbon to the sediments in the Vmax experiment, because transport and water-column remineralisation within the SO limits an increase in POC burial there. In the SO, net carbon uptake (Fnet) increases fourfold to 1.5 Gt C yr−1 (Fig. S6) because the high nutrient and carbon consumption transport carbon into the ocean interior and do not permit CO2 to escape to the atmosphere.
SO export production of POC is increased (Fig. S7) by a factor of 2.4, causing global POC export production to increase by 15 %, albeit reducing lower-latitude productivity (non-SO up to ∼35∘ N) by 13 %. This relocation of global POC export production in response to SO increased nutrient uptake efficiency is in agreement with earlier studies (Marinov et al., 2006; Primeau et al., 2013). The increased surface ocean δ13C signature everywhere north of the SO sea ice edge (Fig. 7a) is in the SO attributed to increased POC export production counteracted by a decreased Fu+d (which would reduce δ13Csurface in the SO because of the negative δ13Cdiseq; Figs. 3 and S5). At lower latitudes, the decreased Fu+d (which increases δ13Csurface in lower latitudes because of the positive δ13Cdiseq; Figs. 3 and S5) dominates the effect of the 13 % lower POC export production on δ13Csurface. At depth and under the sea ice in the Antarctic, where deep water upwells, the imprint of additional POC remineralisation at depth decreases δ13C of DIC (Fig. 7). This decrease in δ13C is only visible in water masses downstream of the SO (Fig. 7b and c) and is most pronounced in the deep North Pacific (Fig. 7c). The increased nutrient uptake rate in the SO increases global mean Δδ13C by 0.4 ‰ (Fig. 5) as well as increasing Δδ13C in all ocean basins (Fig. 4), as seen for the fast POC sinking rate experiment. Δδ13C is affected more in older waters, where a more pronounced remineralisation imprint has developed (Fig. 4). Besides effects on the δ13C distribution (Fig. 7), the O2 and distributions change as well: the O2 distribution is reorganised such that surface ocean O2 is increased (by up to 20 µmol kg−1, with largest changes in the SO), while deep ocean O2 is reduced downstream of the SO (by up to 40 µmol kg−1). Surface ocean is reduced mostly in the SO (by up to 0.8 µmol kg−1). This signal is, however, too small to significantly increase mean deep ocean . This implies a reduction in global preformed phosphate governed by the efficient nutrient uptake in the SO; see also Primeau et al. (2013). SO nutrient drawdown can thus cause negligible mean (deep) ocean and δ13C changes, despite causing large changes in local δ13C and Δδ13C through the interplay between biology and air–sea gas exchange. Interesting in light of glacial proxy interpretation, the fit to the δ13C : relationship is improved throughout the ocean for the Vmax experiment, similar to the effects of the “Gas slow” experiment (Fig. 8). Changes in the goodness of fit of δ13C and data to the δ13C : relationship (i.e. δ13Cbio , Sect. 2) are usually interpreted as changes in ventilation or air–sea gas exchange (Lear et al., 2016; Eide et al., 2017b). However, here we show that changes in the fit represent the relative importance of biology and air–sea gas exchange in determining δ13C, as both changes in δ13Cdiseq (i.e. gas exchange rate experiments) and changes in the biological pump can affect the goodness of fit to the δ13C : relationship (Fig. 8).
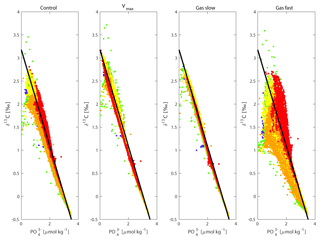
Figure 8δ13C versus for the control, Vmax, “gas slow” and “gas fast” experiments for all ocean basins except the Nordic Seas (i.e. basins A to F in Fig. S8). Dark blue = A/North Atlantic, Light blue = B/South Atlantic, Red = C/Southern Ocean, Yellow = D/South Pacific, Green = E/North Pacific, Orange = F/Indian Ocean. The black line is the δ13Cbio relationship, i.e. the relationship between δ13C and expected if only biology affected δ13C (Sect. 2). Deviations from the black line represent the relative importance of air–sea gas exchange compared to biology for δ13C.
3.3.4 Southern Ocean sea ice cover
The sea ice cover of the SO changes considerably over glacial–interglacial cycles, as well as on seasonal timescales (Crosta, 2009) and Fig. A22 therein). In general, the model sea ice cover will inhibit light penetration into the surface ocean and limit air–sea gas exchange based on its thickness (Sect. 2). In the sensitivity experiments we assume complete inhibition of both light and air–sea carbon exchange by the sea ice. In this section we thus explore the effect of both biological production and air–sea gas exchange in two extreme cases, (i) the largest realistic sea ice cover based on the glacial maximum winter extreme (50∘ S) and (ii) the smallest sea ice cover based on the contemporary summer minimum sea ice extent (70∘ S). Note that there is a constant sea ice cover about north of 70∘ N and south of 60∘ S in the control run of the model. Therefore, the strongest marine δ13C change is expected south of 60∘ S for a decreased sea ice cover and between 50 and 60∘ S for an increased sea ice cover, as this is the area where ice cover is altered relative to the control run. Ocean circulation changes that could result from a changed sea ice cover are not taken into account, as we want to study the potential isolated effect of sea ice on δ13C through biological and air–sea gas exchange changes.
Figure 9δ13C of DIC (‰) difference after 2000 years for the Antarctic sea ice cover experiments (experiment − control): the effect of a large (a, c) and small (b, d) Antarctic sea ice cover, for 25 m depth (a, b) and an Atlantic transect (c, d).
Both local and global air–sea carbon fluxes are influenced by a change in the SO sea ice cover, which results in changes in and δ13Catm. In our experiment, increases by 9 ppm for an increased sea ice cover and decreases by 6 ppm for a decreased sea ice cover (Table 2, Fig. S3). As noted in Sect. 3.3.1, a change in is governed by a transient change in the net air–sea gas exchange flux Fnet until a new equilibrium is established. An extended ice cover causes more CO2 to remain in the atmosphere because the additional ice covers a part of the SO that is a sink for CO2 (Fig. S4 – Control). As the net global air–sea gas exchange Fnet approaches equilibrium, the non-SO ocean therefore becomes a smaller source for carbon. This reduces the net gas exchange Fnet inside and outside of the SO by ∼40 %–50 %. Our results show that the effects of a changed sea ice cover on are yet to be fully understood: Stephens and Keeling (2000) for example modelled a strong decrease in in response to an increased sea ice cover south of the Antarctic Polar Front, because they mostly cover a part of the SO that is a source of carbon to the atmosphere. In our study, the reduction in by 6 ppm due to a reduced sea ice cover is attributable to the POC production in the previously ice-covered area between ∼60 and 70∘ S. In a sensitivity experiment where the ice cover influences air–sea gas exchange only, the sea ice retreat leads to an increase in because the region below the ice is strongly supersaturated in carbon with respect to the atmosphere. The increased sea ice cover leads to a complete suppression of air–sea gas exchange south of 50∘ S. Since this region is in negative carbon isotopic disequilibrium with the atmosphere (δ13C < δ13Ceq), the ice cover inhibits a δ13C flux into the ocean. As a result, increases to −6.2 ‰ , while the opposite happens for a reduced sea ice cover, leading to a lowered (−6.6 ‰).
The increased sea ice cover over the SO results in a surface ocean δ13C reduction relative to the control of −0.5 ‰ to −0.1 ‰ in the SO (Figs. 4 and 9), while δ13C increases outside of the SO by 0 ‰–0.2 ‰ (Fig. 9a). The reduction is especially pronounced between 40 and 60∘ S. The ∼40 % reduced POC export production in the SO due to light inhibition by the sea ice cover causes a major part of the SO surface δ13C reduction, as the absence of photosynthesis will cause lower surface ocean δ13C. Next to that, the reduced air–sea gas exchange Fu+d in the SO also leads to a lowered surface ocean δ13C signature. About the opposite happens when we simulate a strongly decreased sea ice cover (only ice south of 70∘ S): a small reduction of δ13C is modelled outside the SO, but the SO δ13Csurface locally becomes up to ∼0.8 ‰ higher relative to the control (Fig. 9b) as the increased amount of air–sea gas exchange Fu+d decreases the carbon isotopic disequilibrium and increases POC production in the newly exposed area, both acting to increase δ13C of DIC.
The effect of a changed ice cover on deep ocean δ13C is less than ∼0.1 ‰ (Fig. 9c, d) as the surface signal is diluted while it follows the general ocean circulation. As for air–sea gas exchange (Sect. 3.3.1), no pronounced deep ocean δ13C signal is found outside of the SO due to sea ice cover changes (this is opposed to interpretations by Lear et al., 2016). Global mean Δδ13C is not significantly affected by changes in the SO sea ice cover (Fig. 5) because the low- and high-latitude effects on δ13Csurface cancel each other out. The SO Δδ13C, however, weakens considerably to 0.4 ‰ when the 50–60∘ S region becomes covered with sea ice and strengthens to 1 ‰ if the sea ice is removed between 60 and 70∘ S (Fig. 4). The presence or absence of a sea ice cover should thus be clearly visible in especially planktic SO δ13C sediment records. The effect on Δδ13C spreads downstream of the SO, where Δδ13C is increased by up to 0.2 ‰ in the Pacific Ocean for an increased SO sea ice cover (Fig. 4).
3.4 Modelled versus observed Δδ13C variations
The variations in Δδ13C on glacial–interglacial timescales provide researchers with a tracer to study the biogeochemical state of the past global ocean, under the condition that we can interpret (variations in) Δδ13C. The idealised perturbations made to the (Southern) Ocean in this study show that global mean Δδ13C is particularly sensitive to an increased gas exchange rate and changes in the efficiency of the biological pump. Global mean Δδ13C varies by up to about ±0.35 ‰ around the pre-industrial model reference (1.2 ‰ ) in response to biogeochemical change (Fig. 5) – under the assumption of a constant ocean circulation. However, the sensitivity of Δδ13C to biogeochemical changes depends strongly on location for all sensitivity experiments (Fig. 4), possibly explaining part of the incoherency of reconstructed planktic and benthic foraminiferal δ13C from sediment cores (Oliver et al., 2010). In general, such Δδ13C reconstructions show Δδ13C variations in ∼1 ‰ over the past 350 000 years (Shackleton et al., 1983; Shackleton and Pisias, 1985; Boyle, 1988; Charles et al., 2010; Oliver et al., 2010; Ziegler et al., 2013). Ocean circulation changes explain at least part of these variations in Δδ13C (Oppo et al., 1990; Heinze et al., 1991; Heinze and Hasselmann, 1993; Toggweiler 1999; Charles et al., 2010; Jansen, 2017). However, the changes in the biogeochemical state of the ocean imposed in our experiments show that variations in Δδ13C could be strongly influenced by (SO) biogeochemistry as well. Δδ13C is increased during glacials and reduced during interglacials across a large set of sediment cores (Boyle, 1988; Charles et al., 2010; Oliver et al., 2010; Ziegler et al., 2013). Rapid and large changes have been documented for SO Δδ13C records (Ziegler et al., 2013), and here we show that biogeochemical changes in the SO affect Δδ13C globally. Our results show that an increase in mean Δδ13C could biogeochemically result from slower gas exchange, increased POC sinking rates, or an increased nutrient uptake rate in the SO (Figs. 4 and 5). Such biogeochemical changes have been associated with glacial periods (for example, Ziegler et al., 2013) and are potential candidates to explain part of the Δδ13C increase in interplay with stronger ocean stratification. Sediment-core reconstructions of Δδ13C show that an increased Δδ13C can originate from a downward shift of the metabolic imprint of low-δ13C POC, which would increase shallow δ13C (Boyle, 1988; Mulitza et al., 1998; Toggweiler, 1999; Charles et al., 2010), and/or a deep ocean δ13C decrease (Broecker, 1982; Boyle, 1988; Oliver et al., 2010), with little variation recorded for surface ocean δ13C. The absence of a clear surface δ13C signal could in the SO be the net effect of an increased sea ice cover (locally decreasing δ13C; Figs. 4 and 9a) and an increased biological pump efficiency (locally increasing δ13Csurface; Figs. 6a and b, 7a) or increased SO thermodynamic equilibrium (Fig. 3a and b) – if these opposing signals get mixed. A pronounced deep ocean δ13C decrease is associated with an efficient biological pump and older water masses in our study (Fig. 4). Interestingly, large local changes in deep ocean δ13C and Δδ13C do not necessarily imply changes in mean deep ocean (Sect. 3.3.3).
The local character of the Δδ13C sensitivity (Fig. 4) implies that correlations between sediment core Δδ13C ∕ δ13C variations and global parameters (e.g. pCO2) are not easily extrapolated to other sediment cores over large distances. Analysis of SO Δδ13C reconstructions from sediment cores, at 42 and 46∘ S (Charles et al., 2010) for example, shows that there is a strong correlation between these cores and Northern Hemisphere climate fluctuations. We expect that this strong correlation does not exist for cores further south in the SO because our results indicate that the SO south of ∼50–60∘ S often has a different Δδ13C response to biogeochemical change than the rest of the ocean.
Interglacial periods are generally thought to be associated with a decrease in the efficiency of the biological pump and increased deep-ocean ventilation via southern-sourced water masses (Gottschalk et al., 2016). Increased deep-ocean ventilation might be driven by increased winds (Tschumi et al., 2011), which would (apart from changing the SO circulation pattern) also increase gas exchange rates. Each of these processes indeed reduces mean Δδ13C in our experiments (Fig. 5), although this is less pronounced in the SO (Fig. 4). However, the interglacial reduction of Δδ13C seems to originate from a deep ocean δ13C increase compared to the glacial deep ocean δ13C (Broecker, 1982; Charles et al., 2010; Oliver et al., 2010). Our results show that neither an inefficient biological pump nor fast gas exchange can be associated with a pronounced deep sea δ13C increase relative to our control, because their effects are restricted to the surface ocean. On the other hand, the interglacial decrease in Δδ13C is a decrease compared to the glacial state: if glacial SO nutrient uptake was higher (Vmax), a return to the “normal” state (i.e. the model control run) would result in a major decrease in Δδ13C (Figs. 4 and 5).
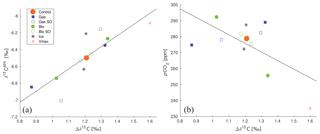
Figure 10Relationships between global mean Δδ13C, δ13Catm, and . (a) Global mean Δδ13C versus δ13Catm of the different sensitivity experiments. R2 of the best-fit line is 0.71, and the line is described by . (b) Global mean Δδ13C versus of the different sensitivity experiments. R2 of the best-fit line is 0.39, and the line is described by .
3.5 The relationship between Δδ13C, δ13Catm, and
One would expect variations in δ13Catm as well as Δδ13C to correlate with variations in , because similar processes (biology and air–sea gas exchange) steer their distribution and concentrations (Shackleton and Pisias, 1985; this article). Δδ13C is considered a promising proxy for reconstructions of for times predating ice-core records (Lisiecki, 2010). Here we show that a positive linear relationship between δ13Catm and global mean Δδ13C (Fig. 10a) holds over a wide range of biogeochemical states, as simulated in the sensitivity experiments. However, the negative linear relationship between and global mean Δδ13C (Fig. 10b) is weak (R2=0.39). Yet, previous studies do show the existence of a correlation between local Δδ13C and (such as found by for example Dickson et al., 2008), and correlation of modified Δδ13C between ocean basins with (Lisiecki, 2010). The effects of ocean circulation on glacial–interglacial δ13Catm changes, not studied here, are most pronounced in response to Antarctic Bottom Water formation rate variations and are of the order of 0 ‰–0.15 ‰ (Menviel et al., 2015). Our results show that δ13Catm varies by up to about ±0.5 ‰ in response to biogeochemical changes (Table 2). Changes in the POC sinking rate lie approximately along a line in δ13Catm : Δδ13C space (Fig. 10a), suggesting that changes in the biological pump efficiency are important for the δ13Catm : Δδ13C relationship. Likewise, both the gas exchange rate and biological pump experiments lie along an approximate lines in : Δδ13C space (Fig. 10b, albeit a different one – leading to a low total correlation). Changes in air–sea gas exchange (as simulated in the gas exchange and sea ice cover experiments) affect δ13Catm more than Δδ13C. This confirms the idea that Δδ13C is governed by biological processes and will also set δ13Catm, unless air–sea gas exchange gets the chance to dominate δ13Catm. The high potential of SO air–sea gas exchange to change δ13Catm (Table 2: sea ice and gas exchange rate experiments) complements recent studies showing that increased SO ventilation of deep ocean carbon is a likely candidate for glacial–interglacial δ13Catm excursions – which are of the order of 0.5 ‰ (Lourantou et al., 2010; Menviel et al., 2015; Bauska et al., 2016; Eggleston et al., 2016).
This study addresses the sensitivity of modelled marine and atmospheric δ13C and Δδ13C to changes in biogeochemical parameters under constant ocean circulation, focusing on the contribution of the SO (the ocean south of 45∘ S, 22 % of the global ice-free ocean area). Variations in Δδ13C recorded in sediment records are sensitive to ocean circulation changes as shown in previous studies, but here we show that the biogeochemical state of the (Southern) Ocean can also have large effects on Δδ13C across all ocean basins. Using the ocean biogeochemistry general circulation model HAMOCC2s, a set of sensitivity experiments was carried out, which focuses on the biogeochemical aspects known to be important for δ13C and Δδ13C. Specifically, the experiments explore changes in air–sea gas exchange rate, sea ice extent (influencing both biological production and the air–sea gas exchange of carbon), and the efficiency of the biological pump through the POC sinking rate and nutrient uptake rate.
The results show the important role of the SO in determining global δ13C and Δδ13C sensitivities, as well as the strong spatial differences in these. A new quasi-equilibrium state developed mostly within the first 100–800 years of the sensitivity experiments, except for the POC sinking experiment, where an imbalance between weathering and burial causes a long-term drift. The δ13C signature is governed by different processes depending on location: air–sea gas exchange sets surface ocean δ13C in all ocean basins, contributing 60 %–100 % to the δ13C signature. At depth and with increasing water mass age, the influence of biology increases to 50 % in the oldest water masses (North Pacific) due to POC remineralisation. This spatial pattern behind the δ13C signature of a water parcel results in a non-uniform sensitivity of δ13C to biogeochemical change. Global mean Δδ13C varies by up to about ±0.35 ‰ due to biogeochemical state changes in our experiments (at a constant ocean circulation) (Fig. 5). This amplitude is almost half of the reconstructed variation in Δδ13C on glacial–interglacial timescales (1 ‰), and could thus contribute to variations in Δδ13C together with ocean circulation changes. However, Δδ13C can have a different response on a basin scale: the ocean's oldest water (North Pacific) responds most to biological changes, the young deep water (North Atlantic) responds strongly to air–sea gas exchange changes, and the vertically well-mixed water (SO) has a low or even reversed Δδ13C sensitivity compared to the other basins. The amplitude of the Δδ13C sensitivity can be higher at decreasing scale, as seen from a maximum sensitivity of about −0.6 ‰ on ocean basin scale (Fig. 4). Interestingly, the direction of both glacial (intensification of Δδ13C) and interglacial (weakening of Δδ13C) Δδ13C change matches changes in biogeochemical processes thought to be associated with these periods. This supports the idea that biogeochemistry explains part of the reconstructed variations in Δδ13C, in addition to changes in ocean circulation.
An increased gas exchange rate has the potential to reduce the biologically induced Δδ13C through the reduction of surface ocean and atmospheric δ13C. Increased gas exchange, however, only reduces Δδ13C in the low latitudes: in high latitudes, increased gas exchange will increase Δδ13C (by increasing δ13Csurface) because of the negative disequilibrium δ13Cdiseq (i.e. δ13C < δ13Ceq) in this region, and thus potentially increase δ13Csurface (Sect. 3.3.1). Notably, , δ13Catm, and marine δ13C are shown to be disproportionally sensitive to SO gas exchange rate changes.
Changes in the efficiency of the biological pump also have a major potential to alter Δδ13C as well as and δ13Catm. The globally increased POC sinking rate experiment shows that Δδ13C strengthens in low latitudes (and more so in older waters) by deepening the low-δ13C signature of remineralised POC, while SO Δδ13C is not very sensitive to POC sinking rates. The SO effects are comparably small because the vertical mixing in the SO of the low-δ13C deep water only causes shifts in the entire δ13C profile, not a change in the gradient (Fig. 4). Increased POC sinking causes a long-term imbalance between weathering and sediment burial which leads to an increase in mean δ13C and δ13Catm (of about 0.15 ‰) after 2000 years. Increased nutrient uptake in the SO (Vmax experiment) results in 13 % lower non-SO POC export production up to ∼35∘ N, in agreement with previous studies on the role of the SO biological pump in lower-latitude productivity. Interestingly, the increase in Δδ13C in all ocean basins occurs without significantly changing mean (deep) ocean , which advocates for increased SO nutrient uptake to explain (part of) glacial–interglacial Δδ13C variations. Furthermore, our results show that improved goodness of fit of the model data to the δ13C : relationship can be driven by reduced gas exchange as well as biological uptake efficiency in the SO, since both increase the importance of biology relative to air–sea gas exchange for δ13C. Caution should thus be exercised when interpreting changes in the fit of observations to the δ13C : relationship as changes in ocean ventilation or air–sea gas exchange alone.
A significant linear relationship was found across the sensitivity experiments between δ13Catm and Δδ13C (R2=0.71), and a weaker one (R2=0.39) for and Δδ13C. This result shows that paleo-reconstructions of δ13Catm based on Δδ13C could be valid for a wide range of biogeochemical states. Previous studies have shown good correlation between and local Δδ13C, but our results suggest that the relationship may not be valid if both biological and gas exchange rate changes occur. The maximum response of δ13Catm to the biogeochemical changes imposed in our experiments (up to 0.5 ‰) is larger than the idealised maximum effect of ocean circulation changes on δ13Catm (0 ‰–0.15 ‰; Menviel et al., 2015), which underlines the potential importance of biogeochemical processes for variations in δ13Catm. The high potential of SO air–sea gas exchange to steer δ13Catm (Table 2: sea ice and gas exchange rate experiments) complements recent studies showing that increased SO ventilation of deep ocean carbon is a likely candidate for glacial–interglacial δ13Catm excursions.
As an outlook, the use of a more complex model with a higher horizontal and vertical resolution and a shorter time step (resolving seasonal variations) could provide valuable additional information. For example, the role of different regions within the SO on the global δ13C distribution could be better studied with a more complex model. Sediment core-based reconstructions of the global carbon cycle could possibly be aided by a more complex model with a finer grid and higher time resolution, by providing more detailed information on the contribution of biogeochemical processes to local ocean tracers. Next to that, exploring the effect on Δδ13C of a glacial model circulation field could provide a way to quantify the maximum combined effect of circulation and biogeochemical change on Δδ13C.
The model and model output can be made available upon request to the main author.
The supplement related to this article is available online at: https://doi.org/10.5194/bg-15-7205-2018-supplement.
CH provided the HAMOCC2s model. ALM and CH designed the model experiments and ALM carried them out. ALM analysed the results and prepared the paper, with feedback and supervision from JS and CH.
The authors declare that they have no conflict of interest.
This article is part of the special issue “The 10th International Carbon Dioxide Conference (ICDC10) and the 19th WMO/IAEA Meeting on Carbon Dioxide, other Greenhouse Gases and Related Measurement Techniques (GGMT-2017) (AMT/ACP/BG/CP/ESD inter-journal SI)”. It is a result of the 10th International Carbon Dioxide Conference, Interlaken, Switzerland, 21–25 August 2017.
The authors would like to thank two anonymous reviewers for their
constructive and helpful comments, which improved this paper. This
study is a contribution to the project “Earth system modelling of climate
variations in the Anthropocene” (EVA; grant no. 229771) as well as the
project “Overturning circulation and its implications for global carbon
cycle in coupled models” (ORGANIC; grant no. 239965) which are both funded
by the Research Council of Norway. This is a contribution to the Bjerknes
Centre for Climate Research (Bergen, Norway). Storage resources were
provided by the Norwegian storage infrastructure of Sigma2 (NorStore project
ns2980k). Anne L. Morée is grateful for PhD funding through the Faculty for
Mathematics and Natural Sciences of the University of Bergen and the Meltzer
Foundation. Christoph Heinze acknowledges sabbatical support from the
Faculty for Mathematics and Natural Sciences of the University of Bergen.
Edited by: Fortunat Joos
Reviewed by: two anonymous referees
Bauska, T. K., Baggenstos, D., Brook, E. J., Mix, A. C., Marcott, S. A., Petrenko, V. V., Schaefer, H., Severinghaus, J. P., and Lee, J. E.: Carbon isotopes characterize rapid changes in atmospheric carbon dioxide during the last deglaciation, P. Natl. Acad. Sci. USA, 113, 3465–3470, https://doi.org/10.1073/pnas.1513868113, 2016.
Boyle, E. A.: The role of vertical chemical fractionation in controlling late Quaternary atmospheric carbon dioxide, J. Geophys. Res.-Oceans, 93, 15701-15714, https://doi.org/10.1029/JC093iC12p15701, 1988.
Broecker, W. S.: Ocean chemistry during glacial time, Geochim. Cosmochim. Ac., 46, 1689–1705, https://doi.org/10.1016/0016-7037(82)90110-7, 1982.
Broecker, W. S. and Maier-Reimer, E.: The influence of air and sea exchange on the carbon isotope distribution in the sea, Global Biogeochem. Cy., 6, 315–320, https://doi.org/10.1029/92GB01672, 1992.
Broecker, W. S. and McGee, D.: The 13C record for atmospheric CO2: What is it trying to tell us?, Earth Planet. Sci. Lett., 368, 175–182, https://doi.org/10.1016/j.epsl.2013.02.029, 2013.
Broecker W, S. and Peng, T. H.: Gas exchange rates between air and sea, Tellus, 26, 21–35, https://doi.org/10.1111/j.2153-3490.1974.tb01948.x, 1974.
Broecker, W. S. and Peng, T.-H.: The role of CaCO3 compensation in the glacial to interglacial atmospheric CO2 change, Global Biogeochem. Cy., 1, 15–29, https://doi.org/10.1029/GB001i001p00015, 1987.
Charles, C. D., Pahnke, K., Zahn, R., Mortyn, P. G., Ninnemann, U., and Hodell, D. A.: Millennial scale evolution of the Southern Ocean chemical divide, Quaternary Science Reviews, 29, 399-409, https://doi.org/10.1016/j.quascirev.2009.09.021, 2010.
Craig, H.: Isotopic standards for carbon and oxygen and correction factors for mass-spectrometric analysis of carbon dioxide, Geochim. Cosmochim. Ac., 12, 133–149, https://doi.org/10.1016/0016-7037(57)90024-8, 1957.
Crosta, X.: Antarctic Sea Ice History, Late Quaternary, in: Encyclopedia of Paleoclimatology and Ancient Environments, edited by: Gornitz, V., Springer Netherlands, Dordrecht, the Netherlands, 31–34, 2009.
Crucifix, M.: Distribution of carbon isotopes in the glacial ocean: A model study, Paleoceanography, 20, PA4020, https://doi.org/10.1029/2005PA001131, 2005.
Curry, W. B. and Oppo, D. W.: Glacial water mass geometry and the distribution of δ13C of ΣCO2 in the western Atlantic Ocean, Paleoceanography, 20, PA1017, https://doi.org/10.1029/2004PA001021, 2005.
DeVries, T., Primeau, F., and Deutsch, C.: The sequestration efficiency of the biological pump, Geophys. Res. Lett., 39, L13601, https://doi.org/10.1029/2012GL051963, 2012.
Dickson, A. J., Leng, M. J., and Maslin, M. A.: Mid-depth South Atlantic Ocean circulation and chemical stratification during MIS-10 to 12: implications for atmospheric CO2, Clim. Past, 4, 333–344, https://doi.org/10.5194/cp-4-333-2008, 2008.
Dunne, J. P., Sarmiento, J. L., and Gnanadesikan, A.: A synthesis of global particle export from the surface ocean and cycling through the ocean interior and on the seafloor, Global Biogeochem. Cy., 21, GB4006, https://doi.org/10.1029/2006GB002907, 2007.
Duplessy, J. C., Shackleton, N. J., Fairbanks, R. G., Labeyrie, L., Oppo, D., and Kallel, N.: Deepwater source variations during the last climatic cycle and their impact on the global deepwater circulation, Paleoceanography, 3, 343–360, https://doi.org/10.1029/PA003i003p00343, 1988.
Eggleston, S., Schmitt, J., Bereiter, B., Schneider, R., and Fischer, H.: Evolution of the stable carbon isotope composition of atmospheric CO2 over the last glacial cycle, Paleoceanography, 31, 434–452, https://doi.org/10.1002/2015PA002874, 2016.
Eide, M., Olsen, A., Ninnemann, U. S., and Eldevik, T.: A global estimate of the full oceanic 13C Suess effect since the preindustrial, Global Biogeochem. Cy., 31, 492–514, https://doi.org/10.1002/2016GB005472, 2017a.
Eide, M., Olsen, A., Ninnemann, U. S., and Johannessen, T.: A global ocean climatology of preindustrial and modern ocean δ13C, Global Biogeochem. Cy., 31, 515–534, https://doi.org/10.1002/2016GB005473, 2017b.
Emerson, S. and Hedges, J.: Chemical oceanography and the marine carbon cycle, Cambridge University Press, Cambridge, UK, xi, 453 pp. of col. plates pp., 2008.
Galbraith, E. D., Kwon, E. Y., Bianchi, D., Hain, M. P., and Sarmiento, J. L.: The impact of atmospheric pCO2 on carbon isotope ratios of the atmosphere and ocean, Global Biogeochem. Cy., 29, 307–324, https://doi.org/10.1002/2014GB004929, 2015.
Gottschalk, J., Skinner, L. C., Lippold, J., Vogel, H., Frank, N., Jaccard, S. L., and Waelbroeck, C.: Biological and physical controls in the Southern Ocean on past millennial-scale atmospheric CO2 changes, Nat. Commun., 7, 11539, https://doi.org/10.1038/ncomms11539, 2016.
Gruber, N. and Keeling, C. D.: An improved estimate of the isotopic air-sea disequilibrium of CO2: Implications for the oceanic uptake of anthropogenic CO2, Geophys. Res. Lett., 28, 555–558, https://doi.org/10.1029/2000GL011853, 2001.
Gruber, N., Keeling, C. D., Bacastow, R. B., Guenther, P. R., Lueker, T. J., Wahlen, M., Meijer, H. A. J., Mook, W. G., and Stocker, T. F.: Spatiotemporal patterns of carbon-13 in the global surface oceans and the oceanic suess effect, Global Biogeochem. Cy., 13, 307–335, https://doi.org/10.1029/1999GB900019, 1999.
Heinze, C.: Assessing the importance of the Southern Ocean for natural atmospheric pCO2 variations with a global biogeochemical general circulation model, DeepSea Res. Pt. II, 49, 3105–3125, https://doi.org/10.1016/S0967-0645(02)00074-7, 2002.
Heinze, C., and Hasselmann, K.: Inverse Multiparameter Modeling of Paleoclimate Carbon Cycle Indices, Quaternary Res., 40, 281–296, https://doi.org/10.1006/qres.1993.1082, 1993.
Heinze, C. and Maier-Reimer, E.: The Hamburg Oceanic Carbon Cycle Circulation Model Version “HAMOCC2s” for long time integrations, Max-Planck-Institut für Meteorologie, Hamburg REPORT 20, 1999.
Heinze, C., Maier-Reimer, E., and Winn, K.: Glacial pCO2 Reduction by the World Ocean: Experiments With the Hamburg Carbon Cycle Model, Paleoceanography, 6, 395–430, https://doi.org/10.1029/91PA00489, 1991.
Heinze, C., Hoogakker, B. A. A., and Winguth, A.: Ocean carbon cycling during the past 130 000 years – a pilot study on inverse palaeoclimate record modelling, Clim. Past, 12, 1949–1978, https://doi.org/10.5194/cp-12-1949-2016, 2016.
Hilting, A. K., Kump, L. R., and Bralower, T. J.: Variations in the oceanic vertical carbon isotope gradient and their implications for the Paleocene-Eocene biological pump, Paleoceanography, 23, PA3222, https://doi.org/10.1029/2007PA001458, 2008.
Holden, P. B., Edwards, N. R., Müller, S. A., Oliver, K. I. C., Death, R. M., and Ridgwell, A.: Controls on the spatial distribution of oceanic δ13CDIC, Biogeosciences, 10, 1815–1833, https://doi.org/10.5194/bg-10-1815-2013, 2013.
Hollander, D. J. and McKenzie, J. A.: CO2 control on carbon-isotope fractionation during aqueous photosynthesis: A paleo-pCO2 barometer, Geology, 19, 929–932, https://doi.org/10.1130/0091-7613(1991)019<0929:ccocif>2.3.co;2, 1991.
Hoogakker, B. A. A., Elderfield, H., Schmiedl, G., McCave, I. N., and Rickaby, R. E. M.: Glacial-interglacial changes in bottom-water oxygen content on the Portuguese margin, Nat. Geosci., 8, 40–43, https://doi.org/10.1038/ngeo2317, 2015.
Jahn, A., Lindsay, K., Giraud, X., Gruber, N., Otto-Bliesner, B. L., Liu, Z., and Brady, E. C.: Carbon isotopes in the ocean model of the Community Earth System Model (CESM1), Geosci. Model Dev., 8, 2419–2434, https://doi.org/10.5194/gmd-8-2419-2015, 2015.
Jansen, M. F.: Glacial ocean circulation and stratification explained by reduced atmospheric temperature, P. Natl. Acad. Sci. USA, 114, 45–50, https://doi.org/10.1073/pnas.1610438113, 2017.
Jones, D. C., Ito, T., Takano, Y., and Hsu, W.-C.: Spatial and seasonal variability of the air-sea equilibration timescale of carbon dioxide, Global Biogeochem. Cy., 28, 1163–1178, https://doi.org/10.1002/2014GB004813, 2014.
Keir, R. S.: The effect of vertical nutrient redistribution on surface ocean δ13C, Global Biogeochem. Cy., 5, 351–358, https://doi.org/10.1029/91GB01913, 1991.
Kroopnick, P.: The distribution of 13C in the Atlantic Ocean, Earth Planet. Sc. Lett., 49, 469–484, https://doi.org/10.1016/0012-821X(80)90088-6, 1980.
Kroopnick, P. M.: The distribution of 13C of ΣCO2 in the world oceans, Deep-Sea Res., 32, 57–84, https://doi.org/10.1016/0198-0149(85)90017-2, 1985.
Laws, E. A., Bidigare, R., R., and Popp, B. N.: Effects of growth rate and CO2 concentration on carbon isotopic fractionation by the marine diatom Phaeodactylum tricornutum, Limnol. Oceanogr., 42, 1552–1560, 1997.
Lear, C. H., Billups, K., Rickaby, R. E. M., Diester-Haass, L., Mawbey, E. M., and Sosdian, S. M.: Breathing more deeply: Deep ocean carbon storage during the mid-Pleistocene climate transition, Geology, 44, 1035–1038, https://doi.org/10.1130/G38636.1, 2016.
Lisiecki, L. E.: A benthic δ13C-based proxy for atmospheric pCO2 over the last 1.5 Myr, Geophys. Res. Lett., 37, L21708, https://doi.org/10.1029/2010GL045109, 2010.
Lourantou, A., Lavrič Jošt, V., Köhler, P., Barnola, J. M., Paillard, D., Michel, E., Raynaud, D., and Chappellaz, J.: Constraint of the CO2 rise by new atmospheric carbon isotopic measurements during the last deglaciation, Global Biogeochem. Cy., 24, GB2015, https://doi.org/10.1029/2009GB003545, 2010.
Lutz, M. J., Caldeira, K., Dunbar, R. B., and Behrenfeld, M. J.: Seasonal rhythms of net primary production and particulate organic carbon flux to depth describe the efficiency of biological pump in the global ocean, J. Geophys. Res.-Oceans, 112, C10011, https://doi.org/10.1029/2006JC003706, 2007.
Lynch-Stieglitz, J., Stocker, T. F., Broecker, W. S., and Fairbanks, R. G.: The influence of air-sea exchange on the isotopic composition of oceanic carbon: Observations and modeling, Global Biogeochem. Cy., 9, 653–665, https://doi.org/10.1029/95GB02574, 1995.
MacCready, P. and Quay, P.: Biological export flux in the Southern Ocean estimated from a climatological nitrate budget, Deep-Sea Res. Pt. II, 48, 4299–4322, https://doi.org/10.1016/S0967-0645(01)00090-X, 2001.
Mackenzie, F. T. and Lerman, A.: Isotopic Fractionation of Carbon: Inorganic and Biological Processes, in: Carbon in the Geobiosphere – Earth's Outer Shell –, edited by: Mackenzie, F. T. and Lerman, A., Springer Netherlands, Dordrecht, the Netherlands, 165–191, 2006.
Marchal, O., Stocker, T. F., and Joos, F.: A latitude-depth, circulation-biogeochemical ocean model for paleoclimate studies. Development and sensitivities, Tellus B, 50, 290–316, https://doi.org/10.1034/j.1600-0889.1998.t01-2-00006.x, 1998.
Marinov, I., Gnanadesikan, A., Toggweiler, J. R., and Sarmiento, J. L.: The Southern Ocean biogeochemical divide, Nature, 441, 964–967, 2006.
Menviel, L., Mouchet, A., Meissner, K. J., Joos, F., and England, M. H.: Impact of oceanic circulation changes on atmospheric δ13CO2, Global Biogeochem. Cy., 29, 1944–1961, https://doi.org/10.1002/2015GB005207, 2015.
Menviel, L., Yu, J., Joos, F., Mouchet, A., Meissner, K. J., and England, M. H.: Poorly ventilated deep ocean at the Last Glacial Maximum inferred from carbon isotopes: A data-model comparison study, Paleoceanography, 32, 2–17, https://doi.org/10.1002/2016PA003024, 2016.
Milliman, J. D. and Droxler, A. W.: Neritic and pelagic carbonate sedimentation in the marine environment: ignorance is not bliss, Geol. Rundsch., 85, 496–504, https://doi.org/10.1007/BF02369004, 1996.
Mook, W. G.: 13C in atmospheric CO2, Neth. J. Sea Res., 20, 211–223, https://doi.org/10.1016/0077-7579(86)90043-8, 1986.
Mulitza, S., Rühlemann, C., Bickert, T., Hale, W., Pätzold, J., and Wefer, G.: Late Quaternary δ13C gradients and carbonate accumulation in the western equatorial Atlantic, Earth Planet Sci. Lett., 155, 237–249, https://doi.org/10.1016/S0012-821X(98)00012-0, 1998.
Murnane, R. J. and Sarmiento, J. L.: Roles of biology and gas exchange in determining the δ13C distribution in the ocean and the preindustrial gradient in atmospheric δ13C, Global Biogeochem. Cy., 14, 389–405, https://doi.org/10.1029/1998GB001071, 2000.
Nevison, C. D., Keeling, R. F., Kahru, M., Manizza, M., Mitchell, B. G., and Cassar, N.: Estimating net community production in the Southern Ocean based on atmospheric potential oxygen and satellite ocean color data, Global Biogeochem. Cy., 26, GB1020, https://doi.org/10.1029/2011GB004040, 2012.
Oliver, K. I. C., Hoogakker, B. A. A., Crowhurst, S., Henderson, G. M., Rickaby, R. E. M., Edwards, N. R., and Elderfield, H.: A synthesis of marine sediment core δ13C data over the last 150 000 years, Clim. Past, 6, 645–673, https://doi.org/10.5194/cp-6-645-2010, 2010.
Oppo, D. W., Fairbanks, R. G., and Gordon, A. L.: Late Pleistocene Southern Ocean δ13C variability, Paleoceanography, 5, 43–54, https://doi.org/10.1029/PA005i001p00043, 1990.
Primeau, F. W., Holzer, M., and DeVries, T.: Southern Ocean nutrient trapping and the efficiency of the biological pump, J. Geophys. Res.-Oceans, 118, 2547–2564, https://doi.org/10.1002/jgrc.20181, 2013.
Quay, P., Sonnerup, R., Westby, T., Stutsman, J., and McNichol, A.: Changes in the 13C ∕ 12C of dissolved inorganic carbon in the ocean as a tracer of anthropogenic CO2 uptake, Global Biogeochem. Cy., 17, 4-1–4-20, https://doi.org/10.1029/2001GB001817, 2003.
Roth, R., Ritz, S. P., and Joos, F.: Burial-nutrient feedbacks amplify the sensitivity of atmospheric carbon dioxide to changes in organic matter remineralisation, Earth Syst. Dynam., 5, 321–343, https://doi.org/10.5194/esd-5-321-2014, 2014.
Sarmiento, J. L., Gruber, N., Brzezinski, M. A., and Dunne, J. P.: High-latitude controls of thermocline nutrients and low latitude biological productivity, Nature, 427, 56–60, 2004.
Schlitzer, R.: Carbon Export Fluxes in the Southern Ocean: Results from Inverse Modeling and Comparison with Satellite Estimates, Deep-Sea Res., 2, 1623–1644, 2002.
Schmittner, A. and Somes, C. J.: Complementary constraints from carbon (13C) and nitrogen (15N) isotopes on the glacial ocean's soft-tissue biological pump, Paleoceanography, 31, 669–693, https://doi.org/10.1002/2015PA002905, 2016.
Schmittner, A., Gruber, N., Mix, A. C., Key, R. M., Tagliabue, A., and Westberry, T. K.: Biology and air–sea gas exchange controls on the distribution of carbon isotope ratios (δ13C) in the ocean, Biogeosciences, 10, 5793–5816, https://doi.org/10.5194/bg-10-5793-2013, 2013.
Shackleton, N. J., Hall, M. A., Line, J., and Shuxi, C.: Carbon isotope data in core V19-30 confirm reduced carbon dioxide concentration in the ice age atmosphere, Nature, 306, 319–322, https://doi.org/10.1038/306319a0, 1983.
Shackleton, N. J. and Pisias, N. G.: Atmospheric carbon dioxide, orbital forcing, and climate, in: The Carbon cycle and atmospheric CO2: natural variations archean to present, edited by: Sundquist, E. T., and Broecker, W. S., Geophysical Monograph, American Geophysical Union, Washington, USA, 303–317, 1985.
Sonnerup, R. E. and Quay, P. D.: 13C constraints on ocean carbon cycle models, Global Biogeochem. Cy., 26, GB2014, https://doi.org/10.1029/2010GB003980, 2012.
Stephens, B. B. and Keeling, R. F.: The influence of Antarctic sea ice on glacial–interglacial CO2 variations, Nature, 404, 171–174, https://doi.org/10.1038/35004556, 2000.
Tagliabue, A. and Bopp, L.: Towards understanding global variability in ocean carbon-13, Global Biogeochem. Cy., 22, GB1025, https://doi.org/10.1029/2007GB003037, 2008.
Toggweiler, J. R.: Variation of atmospheric CO2 by ventilation of the ocean's deepest water, Paleoceanography, 14, 571–588, https://doi.org/10.1029/1999PA900033, 1999.
Tréguer, P.: Silica and the cycle of carbon in the ocean, C. R. Geosci., 334, 3–11, https://doi.org/10.1016/S1631-0713(02)01680-2, 2002.
Tschumi, T., Joos, F., Gehlen, M., and Heinze, C.: Deep ocean ventilation, carbon isotopes, marine sedimentation and the deglacial CO2 rise, Clim. Past, 7, 771–800, https://doi.org/10.5194/cp-7-771-2011, 2011.
Zahn, R., Winn, K., and Sarnthein, M.: Benthic foraminiferal δ13C and accumulation rates of organic carbon: Uvigerina Peregrina group and Cibicidoides Wuellerstorfi, Paleoceanography, 1, 27–42, https://doi.org/10.1029/PA001i001p00027, 1986.
Zeebe, R. and Wolf-Gladrow, D.: CO2 in Seawater: Equilibrium, Kinetics, Isotopes, Elsevier Oceanography Series, edited by: Halpern, D., Elsevier Science B.V., Amsterdam, the Netherlands, 346 pp., 2001.
Zhang, J., Quay, P. D., and Wilbur, D. O.: Carbon isotope fractionation during gas-water exchange and dissolution of CO2, Geochim. Cosmochim. Ac., 59, 107–114, https://doi.org/10.1016/0016-7037(95)91550-D, 1995.
Ziegler, M., Diz, P., Hall, I. R., and Zahn, R.: Millennial-scale changes in atmospheric CO2 levels linked to the Southern Ocean carbon isotope gradient and dust flux, Nat. Geosci., 6, 457–461, https://doi.org/10.1038/ngeo1782, 2013.